k CD/Nr
12
k2
=
=
CENr
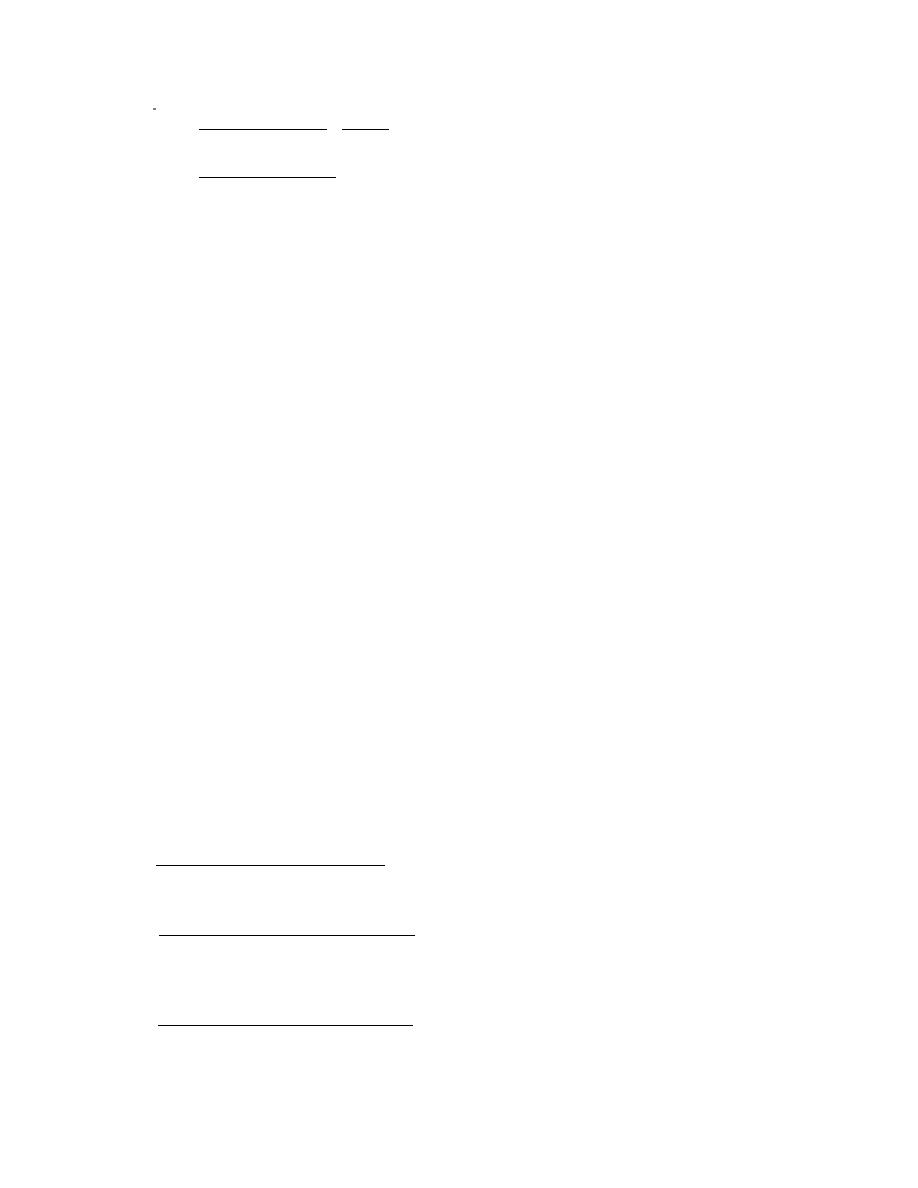
Drag coefficient
[ln(r / z0 )][ln(r / zQ )] ln(r/zQ )
Overland (1985) reviewed 45 evaluations of the drag
CDNr
(107)
coefficient over sea ice published through 1984. Since
=
.
1 C1/2 ln(z / z )
k
1
then, Anderson (1987), Fairall and Markson (1987),
0
Q
DNr
Guest and Davidson (1987, 1991), Martinson and
Thus, knowing z0, zT and zQ is equivalent to knowing
Wamser (1990), Wamser and Martinson (1993),
the neutral-stability bulk transfer coefficients for any
Andreas et al. (1993b), and Andreas and Claffey (1995)
reference height r.
have added to this information pool.
We also see from eq 106 and 107 that equality of z0,
But I still feel that one of the most important works
zT and zQ implies that CDNr = CHNr = CENr. Although
on this subject is that by Banke et al. (1980). They
some measurements have suggested that CHNr = CENr,
showed that CDN10 is related to a measurable surface
none have confirmed that CDNr is, in general, equal to
roughness parameter ξ through (Fig. 8)
either of these, as I will explain shortly. Thus, equality
between z0 and zT or zQ is not true either.
103 CDN10 = 1.10 + 0.072ξ
(111)
From eq 102104, it is clear that if we know the
neutral-stability transfer coefficients or z0 and zT and
where ξ must be in centimeters. Here, ξ obtains from an
zQ, we know the stability-dependent transfer coefficients
integration under the snow-surface roughness spectrum,
needed in eq 9193 to compute the turbulent fluxes of
Φs
interest. Deardorff (1968) was one of the first to show
plots of the bulk transfer coefficients as functions of sta-
∞
∫ Φs (κ)dκ
ξ =
bility. More recently, Smith (1988) presented updated
2
(112)
plots using modern expressions for the necessary simi-
κ0
larity functions. In a nutshell, for constant wind speed,
where κ is the wavenumber and κ0 = 0.5 rad/m, which
CD, CH and CE all increase as conditions become more
unstable; and, again for a constant wind speed, all de-
corresponds to a maximum wavelength of about 13 m.
In turn, the snow-surface roughness spectrum, Φs(κ),
crease as stability increases. These results follow intui-
tively from our discussion of how vertical exchange
is the spectral density (Andreas et al. 1993b) obtained
is enhanced in unstable conditions and suppressed in
from a Fourier analysis of a snow-surface elevation pro-
stable conditions.
file. Figure 9 shows such a snow-surface elevation pro-
Since we can obtain the bulk transfer coefficients at
file and the actual ice-surface elevation profile measured
any height and for any stratification from the roughness
on Ice Station Weddell (ISW) in 1992 (Anonymous 1992,
lengths or from the neutral-stability coefficients, we can
ISW Group 1993). Figure 10 shows the resulting (non-
dimensional) snow-surface (Φs) and ice-surface (Φi)
confine the rest of our discussion to the values of these
neutral-stability coefficients. I will also settle on the stan-
roughness spectra implied by these profiles (see Andreas
dard reference height of 10 m and, henceforth, confine
et al. [1993b] for computational guidelines). According
to eq 112, ξ is obtained from the Φs line in Figure 10
my discussion to the neutral-stability transfer coefficients
by integrating from κ = 0.5 rad/m to infinity. That upper
the following equations show how to compute CDr, CHr
integration limit, however, is not actually infinity but rather
the Nyquist wavenumber 2π/2∆, where ∆ is the sampling
and CEr from these neutral-stability values (Andreas and
Murphy 1986)
interval. Since Banke et al. (1980) used a sampling inter-
val of 1 m, for their ξ values the upper integration limit
CDN10
was π rad/m. Because the sampling interval for the ISW
CDr =
(108)
{1 +
}
2
k 1 CD/N10 [ln(r /10) -
ψm(r / L)]
12
profiles was 0.5 m, however, the Nyquist wavenumber for
these is 2π rad/m (Fig. 10).
Figure 8 and eq 111 suggest that form drag is impor-
CDN10 (CDr / CDN10 )1 / 2
=
CHr
tant in fostering momentum exchange. As the sur-
1 + k 1 CHN10 CD1/20 [ln(r / 10) - ψ h (r / L)]
face gets measurably rougher, there are more vertical
N1
surfaces for the wind to push against; CDN10 conse-
(109)
quently increases. Measurements in the 1980s in the
marginal ice zone--arguably the roughest oceanic sur-
CEN10 (CDr / CDN10 )1 / 2
=
CEr
face--corroborated this hypothesis that as the surface
1 + k 1 CEN10 CD1/20 [ln(r / 10) - ψ h (r / L)]
gets visibly rougher the drag coefficient increases
N1
(110)
(Andreas et al. 1984; Brown 1986, 1990; Anderson 1987;
where r must be expressed in meters.
Guest and Davidson 1987, 1991).
16



 Previous Page
Previous Page
