5
h = 15 cm
4
3
2
γ = 0.20
0.15
0.10
1
0.05
0
0
20
40
60
80
100
120
140
160
180
Wind Direction, Φ ( )
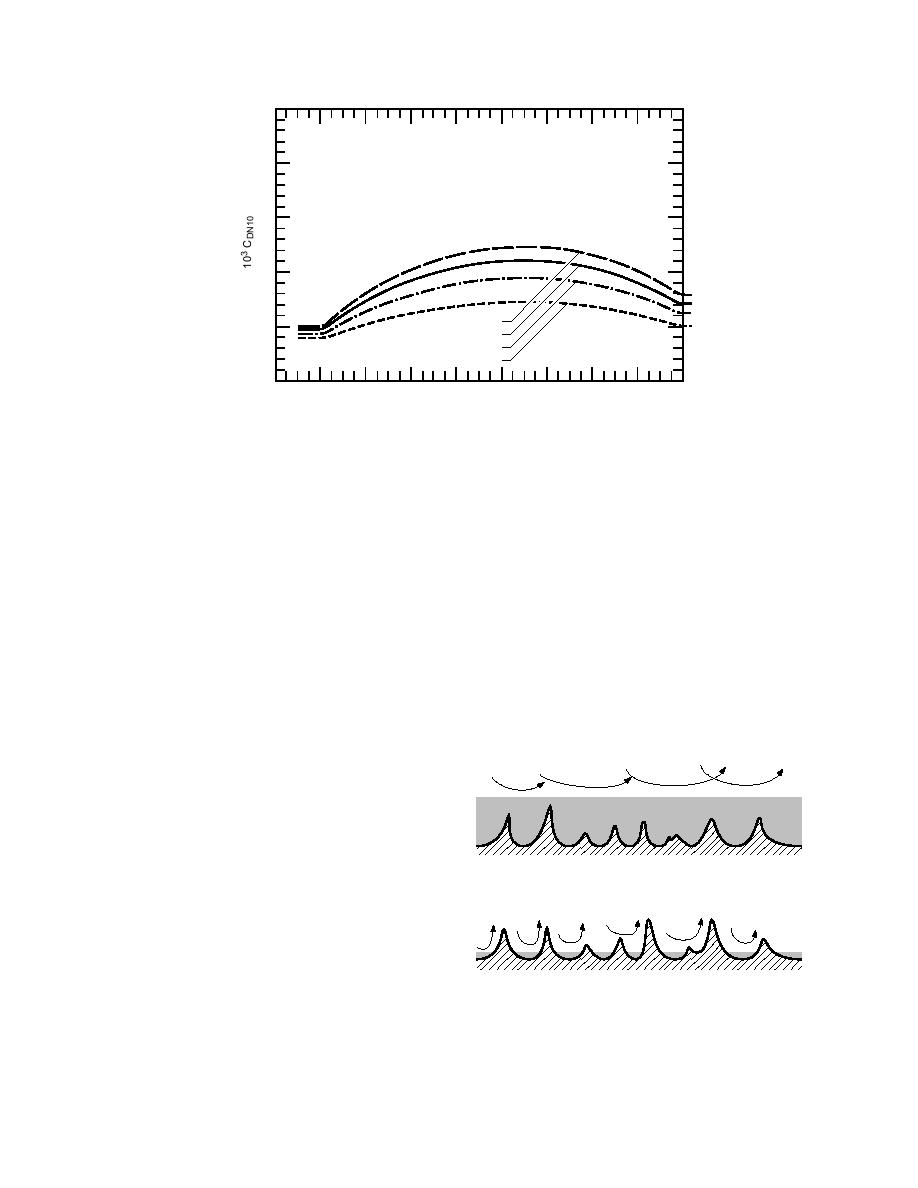
Figure 17. Model calculations of the neutral-stability, 10-m drag coefficient,
CDN10, as a function of the wind orientation, Φ, with respect to the dominant
axis of the sastrugi depicted in Figure 16 (from Andreas 1995a,b). Here, the
sastrugi height, h, is 15 cm; and γ is the fraction of the surface that the sastrugi
cover. Also, m = 4 and n = 10 (see Fig. 16).
ISW the minimum CDN10 value was about 1.5 103.
surfaces, momentum and scalars are transferred by dif-
ferent processes.
When the wind is roughly at a right angle to the sastrugi,
the model predicts a maximum in CDN10 of 2.70 103,
Figure 18 is a depiction of the microscale of a natu-
while our observed maximum was 2.54 103.
ral surface such as snow. The surface has millimeter-
size roughness elements that sometimes are embedded
Our ISW observations and this modeling have, thus,
in the viscous sublayer and sometimes protrude above
shown some deficiencies in eq 111. Because drifting
the viscous sublayer. The roughness Reynolds number,
snow makes a sea ice surface anisotropic, eq 111 can be
true only if ξ is obtained from a snow-surface profile
eq 53, parameterizes the aerodynamic character of a
surface. When R ≤ 0.135, the surface is aerodynami-
aligned with the mean wind. But our observations and
*
cally smooth; when R ≥ 2.5, the surface is aerodynami-
modeling also reiterate one of the conclusions implicit
*
cally rough; when 0.135 < R < 2.5, the surface is in
in eq 111: Small roughness elements (heights of order
*
transition (Businger 1973).
1015 cm) with fairly short wavelengths (order
of meters) dominate the local form drag over snow-
covered surfaces. Jackson and Carroll (1978) had reached
the same conclusion. Because the horizontal scales in-
volved are much smaller than either Arctic or Ant-
arctic ridge spacings (Lytle and Ackley 1991), pressure
ridges seem to be less important in local processes.
Nevertheless, pressure ridges may still be important in
Aerodynamically Smooth, R ≤ 0.135
*
setting the large-scale roughness and drag coefficient
over sea ice (Arya 1973, 1975).
Scalar bulk transfer coefficients
The Reynolds analogy (Schlichting 1968, p. 268 f.
and 662 f.; Hinze 1975, p. 746) is the hypothesis that
Aerodynamically Rough, 2.5 ≤ R
momentum and scalars, such as temperature or humid-
*
ity, are transferred similarly. An immediate conclusion
Figure 18. Schematic depiction of the microscale rough-
of the Reynolds analogy is that CDN10 = CHN10 = CEN10.
ness of an aerodynamically smooth and an aerodynami-
In discussing eq 105107, however, I noted that, in geo-
cally rough surface. The shading shows the viscous sub-
physical flows at least, CDN10 has never been proven to
layer, where momentum transfer to the surface must be by
molecular processes.
equal CHN10 or CEN10. The reason is that, over natural
21



 Previous Page
Previous Page
