Figure 15. CDN10 values in Figure 12, averaged over 1-m/s bins of the 10-m wind speed

Figure 13. Two long events on Ice Station Weddell that were characterized by a relatively constant wind direction

Scalar bulk transfer coefficients

M96_02
Page Navigation
20

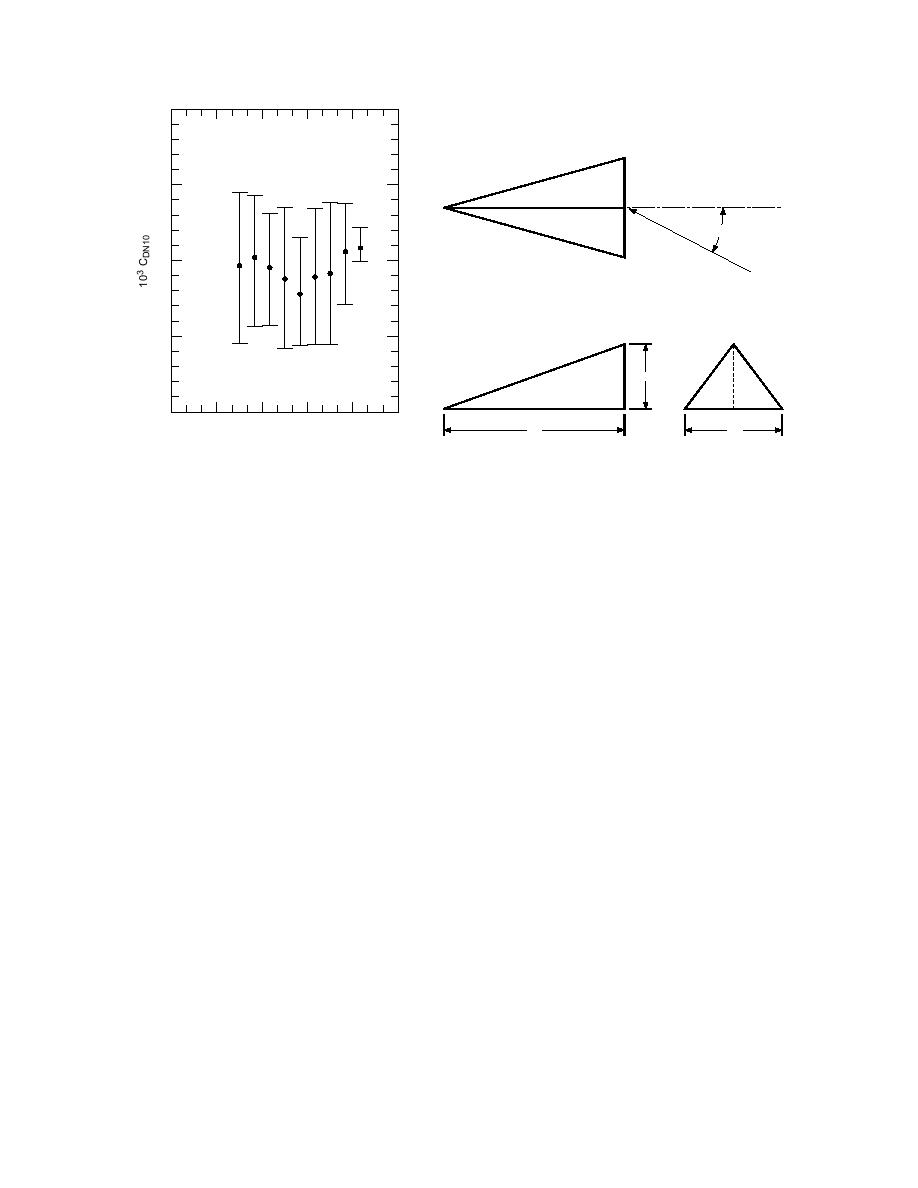
Figure 15. CDN10 values in Figure 12, averaged

Figure 16. Geometry of the sastrugi-like roughness elements

over 1-m/s bins of the 10-m wind speed; the filled

envisioned to dictate the drag coefficient over snow-covered

circle denotes this average. Next to each circle is

sea ice. If the wind has been blowing above 68 m/s for

the number of CDN10 values in each bin. The verti-

1012 hours, the sastrugi will be aligned with the mean

wind direction; Φ will then be 0.

cal bar indicates the 90% confidence interval for

the population within each bin, assuming a beta

distribution with lower limit 1.2 103 and upper

limit 2.60 103 (Harr 1977, p. 495 f.).

We thus inferred that, for most of the hourly values

rudimentary sastrugi-like roughness elements that I treat

depicted in Figure 13, wind-driven snow was present

with the model. Although we made no formal observa-

(Andreas and Claffey 1995). As a result, the decrease in

tions of sastrugi or surface texture on ISW, we often

the CDN10 values during the two events suggests that

saw that high wind sculpted the snow surface into linear

snowdrifts perpendicular to the wind were eroding, and

drifts that looked like Figure 16. Figure 9 supports these

this snow was depositing in drifts that tended to stream-

casual observations; it shows that the snow collected

line the surface in the mean wind direction. This stream-

around protrusions in the sea ice surface but that the re-

lining lowered the aerodynamic roughness and therefore

sulting drifts were generally small. Hence, I assume that

the drag coefficient.

the typical sastrugi height, h in Figure 16, was roughly

Visual inspection of Figure 13 may suggest that the

1015 cm on ISW.

behavior of CDN10 is simply a wind speed effect: Lower

Figure 17 shows model predictions of how CDN10

CDN10 values seem to be associated with lower wind

depends on the wind's orientation with respect to the

long axis of the sastrugi (Φ in Fig. 16). In the figure, γ is

speeds. But looking at the entire ISW set of drag coeffi-

cients (Fig. 15) does not corroborate this speculation.

the fractional surface coverage of the roughness elements

CDN10 does not have any obvious dependence on the

depicted in Figure 16. Notice, because of the geometry

wind speed for speeds between 4 and 14 m/s. Likewise,

of the modeled sastrugi, the maximum possible value of

γ is 0.50.

Shirasawa (1981) found no wind speed dependence in

drag coefficients measured over snow-covered lake ice

Figure 17 reproduces some of the observations that I

for winds up to 11 m/s, and Inoue (1989) also found no

have been describing. When the wind is well aligned

(within 15) with the sastrugi, CDN10 has a minimum.

wind speed dependence at Mizuho Station on the Ant-

arctic continent for winds up to about 15 m/s.

As the wind turns to blow more perpendicularly to the

To investigate further this hypothesis that streamlining

sastrugi (without being high enough to erode them),

CDN10 increases. For h = 15 cm and γ = 0.15, the model

of the surface by wind-driven snow has a major effect

on the airice drag coefficient, I (Andreas 1995a, 1995b)

predicts CDN10 values in line with what we observed on

ISW. The model yields CDN10 = 1.43 103 when the

developed a physically based form drag model follow-

wind is within 12 of head-on to the sastrugi, while on

ing Raupach's (1992) guidelines. Figure 16 depicts the

Index

Privacy Statement
Press Release
Contact