Ve
tb 2
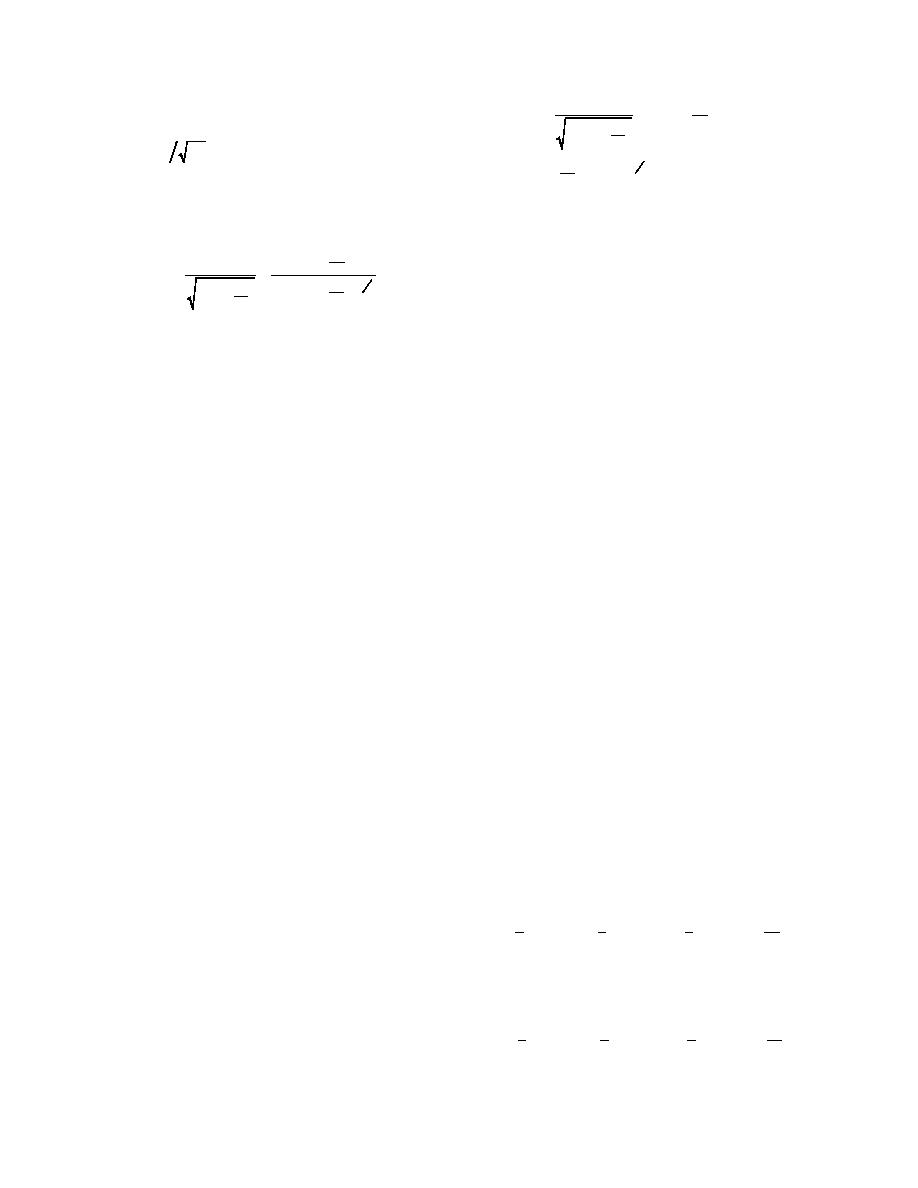
Fe,c =
= [-2.26(
) +
0.7 m/s (2.25 ft/s) and the channel Froude number
ρi
L
gtb (1 -
(V
)
ρ)
gh
tb
+ 0.015]- 2
1
(40)
2.14
L
does not exceed 0.08. Ashton (1974) analyzed the
surface stability of floating ice blocks and obtained the
where Vc = flow velocity below the cover and L = length
following submergence condition:
of ice floe. The experimental study of Kawai et al.
tb
2(1 -
(1997) showed that the critical Froude number depends
)
Vc
H
Fc =
=
not only on tb /L. For different floe sizes, their
(39)
tb 2 12
ρ
gt(1 - ρi ) [5 - 3(1 - H ) ]
experimental results showed that the Fe,c = f(tb/L)
relationships are different. This shows the complexity
where
of the problem, and the difficulty in describing the
Vc = water velocity upstream of the ice block
mechanism by a simple formula. For typical Missouri
tb = ice block thickness
River ice floes of thickness tb = 0.15 m, and size L = 1.5
H = flow depth upstream of the accumulation.
~ 6 m, eq 40 gives Ve,c = 0.76 ~ 1.4 m/s. In a 1:25
physical model test with natural ice scaled to the
This formula compared well with experimental data.
observed ice size distribution of the Missouri River ice,
Larsen (1975) studied the stability of thin blocks and
Tuthill and Gooch (1998) measured an erosion velocity
showed that Fc is much larger than 1.4 when
to be about 1.5 m/s (5 ft/s).
(tb/H) < 0.1. Daly and Axelson (1990) further refined
Boom submergence condition
these studies. More recent field studies showed that,
under certain conditions, specially designed booms
The present model assumes that each span of the
can perform successfully at water velocities as
ice boom is designed to submerge and to allow ice to
high as 0.76 m/s (2.5 ft/s) and Froude numbers
overtop it when a critical value of cable tension is
above 0.12 (White 1992). Results of a recent 1:25
exceeded (Shen et al. 1997). When the load is reduced
scale physical model study by Tuthill and Gooch
and the weight of the ice above the boom is smaller
(1998) support existing ice entrainment criteria, find-
than the net buoyancy of the boom, the boom will rise
ing that 0.3-m-thick-ice blocks submerge in a
again to prevent ice from passing through. The value
4.5-m-deep channel at a velocity of about 0.76 m/s (2.5
of critical cable tension, which varies with the geometry
ft/s) (all units prototype), and a channel Froude number
and size of booms, has to be specified for the model
of 0.1.
simulation.
Ice load on the boom
Erosion of ice on the underside of ice jams
When an ice jam forms, its thickness may be limited
When the boom stops the ice movement, mechanical
by the stability of ice particles on its underside. As the
thickening will occur and the internal stress will build
jam thickens near its downstream end, the water flow
up quickly in the ice rubble. The load on the boom and
area decreases, increasing water velocity and shear
the span cable tension can be calculated from the ice
on the ice underside. The shear may become large
rubble stresses.
enough to erode ice pieces from the jam's underside
Consider a triangular differential element of ice
rubble abc (Fig. 5) in contact with the boom, which has
and transport them downstream. Thinning the
a finite thickness ti. The total force acting in the x-
downstream end of the jam by erosion lowers the water
level at the upstream end, increasing the velocity and
direction is
the tendency for ice entrainment. This process of under-
∑ Fx = -σ xx Ntidy - σ yx Ntidx + XNtidl +
ice erosion near the toe and entrainment at the upstream
1 τ Ndxdy + 1 τ Ndxdy + 1 ρ gNt dxdy η
end of the jam may be sufficient to halt upstream
.
2 sx
2 wx
2 i
i
x
progression.
The stability of ice floes on the underside of an ice
(41)
The total force acting in the y-direction is
cover or ice jam has been investigated by Ashton (1974),
Uzuner (1977), Tatinclaux and Gogus (1981), and Daly
∑ Fy = -σ yy Ntidx - σ xy Ntidy + YNtidl +
and Axelson (1990). Tatinclaux and Gogus (1981)
η
recommended an empirical stability criterion in terms
+ 1 τsy Ndxdy + 1 τ wy Ndxdy + 1 ρi gNtidxdy
2
2
2
y
of the critical Froude number Fe,c and the floe aspect
ratio tb /L
(42)
9



 Previous Page
Previous Page
