We can write the surface-layer solutions eq 97, 99
turbulence is essential to the definition of the ABL. Con-
sequently, h should denote the surface that separates the
and 100 as
turbulent ABL from the (generally) nonturbulent upper
[
]
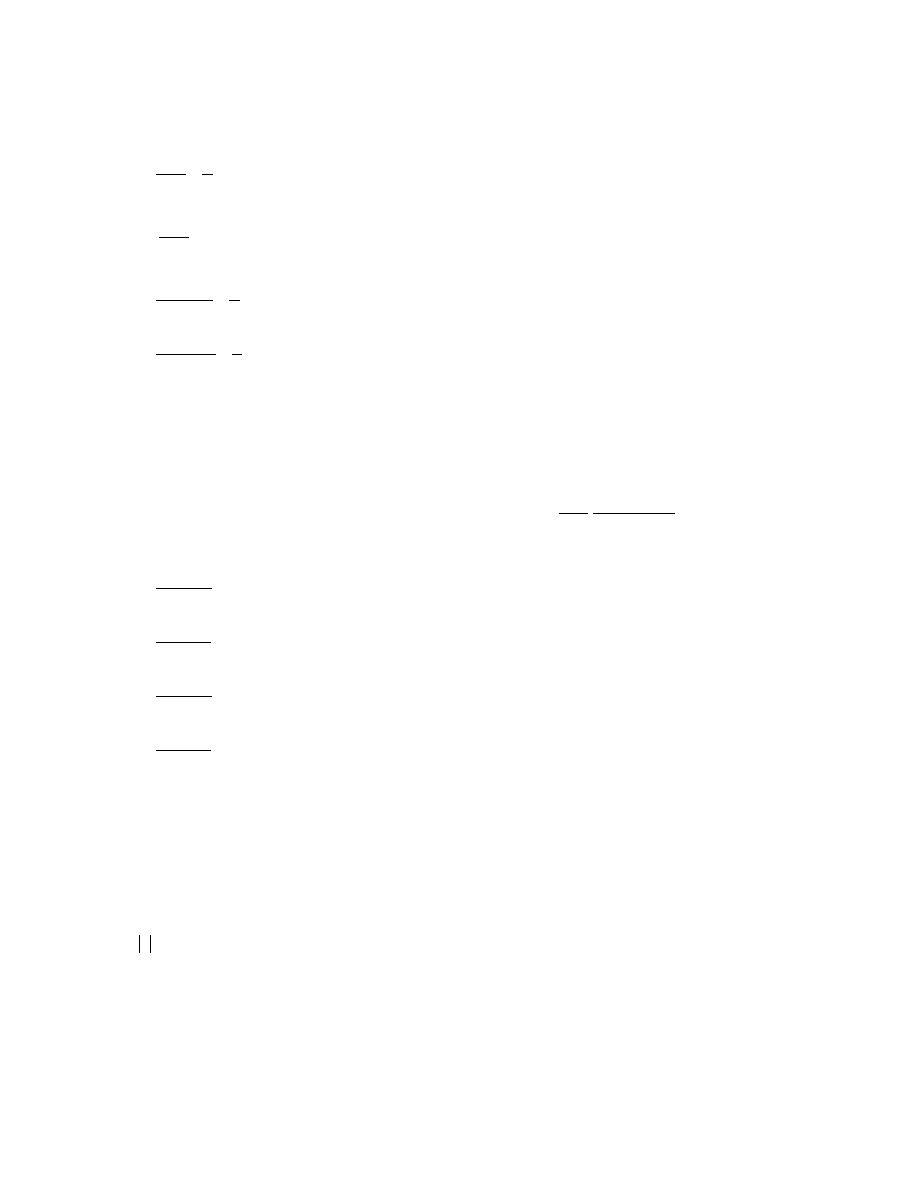
= ln(z / z0 ) - ψ m (ζ)
U(z) 1
air. Rarely, however, do we have profiles of turbulence
(139a)
u*
k
intensities through the ABL, let alone into the
upper air. Thus, it is common to use a surrogate, such as
the height of the temperature inversion zi, as an estimate
V (z)
=0
(139b)
for h (e.g., Kaimal et al. 1976). While zi is a good esti-
u*
mate for h in the convective boundary layer, in the sta-
ble boundary layer often found over polar marine sur-
Θ(z) - Ts 1
[
]
= ln(z / zT ) - ψ h (ζ)
faces, zi can overestimate h (e.g., Mahrt et al. 1979).
(140)
t*
k
Hanna (1969) reviewed a host of empirical methods
for estimating h from surrogate information in both
[(
]
Q(z) - Qs 1
)
= ln z / zQ - ψ h (ζ) .
stable and unstable conditions. Mahrt et al. (1979) con-
(141)
q*
k
centrated on estimating h in the stable ABL. Their main
conclusion was that the height of the core of the low-
level jet (zj) that is common in stable boundary layers is
Equation 139b is true because Monin-Obukhov similar-
ity aligns the x axis with the mean wind (the U compo-
a good estimator of h. In turn, the height of the turbulent
layer predicted by a Richardson number criterion, zRi, is
nent) and assumes no turning of the wind in the surface
layer.
Likewise, on seeing eq 116 and considering the scales
number is computed from
involved, we can show that the profiles in the Ekman
Θ(z) - Ts
gz
Ri(z) =
layer should obey defect laws (Blackadar and Tennekes
(145)
Θ(z) U(z)2 + V (z)2
1968; Yamada 1976; Tennekes 1982; Brutsaert 1982, p.
72 ff.) such that
and zRi is defined as the height at which
U(z) - U
^
= FU (z / h, h / L)
(142a)
Ri(zRi) ≥ 0.4
u*
(146)
V (z) - V
^
= sgnf FV (z / h, h / L)
where 0.4 is the critical Richardson number (see also
(142b)
u*
Heinemann and Rose 1990).
On Ice Station Weddell, our radiosondes often found
Θ(z) - Θ
^
= FΘ (z / h, h / L)
low-level jets (Andreas et al. 1993a, Claffey et al. 1994,
(143)
t*
Andreas et al. 1995). If we assume, as Mahrt et al. (1979)
did, that zj is an estimate for h, Figure 25 shows that the
Q(z) - Q
^
= FQ (z / h, h / L).
inversion height is usually a poor estimate of h in the
(144)
q*
stable ABL. In defining zi in this figure, we used Kahl's
(1990) definition. As such, zi could be called the top of
In these, U, V, Θ and Q are, respectively, longitudinal
^ ^ ^
^
the inversion layer. In unstable conditions, on the other
hand, zi is often taken as the base of the inversion (e.g.,
velocity, transverse velocity, potential temperature and
specific humidity scales that I will explain shortly.
Kaimal et al. 1976). The conflicting definitions arise
If the scaling is accurate, the functions FU, FV, FΘ
because in a stable ABL the inversion base is often at the
and FQ should depend only on the dimensionless ratios
surface (Kahl 1990, Serreze et al. 1992, Claffey et al.
z/h and h/L, where h is now the height of the ABL and L
1994).
Figure 26, in contrast, suggests that zRi, which
is again the Obukhov length. In particular, these func-
tions should not depend on the surface Rossby number
approximates the height at which turbulence ceases, is a
G / f z0 (Blackadar and Tennekes 1968, Tennekes 1973,
fair estimate of h--if we assume that h corresponds to
zj, the height of the jet core. Thus, we see that over sea
Hess 1992). Thus, these and subsequent arguments
are generally referred to as Rossby number similarity
ice in late fall and winter, the ABL is generally quite
shallow; in Figure 26, zj is usually between 50 and 300 m.
(Blackadar and Tennekes 1968, Hess 1973, Tennekes
1973).
Overland and Guest (1991) and Guest and Davidson (1994)
Although to continue the similarity arguments we do
offered additional insight into how the radiation budget,
not need a formal definition of h, this is a good time to
especially during the winter, dictates the thermal and tur-
digress on its meaning. As I explained, the presence of
bulent structure of the ABL and, therefore, its height.
28



 Previous Page
Previous Page
