2.3
plotted with the jam thickness profile for
Baseline
the baseline test. The 100-m upstream
2.2
spacing resulted in a final thickness pro-
2.1
file that was almost identical to the
baseline run. The profile shape and
2.0
Random Spacing
maximum thickness were very similar.
1.9
The 200-m upstream spacing showed
1.8
slightly more variation than the 100-m
upstream spacing but was also very
1.7
5000
4000
3000
2000
1000
0
similar in shape and maximum thickness
x Location
to the baseline run.
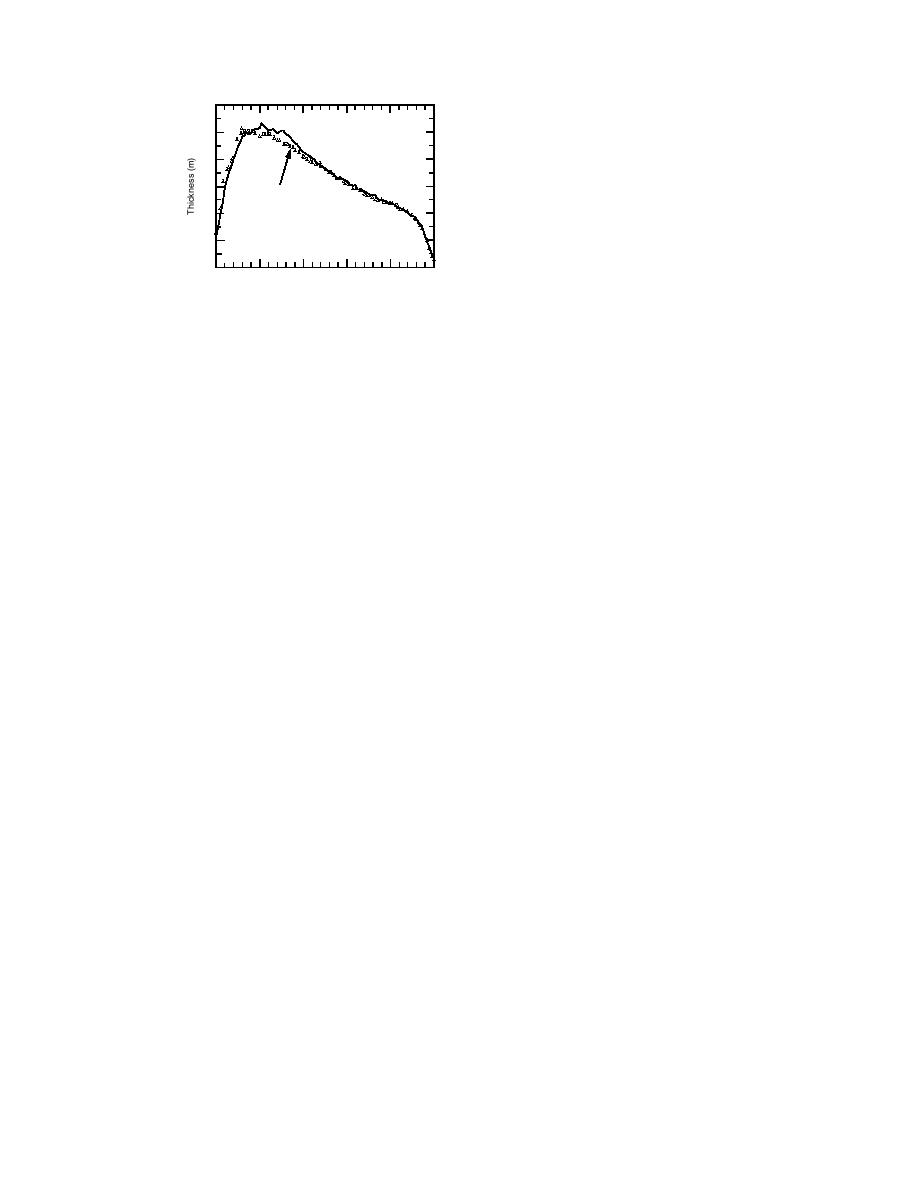
Figure 51. Final jam thickness profile for
The final run simulated random spac-
random length steps throughout the system.
ing of cross sections (typical of field data)
by using randomly assigned length steps
between 25 and 100 m (intervals of 5 m) for a total flow length of 5000 m with 81
cross sections. Now, Cr ranged from 1.5 to 6. Figure 51 shows the final jam thick-
ness profile for this run along with the baseline results. Minor variations existed in
the final thickness profile (up to 0.03 m), primarily in the most upstream 2000 m of
the system. Overall profile shape and maximum thickness, however, were very
similar to the baseline run. This check on model robustness shows that variable
length steps have minor negligible effects on predicted results, as long as the Cou-
rant number limitations identified earlier are followed.
Summary
The main points emerging here are that the fully coupled model of ice jam
dynamics is robust and versatile. Alternate boundary conditions and the use of
variable length steps make the model adaptable to a variety of physical situations
and computational capabilities. The sensitivities of results to computational pa-
rameters are found to be minimal as long as the parameters are held within reason-
able (practical, physically based) ranges.
Even at this stage of use, the model shows that the effects of ice momentum on
the solution are quite significant, as evidenced in the profiles of jam thickness. For
example, the baseline jam thickness profile shows that almost the entire system
ends up with jam thicknesses greater than those calculated using the stationary
jam theory embodied in eq 25. Furthermore, the model shows that shoving and
thickening make up a highly unsteady process, marked by variations and interac-
tions between the dependent variables not represented in past modeling efforts.
Small changes in any of the dependent variables can result in variations in the
timing of the shoving and thickening event, with subsequent variations in the final
jam thickness profile.
UNSTEADY JAM DYNAMICS
Effects of ice momentum
A primary objective of this study is to ascertain the effects of ice momentum on
jam formation and thickness. The importance of ice momentum already became
evident when the stability and overall robustness of the numerical model devel-
oped for the study were evaluated. The fully coupled, numerical model includes
ice momentum in the conservation of momentum expression formulated for ice in
67



 Previous Page
Previous Page
