momentum is important and should be taken into account for determining jam
thickness profile. For these cases, the results of simpler steady-state models should
be used with caution.
The force balance on a jam at static equilibrium can be represented by equations
such as eq 19 or 150 with the nonuniform terms set to zero. The downstream-acting
forces of the water shear stress on the jam underside and the component of gravity
due to the weight of the jam are balanced by the shear resistance at the banks
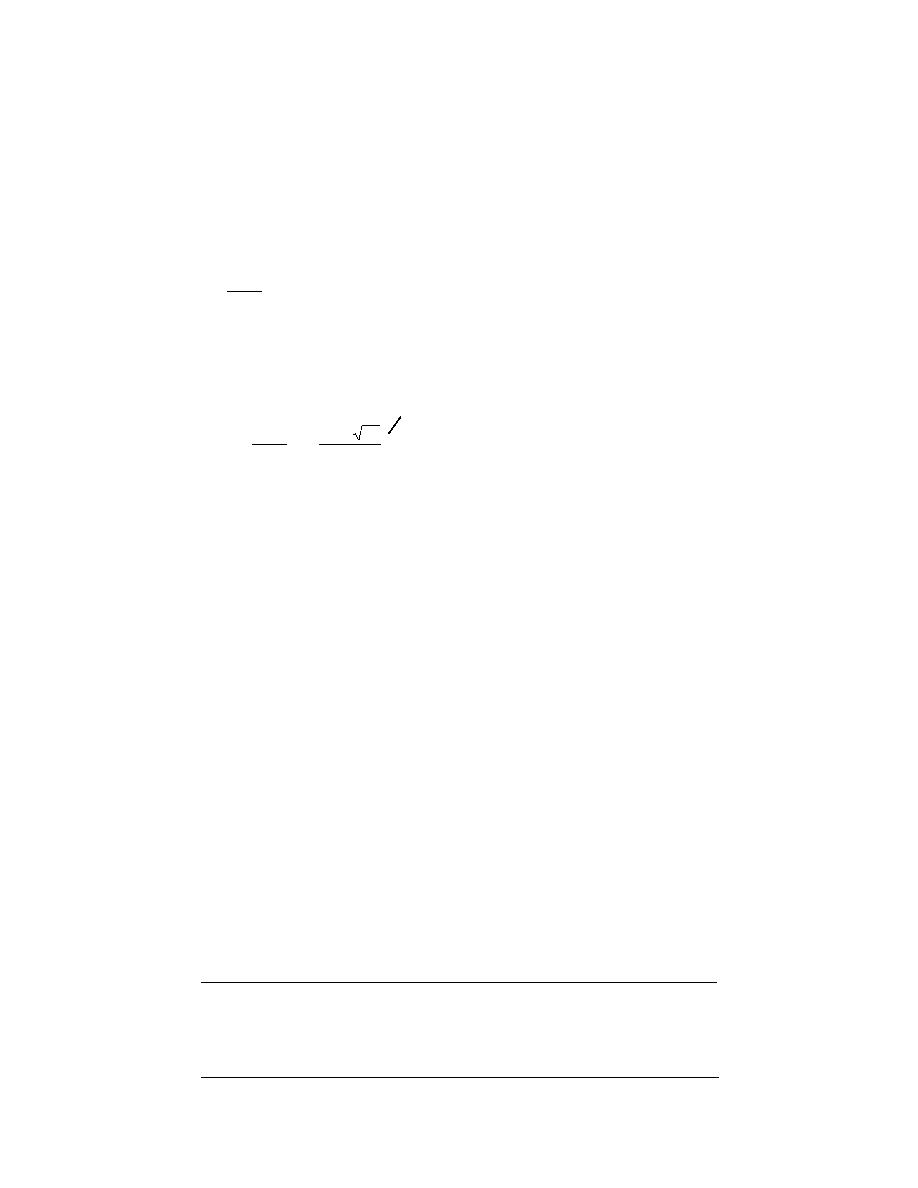
fiu2B
+ gSoBsi η = gsi (1 - p)(1 - si )k0λKpη2 .
(153)
8
If the simplified notation of a + b = c is used to describe eq 153, then a/c represents
the portion of the jam strength (represented by the bank shear resistance) mobi-
lized by water shear stress. By assuming a wide, rectangular channel, the first term
in eq 153 can also be written as
2
QS g 2B 3
f u2B
a= i
= fi o
.
(154)
8
8 fo
Together with the equilibrium thickness given by eq 25, Table 2 was generated for
jams at the verge of stability (equilibrium thickness) for flow in channels of differ-
ent bed slopes.
Table 2 shows that, for very low bed slopes, downstream gravity forces are mini-
mal with the water shear stress mobilizing most of the jam's strength. For the smallest
bed slope, when the discharge was increased by a factor of 2, a/c remained very
high and the equilibrium thickness increased by 24%. At the highest bed slope
shown, water shear stress only engages a small part of the jam's strength. A similar
factor of 2 increase in discharge increases a/c, but it still remains low and only a 6%
change results in the equilibrium thickness. Smaller bed slopes are associated with
lower water velocities, but also much lower jam thicknesses. As bed slope increases,
water shear stress increases, but at a slower rate than the gravity force term (b in eq
153). Once a jam fails, it is water shear stress that transports the ice downstream.
While this finding is not entirely intuitive, it means that ice momentum effects be-
come more important as the a/c ratio increases, i.e., for smaller bed slopes and
smoother channels.
Consider further the a and c terms and their ratio in eq 153 for a bed slope of
0.0005 given in Table 2. Equation 154 shows that, for a given discharge, the a term
has a set value. Equation 153 balances the forces by adjusting to the value of equi-
librium thickness, giving a value for a/c at the limit of stability. But, for example, if
the thickness had attained a value greater than the equilibrium level (say 1.60 m),
Table 2. Ice parameters for channels at different bed slopes.
ηeq at 100 m3/s
ηeq at 200 m3/s
a/c at 100 m3/s
a/c at 200 m3/s
Bed slope, So
0.000005
0.21 m
0.960
0.26 m
0.968
0.00005
0.49 m
0.829
0.60 m
0.861
0.0005
1.47 m
0.432
1.69 m
0.509
0.001
2.30 m
0.277
2.57 m
0.352
0.0025
4.74 m
0.121
5.02 m
0.171
72



 Previous Page
Previous Page
