range between 1 and 16C. These data were
sis of ice strength was carried out using test data
extrapolated to calculate the friction angle φ and
of Rist and Murrell (1994). This polycrystalline ice
the ice strength for temperature 40C (Fig. 7) and
had a grain size of 1.7 mm. The comparison was
performed for temperature 20C and the strain
compared (see Fig. 10 below) with test data of
rate range between ~103 s1 and ~ 105 s1. The
Rist and Murrell (1994) obtained for this tempera-
ture and for the grain size of 1.7 mm.
results of the analysis confirmed the above con-
One can see that despite the considerable dif-
clusion. At the same time our studies indicate
ference (almost five times) in the grain sizes of
that the ice cohesion magnitude is strongly de-
these two types of ice and the difference in the
pendent on the ice structure.
test temperatures, the predicted angle of internal
friction correlates well with the test data. We find
the friction angle magnitudes are unaffected by
ANGLE OF INTERNAL FRICTION
variations of the grain size of ice.
Thus, eq 27 can be used for calculations of
From the above studies an important conclu-
parameter b(T) in the above equations and for
sion can also be made regarding the physical na-
prediction of the ice strength over a wide spec-
ture of the angle of internal friction of ice. While
trum of temperatures between 0 and 40C. It
the temperature dependency of the friction pa-
rameter b(θ) = tan φ(θ) is nonlinear (Fig. 4), the
should be remembered that the ice friction angle
is a function of the strain rate as well. It decreases
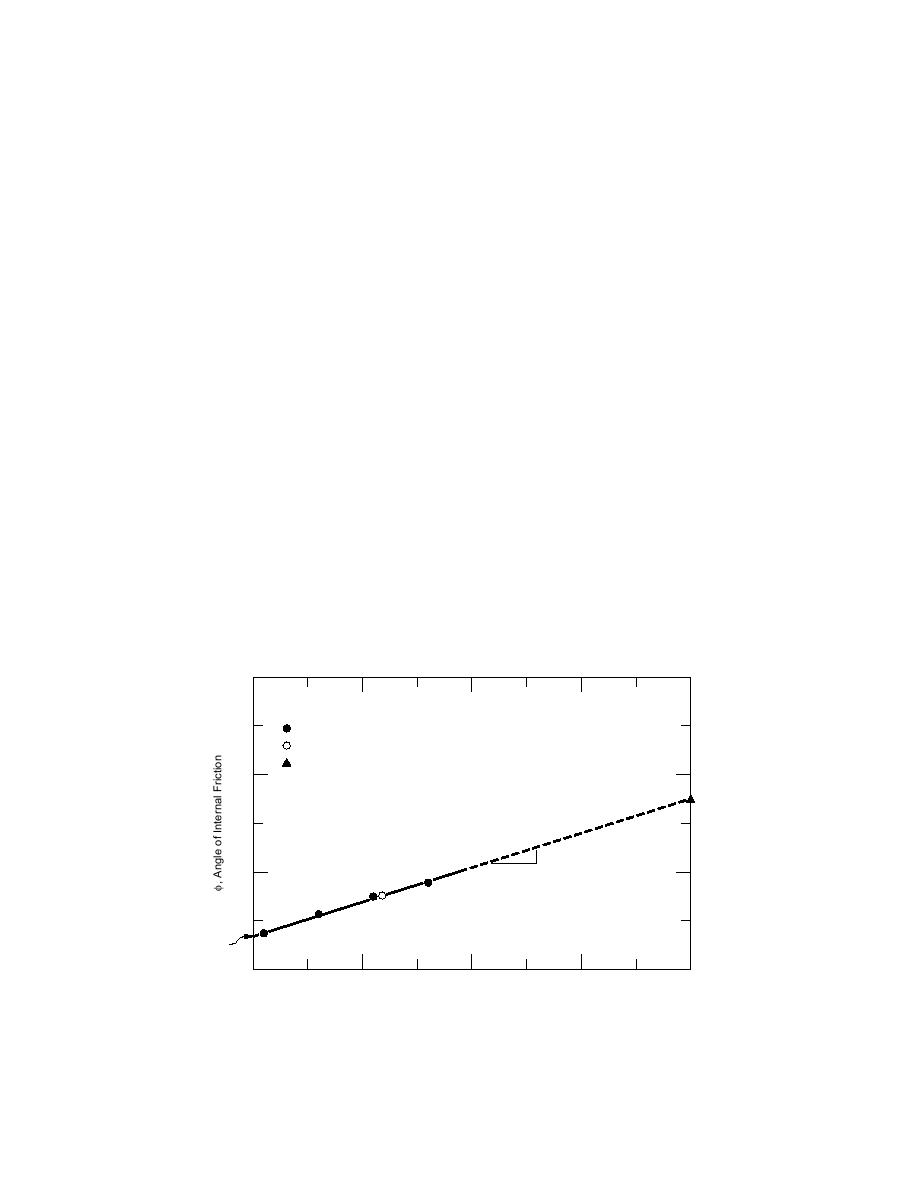
test data from Table 1 plotted in Figure 7 suggest
rapidly with the strain rate decrease. Fish (1991,
that the friction angle is a simplest linear function
1993) showed that at low strain rates ~10-7 s1 and
of temperature:
below, ice at 10C can be considered as an ide-
φ(θ) = φo + ω|θ|
ally cohesive (φ = 0) material, the strength of which
(27)
is defined by eq 10 and 26. At high temperatures
where φo = 250′, ω = 16.4′ degree1 andθ= (Tm
the internal friction angle is small and so is its
T ) is ice temperature (C). It should be remem-
effect on the ice strength. However, at low tem-
bered that the magnitudes of the parameters in eq
peratures or at high strain rates and high confin-
27 refer to a strain rate ε ~ 10-3 s-1.
˙
ing pressures, the effect of the internal friction
Note that the magnitudes of the internal fric-
angle on the strength of ice can be considerable.
tion angles φ in Table 1 and Figure 7 were calcu-
Since parameters c, b and p* were found to be
lated based mainly upon test data on the Labra-
unaffected by variations of the grain size. Table 1
data can be used to predict strength of various
dor iceberg ice (d = 8.1 mm) at the temperature
24
.
ε ≈ 10 3 s 1
Data from
Gagnon and Gammon (1995)
Jones (1982)
Rist and Murrell (1994) (predicted)
16
ted
pola
a
Extr
ω
8
φο
0
10
20
30
40
θ, Temperature (C)
Figure 7. Angle of internal friction of ice as a function of temperature. Data from
Gagnon and Gammon (1995), Jones (1982), and Rist and Murrell (1994).
10



 Previous Page
Previous Page
