U∆T1
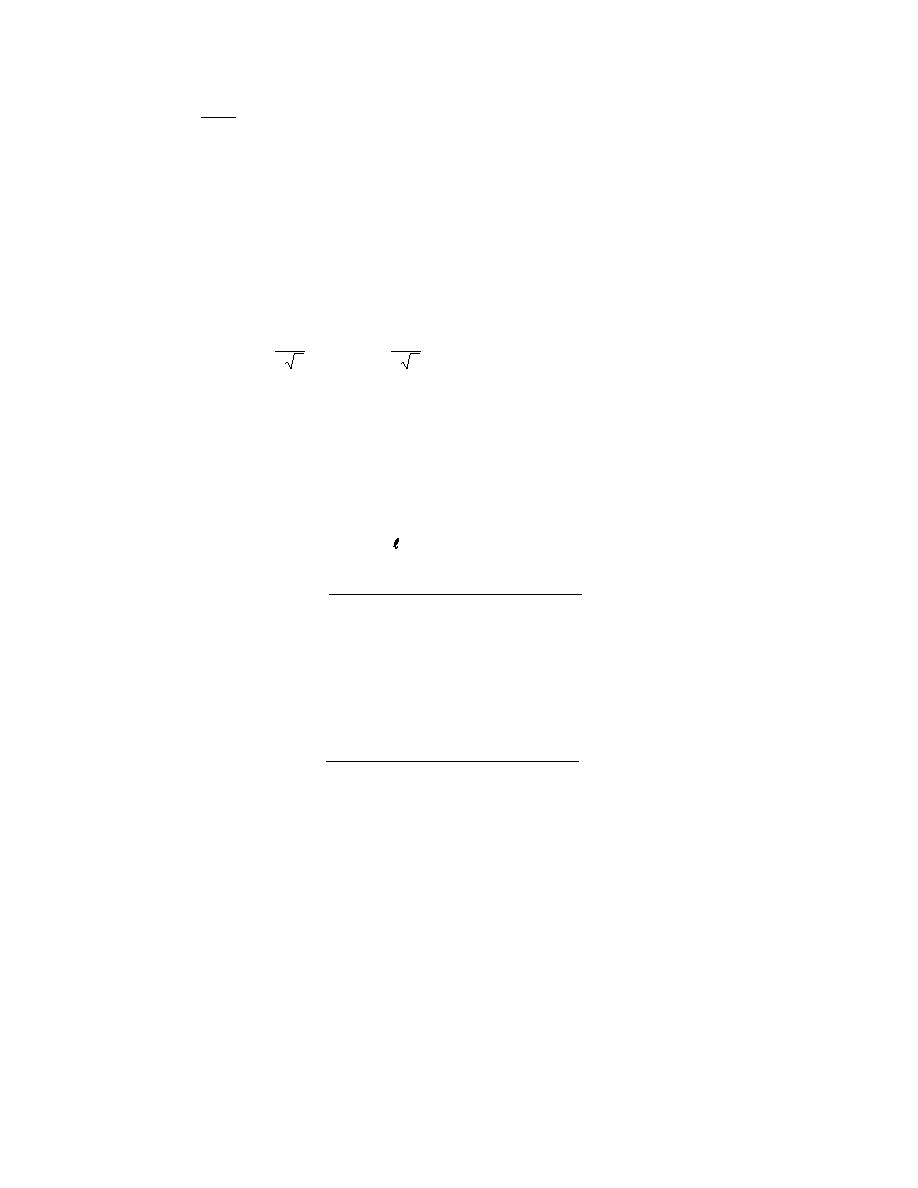
ψ=
.
Gα1
PFTSYNB.FOR in Appendix E). The model requires only the ratios of the thawed to frozen values of ther-
mal conductivity, specific heat capacity, and density for the permafrost soils, as was noted earlier.
Syngenetic model verification
It is possible to check the solution for a special case as was done for the heterogenetic growth. Although
there is no exact solution for the phase-change case, there is an exact solution for the transient location of
the Tf isotherm for the same problem with a homogeneous soil with zero latent heat, i.e, infinite Stefan
number (Lunardini, in prep.). The relation is
B
A
eψσ f ( B - 1) erfc
- ( A + 1) erfc
+ 2A = 0
(29)
.
2 τ
2 τ
A = σf ψτ
where
B = σf + ψτ
σf = location of Tf isotherm.
If we let the Stefan number be large and hold the property ratios to unity, the heat balance integral solution
for syngenetic growth can be compared to this exact relation. Table 2 notes the results for typical cases.
Table 2. Movement of Tf isotherm, homo-
geneous soil, syngenetic freezing.
ST =1000, = 0, Tf Ts = 10C, φ = 0, G =
0.0286C/m.
τ
σf exact σf approximate % difference
a. U = 10 mm/yr
0.0014
0.0087
0.090
1.5
0.0069
0.1634
0.1700
4.0
2.033
0.7739
0.7700
0.5
2.8232
0.8206
0.8100
1.3
b. U = 1 mm/yr
0.005
0.1448
0.15
3.6
0.099
0.3874
0.4006
3.42
1.0689
0.6572
0.6662
1.37
11.4705
0.8795
0.8720
0.85
The results indicate that the approximate technique gives excellent results, especially as the time in-
creases. Thus, the Heat Balance Integral method and the numerical quadrature are robust even for very long
time spans. See also eq C17 for further verification of the solution method with phase change.
DISCUSSION
Equation 9 was solved numerically using Simpson's rule. This resulted in values of the permafrost depth
versus time as a function of ST, ε and the thermal property ratios of the frozen and the thawed zones. The
results are presented in Figures 1517.
These graphs depend only upon the quantities ST, φ and ε. In Appendix A it is shown that the soil por-
osity, ε, determines the saturated soil property ratios. The thermal property ratios used for the graphs are
listed in Tables A1 and A2. The graphs are only valid for the particular soil ratios given. However, this
does not affect the validity of the model itself. Any specific site can be modeled by using site-specific prop-
erty ratios in eq 9. Figures 1517 can be used to estimate permafrost formation times for a wide range of
surface temperatures and geothermal gradients.
The graphs can also be applied to variable surface temperatures with a bit of manipulation. Figure 18
14



 Previous Page
Previous Page
