Figure 19. Yield surface for the crushable foam model.
(After HKS 1998.)
q
pl
dε
σ
g
p
Figure 20. Plastic flow surface for the crushable foam model.
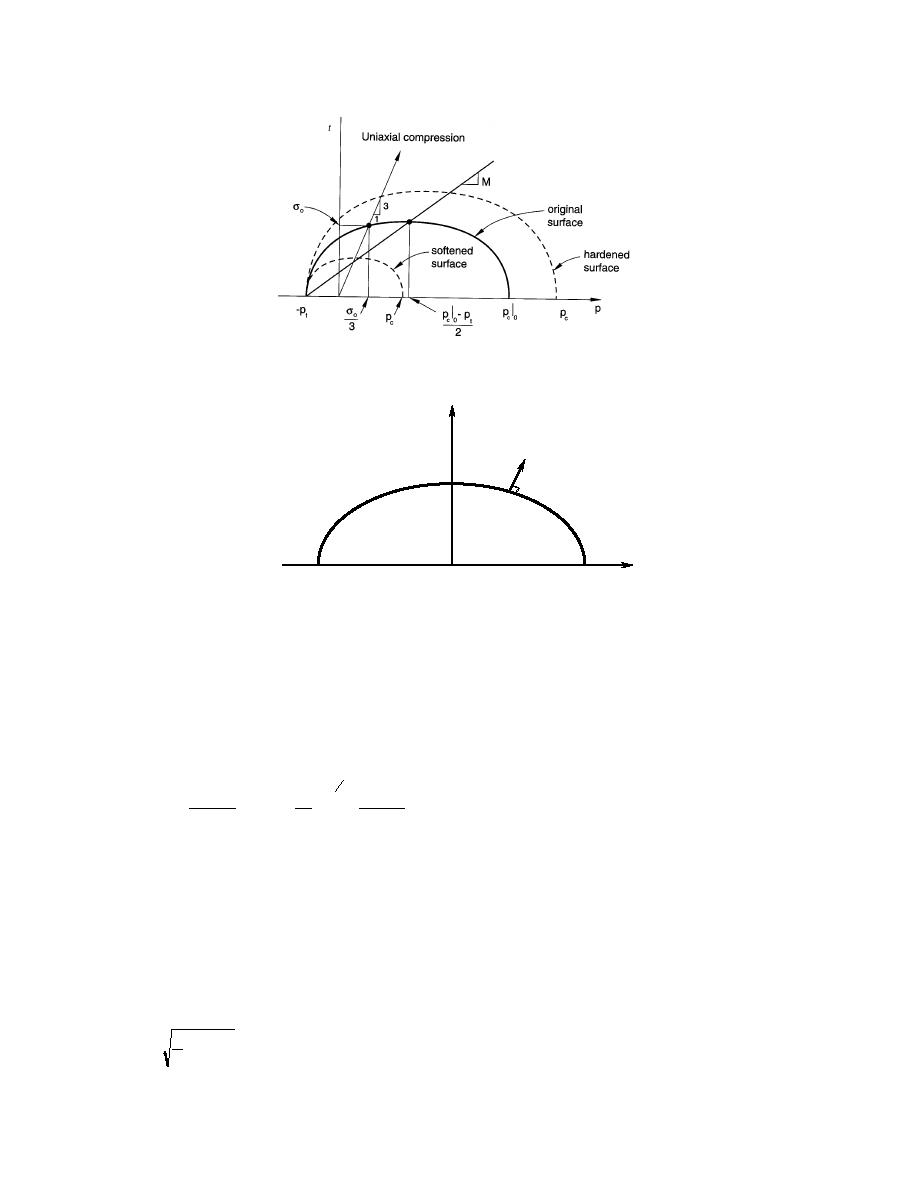
Crushable foam model
This assumes a flow direction identical to the
stress direction for stress paths along the p axis,
The crushable foam model is a class of critical
which suggests that loading in any principal direction
state model and has many similarities to the form of
results in insignificant deformation in the other direc-
the DruckerPrager cap model. The crushable foam
tions (HKS 1998). The validity of this assumption for
model, however, has a single ellipse forming the
snow was examined as part of this study.
yield surface (Fig. 19), defined by (HKS 1998)
The hardening law takes the same form as the
Ffoam = f - f0
hardening law for the DruckerPrager cap model.
(17)
1
p - p
t
2
2 2
p + pt
Constitutive models for snow
= t
+ p + - c
c
M
Two material models were judged to have per-
2
2
formance characteristics most similar to natural fresh
snow: a DruckerPrager cap plasticity model and a
where pt is the material strength in hydrostatic ten-
critical state plasticity, crushable foam model. Liu
sion, pc is the material yield stress in hydrostatic
(1994) used the DruckerPrager plasticity model for
compression, and M is the slope of the critical state
modeling the sliding of rubber blocks on snow.
line in pt plane. As indicated in Figure 19, the yield
Mundl et al. (1997) extended Liu's work using a
surface hardens and softens with yield but the pt is
multi-surface plasticity model to optimize the snow
assumed constant (isotropic hardening).
behavior under both shear and compression. Their
In the crushable foam model the plastic flow is
intent, however, was to simulate shearing forces on
non-associative and perpendicular to the flow surface
compacted snow on roads (with a density of 500
g (Fig. 20):
kg/m3), which is a significantly different material
than encountered during cross-country mobility on
9 2
p + q2 .
g=
fresh snow (with a density of 200300 kg/m3). For
(18)
2
12



 Previous Page
Previous Page
