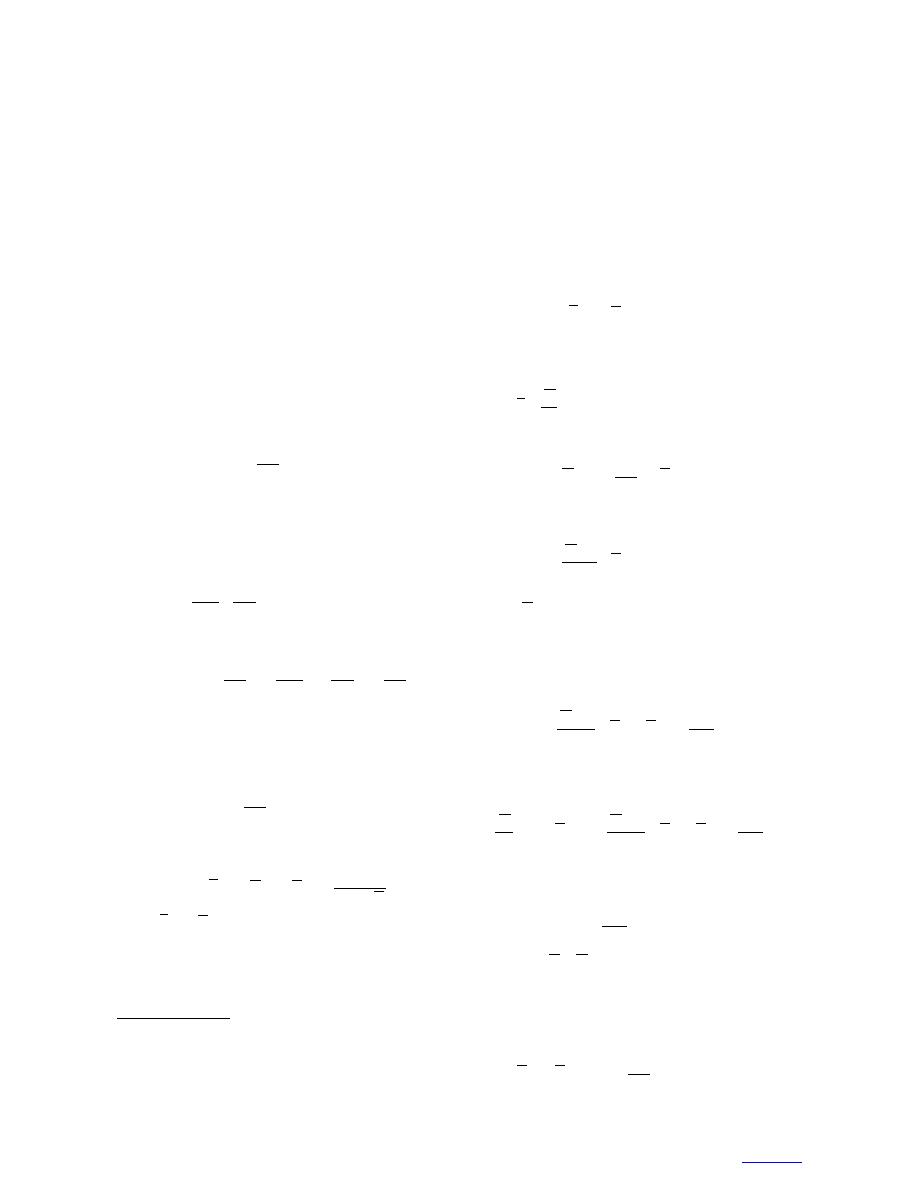
d w = -SdT + VdP - VdΠ
(53)
particles in the frost heave process by applying the gen-
eralized Clapeyron equation (GCE) to the thermody-
since Π, the osmotic pressure, is equal to RTc .
~
namic equilibrium between ice and water in soil. In
If the symbol Pw is used to describe the total soil
order to clarify that the GCE is based on sound thermo-
water potential, then
dynamics, one of Miller's students, J.P.G. Loch (1978)
published a detailed derivation of the GCE. That deri-
Pw = P Π.
(54)
vation is now summarized.
Using the Gibbs-Duhem equation (eq 31) for soil
Note that P is the pressure of the water excluding os-
ice/water equilibrium, Loch (1978) redefined the chem-
motic effects and
ical potential as the Gibbs free energy per unit mass of
a substance so that ηi refers to mass and not moles of a
d w = -S dT + V dPw .
substance, i:*
(55)
ηwdw = SdT + VdP ηsds
Equation 55 is integrated after making the substitu-
(48)
tion that at equilibrium,
where the subscripts w and s refer to water and salt,
H
S=
respectively. The equation for the chemical potential
(56a)
T
for salt in soil water (a form of eq 25) is
to obtain
RT
s =
s (T, P) +
o
∆T
ln xs
(49)
Ms
w = H ln1 +
+ V pw
To
where so(T, P) is the chemical potential of the pure
or for small ∆T,
salt at the same temperature and pressure as the sys-
tem, Ms is the molecular weight of the salt, and xs is the
H∆T
w ≈ -
+ V Pw
mole fraction of the salt. Making the approximation that
(56b)
To
M η
xs ≈ w s
Ms ηw
where H is the enthalpy per unit mass of the solution.
In eq 56a, To is the freezing point of pure water and
∆T is the freezing point depression (K).
we obtain
Assuming that ice contains no solutes, the chemical
RT Mw RT ηs
s = s (T, P) +
+
o
ln
ln
.
potential for pore ice is
Ms Ms Ms ηw
Hi ∆T
A
(50)
i = -
+ Vi P + Vi Ψiw r .
(57)
V
Differentiating eq 50 with respect to ηs gives ds =
To
(RT/Ms)(1/ηs)dηs, or
Setting the chemical potentials of pore ice and water
equal (at equilibrium) results in
η
ηsds = RTd s .
(51)
Ms
H
H ∆T
A
- ∆T + V Pw = - i
+ Vi P + Vi Ψiw r . (58)
V
To
To
Substituting eq 51 into eq 48 gives
Using the following definitions for the pressure of ice,
ηs
d w = -S dT + V dP - V RTd
(52)
Pi, and the latent heat of fusion of water per mass,
MsηwV
A
Pi = P + Ψiw r
where S and V are entropy and volume of solutions per
(59)
V
gram of water, respectively. Since the expression in the
Lf = H - Hi
parentheses of eq 52 is equal to the concentration of
(60)
~
the solute in solution, c , eq 52 can be rewritten as
and substituting eq 59 and 60 into eq 58 results in the
equation of chemical equilibrium between pore ice and
water, or the generalized Clapeyron equation:
* Loch (1978) apparently redefined the chemical potential in this
way in order to arrive at the generalized Clapeyron equation (eq 61)
∆T
in a form that is convenient to work with because the specific vol-
Vi Pi - V Pw = - Lf
.
(61)
ume of a substance is equal to the inverse of its density.
To
11





 Previous Page
Previous Page
