hypothetically explains the observed delays of approxi-
1 (z + zp )ki kp
(
)
φ∆z
∇p + ρlg
=-
mately three and seven days in Figure 11. We recall
(10)
kp z + ki zp
dt
that slow equilibrium times also prevented complete
resaturation on the wetting cycle for runs 1 of both
where is the viscosity of water (1.789 103), g the
samples. In these cases, water continued to enter the
gravitational constant (9.8 m s2), ρl the density of water
ice after the next drying cycle was initiated (e.g., dry-
(1000 kg m3), and ∇p the pressure gradient. The quan-
ing curve in Fig. 10). Solution of eq 11, subject to the
tity in the brackets is the mean harmonic permeability,
observed delay times and a rise height z of hi, yields
extremely low ice permeabilities of around 4 1019
where ki is the ice permeability, zp the thickness of the
and 1 1018, respectively, for runs 2 and 3. Corre-
porous plate, and kp the plate permeability. Pressures
at the top of the rise z and at the bottom of the porous
sponding air entry values are 4.3 and 3.4 kPa. Appen-
plate are respectively the nodal entry tension, PWn,
dix A provides details on the solution.
and the gravitational pressure, ρlg(h0 z′). These act
Once the sample is saturated, water rises rapidly to
over the combined distance hp + z. Integrating eq 10
fill the air gap. Tension at the top of the rise (PWn) is
we obtain an implicit equation of rise height in terms
now 0 and the porosity is 1. We determine the thick-
of time
ness of the air gap tg from the observed volumes of
water re-entry during phase 2. Note that the area ratio
P - ρlg zc
z
B
=t
- 2 log 0
Ar is now close to 1, since we limit flow to the air gap.
(11)
Aki A
P0
Solution of eq 11 for a rise height of hi and for the
observed times to complete phase 2 yields
permeabilities of 1 1015 and 3 1015 m2. Thus, the
where
-c ρlg
predicted permeability of the newly frozen ice in the
A=
φ
air gap is much larger than that for the central core.
In order to not "over-fit" the data, we use the same
P0 + ρlgc ki tp / kp
permeabilities for phase 3 that were determined for the
(12)
B=
central core in phase 1. These ki values slightly
φ ki
overpredict the flow rates for runs 2 and 3. With b = 4,
a middle ki value of 7 1019 m2 corresponds to vein
and where P0 = PWn + ρlgh0 , and c = (1 + φAr). With
doublet widths of 31 and 8 m. These widths are in
varying parameters and boundary conditions (Table 2),
line with values suggested by the MC runs.
eq 11 describes the three flow phases observed in Fig-
The higher permeabilities for phase 2 are consistent
ure 11. We explain the three phases as follows: 1) water
with greater vein dilation in ice at the sides of the
rises under tension within the central ice core until the
sample, but also suggest that by comparison ice in the
veins are saturated, 2) water rises under a gravitational
central core underwent structural changes during the
head to fill the air gap, and 3) water flows to a seepage
experiments. All four runs with sufficient data for analy-
face at the top of the ice sample.
sis showed long equilibrium times, on the order of
The hypothesis of unsaturated flow in phase 1 is the
weeks, for the wetting curve. We speculate that vein-
most speculative of the three scenarios. Flow under ten-
wall melting during the desaturation process may have
sion prevents entry of water into the side air gap and
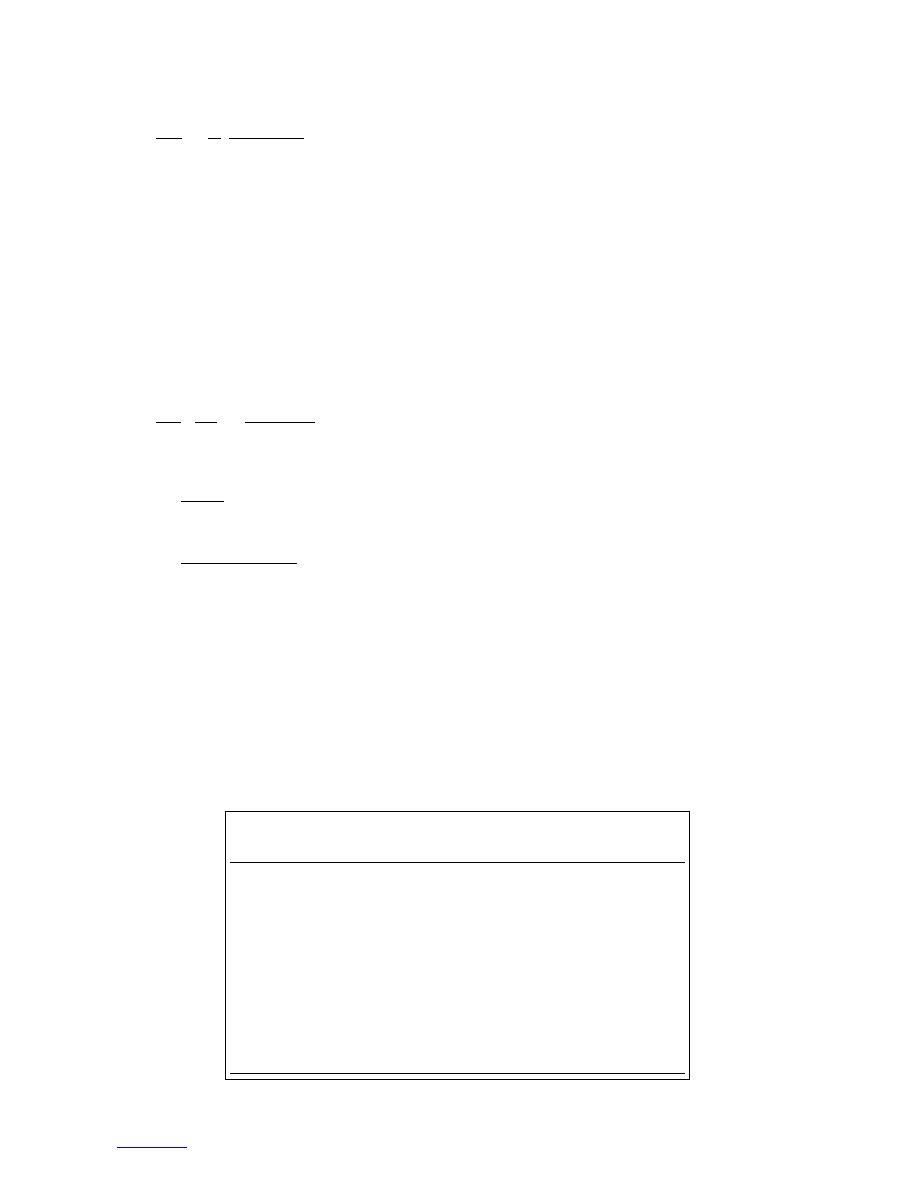
Table 2. Parameters used in computing flow rates through Sample B.
Phase 1
Phase 2
Phase 3
hp (m)
0.00635
hi (m)
0.043
Rc (m)
0.043
6.35 1016
kp (m2)
h0 (m)
0.25 0.29
0.25 0.29
0.21 0.25
Ar
58.1
0.837
0.884
58.1
tg (m)
0.0003
0.0003
0
4 1019 1 1018
1 1015 3 1015
4 1019 1 1018
ki
b
4.0 4.0
na
na
Φ
0.001 0.001
1.0
1.0
Pwn (kPa)
4.3 3.4
0
0
12





 Previous Page
Previous Page
