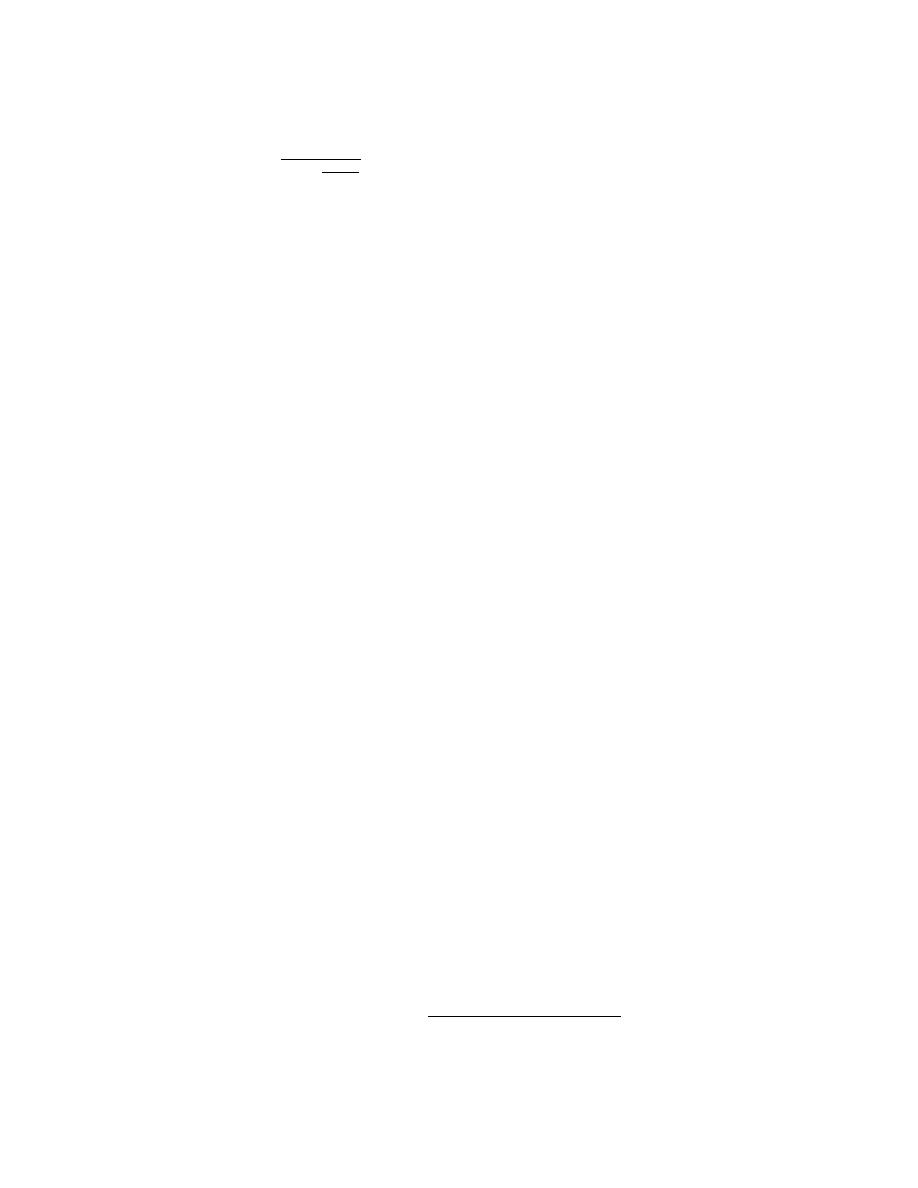
The solvent and solute mole fractions could be calculated by:
mTf
xNaCl =
mTf +
1
(23)
MH2O
xH O = 1 xNaCl
(24)
2
where MH2O is the molar mass of water (0.018 015 28 kg mol1).
Freezing point of the solution phase in bulk
We fitted by regression the freezing-point depression data calculated by the For-
T0 = 273.15 K f mNaCl (aq)
(25)
where Kf is an approximate cryoscopic constant for the H2ONaCl system, which
we found to be 4.12207 K kg mol1. This compares with the expected cryoscopic
constant for a single electrolyte completely disassociated in water of 3.72 K kg
mol1 (Atkins 1990).
Molar entropy of ice
The quantity Sm,H2O ≡ Sm [H2O(cr, I)] at a temperature and pressure of interest
∗s
∗
can be calculated by adding the entropy changes due to cooling and freezing to the
entropy of water at a reference point (i.e., Tr = 273.15 K and pr = 0.1 MPa). This
quantity is calculated by evaluating
[
]
[
]
Sm, pr H2O(cr, I) = Sm,Tr , pr H2O(l)
*
*
(26)
[
]
+ ∆T r 273.15 Sm H2O(l), pr
=
∗
(27)
T
+ ∆lcr,I Sm (H2O, T = 273.15, pr )
∗
(28)
[
]
+ ∆T =273.15 Sm H2O(cr, I), pr .
∗
(29)
Tf
The starting point of the calculation (eq 26) is the molar entropy of pure water at
a reference temperature (Tr = 273.15 K) and pressure (pr = 0.1 MPa), for which the
*
1985).
Haida et al. (1974) reported the entropy change due to the constant-pressure
cooling of water from 298.15 K to its freezing point, ∆T =273.15Sm [H2O(l), pr ] = 6.615
∗
Tr
0.01 (eq 27). This quantity can also be calculated with the equation-of-state model
for water (Hill 1990):
SH 2O(l) (Trw , prc ) SH 2O(l) (Trc , prc ) = 6.616 04 J K 1 mol 1
*
*
(30)
We used the latter value in our calculations.
*
is calculated by
∆lcr,I Hm (H2O, T = 273.15, pr )
*
(H2O, T = 273.15, pr ) =
∆lcr,ISm
*
.
(31)
273.15
Haida et al. (1974) reported a molar enthalpy of melting of 6006.8 J mol1. Giauque
and Stout (1936) earlier reported a value of 6007.0 3.8 J mol1.
13



 Previous Page
Previous Page
