sign would be sufficient to allow drainage of a
are given below:
day's dredging supernatant in a reasonable
amount of time, six to eight hours, without veloci-
H = 0.1, 0.098 ... 0.01 (Head, or height of
ties that would resuspend the poorly consolidated
water above weir in meters)
settled solids or damage the filtering fabric located
P = 0.15, L = 3.2 (Height and length of weir
2 m behind the weir.
in meters)
g = 9.81 (gravitational constant, m/s2)
The following analysis of the drainage of the
Q(H) = 2.54 [L 0.2(H)] (H)1.5 (3600) (Flow rate,
Spoils Retention Basin, sited on the EOD at Eagle
m3/hour [Hicks 1972]).
River Flats, Alaska, uses the Francis Formula for
(3)
fluid flow over a rectangular, sharp-edged weir.
In a normal eight-hour dredge cycle, approxi-
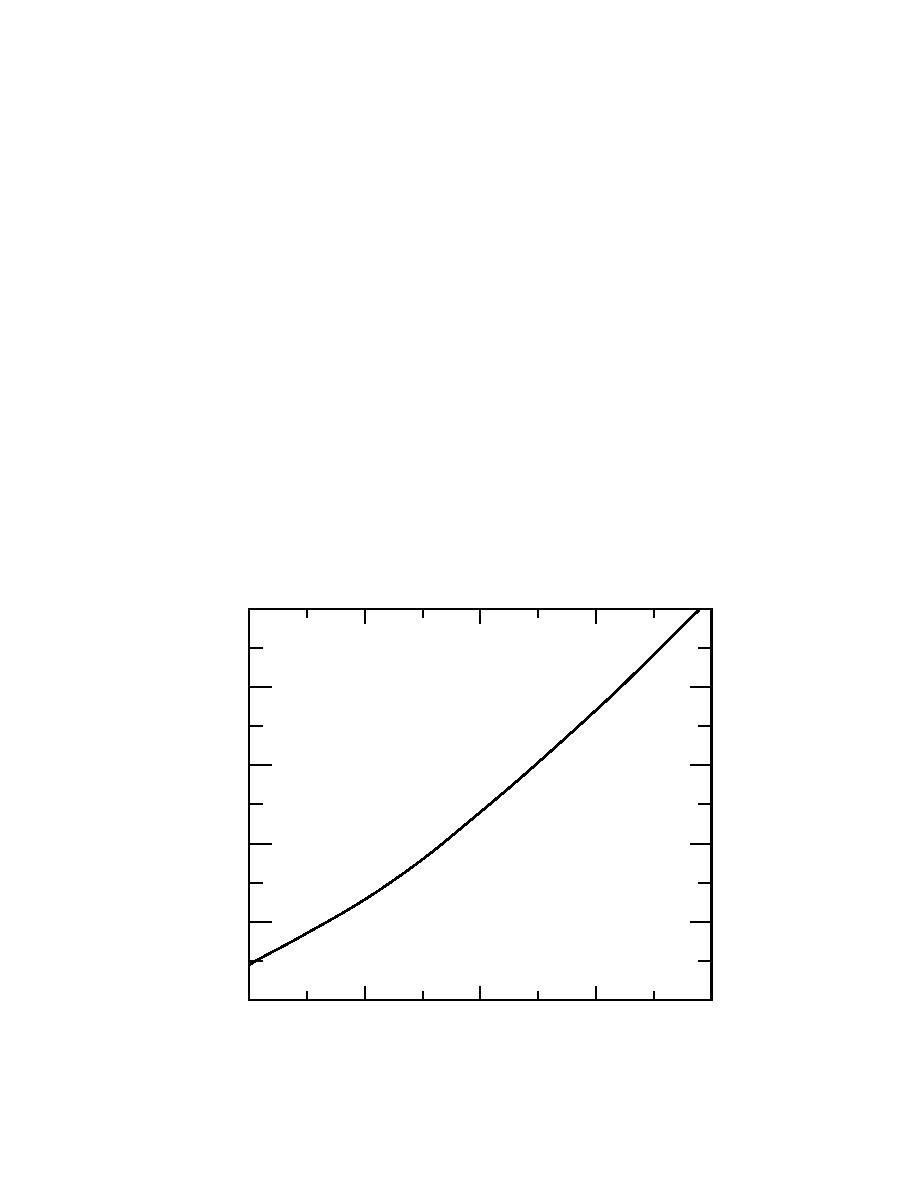
Figure 5 is a graph of the instantaneous flow rate
mately 2400 m3 of water will be pumped into the
over a 3.2-m (10 ft) weir. The volume retained in
retention basin, assuming a 380-m3/hour produc-
the 0.8-ha retention basin above the weir can be
tion rate and a 4:1 ratio of water to spoils (by vol-
expressed as
ume).
V(H) = AH = 2 (4047) H (m3).
The initial analysis is of flow rates over a 3.2-m
(4)
weir for a given head. The height of the weir is
given as about 15 cm. Although the weir height
One of the primary concerns in the retention
affects the flow rate, it will not be considered here,
pond design is the flow over the weir, which will
as the effect is minimal for low heads (< 0.3 m).
be impacting the silt fence located between the
We will be operating with heads of 0.1 m or less.
weir and the basin outflow culvert. To get a
The reason for this is to minimize the turbulence
handle on this, we can analyze the effect of differ-
in the sheet flow towards the weir. Boundary con-
ing weir length for a given head on the flow rate,
ditions and the instantaneous flow rate equation
in cubic yards per hour. This is an extension of
50
40
30
Q(H)
(m 3/hr)
20
10
0
0.02
0.04
0.06
0.08
0.10
H, Head (m)
Figure 5. Instantaneous flow over a rectangular weir.
9



 Previous Page
Previous Page
