tionships in Table 1. In most cases an iterative solution will be required to deter-
mine the air temperature upon which to base thermal properties, if unknown, and
the temperature of surface 2. In general, the air properties can be evaluated at the
average interior surface temperatures. If the effective conductivity relation includes
tion, appropriate emissivities can be selected for use in eq 55; for those that do,
information on the emissivities values used to develop the correlations is limited;
the available data are noted in Table 1.
A number of investigators (Zirjacks and Hwang 1983, Phetteplace et al. 1986,
Kennedy et al. 1988) measured temperatures and heat flows in and around
utilidors. These measurements were generally extensions of modeling efforts and
analysis was limited to confirmation of the conduction models used to predict soil
temperatures around the utilidors.
NUMERICAL MODEL
A numerical model using a finite-element approach was developed to solve the
momentum, energy, and continuity equations in two dimensions for the steady-
state case. The following assumptions were made:
The fluid (air) is Newtonian and incompressible within the Boussinesq ap-
proximation. (Fluid properties are constant, except for density, which is a func-
tion of temperature and affects only the buoyancy term.)
Fluid flow is laminar.
Thermal conductivity is constant for each fluid/material.
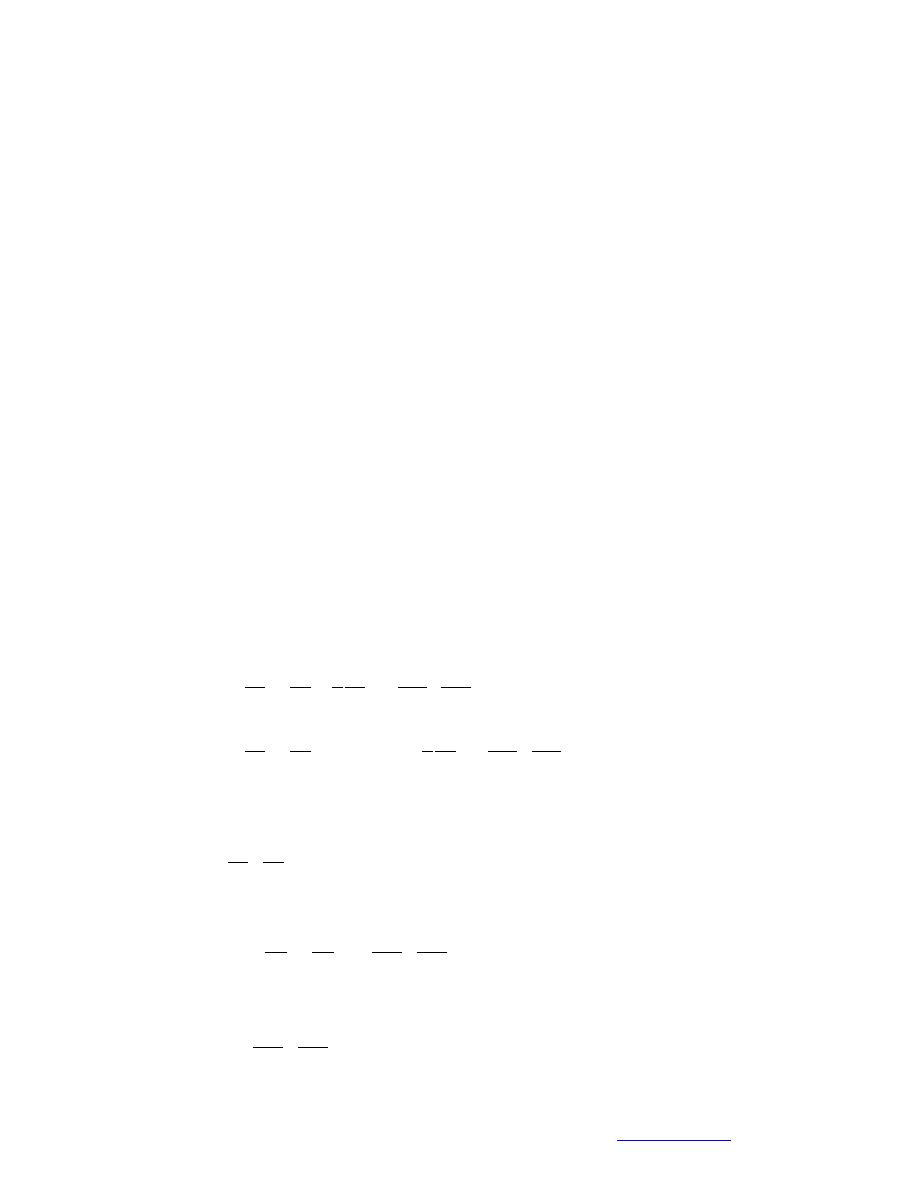
Following Gartling (1977) and Jaluria and Torrance (1986), the momentum equa-
tions are, as stated earlier,
2u
2u
u 1 p
u
+v
+
- υ 2 +
=0
(58)
u
y ρ x
y2
x
x
2v
2v
v
(
)
1 p
v
+ u - gβ T - Tref +
- υ 2 +
=0
(59)
v
y2
ρ y
x
y
x
where y is in the vertical direction and x is in the horizontal direction. The conti-
nuity equation is
u
v
+
=0
(60)
x
y
and the energy equation is
2T
2T
T
T
+u
- k 2 +
-Q = 0,
(61)
Cv v
x
y2
x
y
which becomes for a solid region without convection
2T
2T
-k 2 +
- Q = 0.
(62)
y2
x
13
Back to Contents





 Previous Page
Previous Page
