Equilibrium thickness evaluations
To compare thicknesses after failure and thicknesses predicted from equilibrium
theory, the strength and hydraulic roughness properties of bead accumulations had
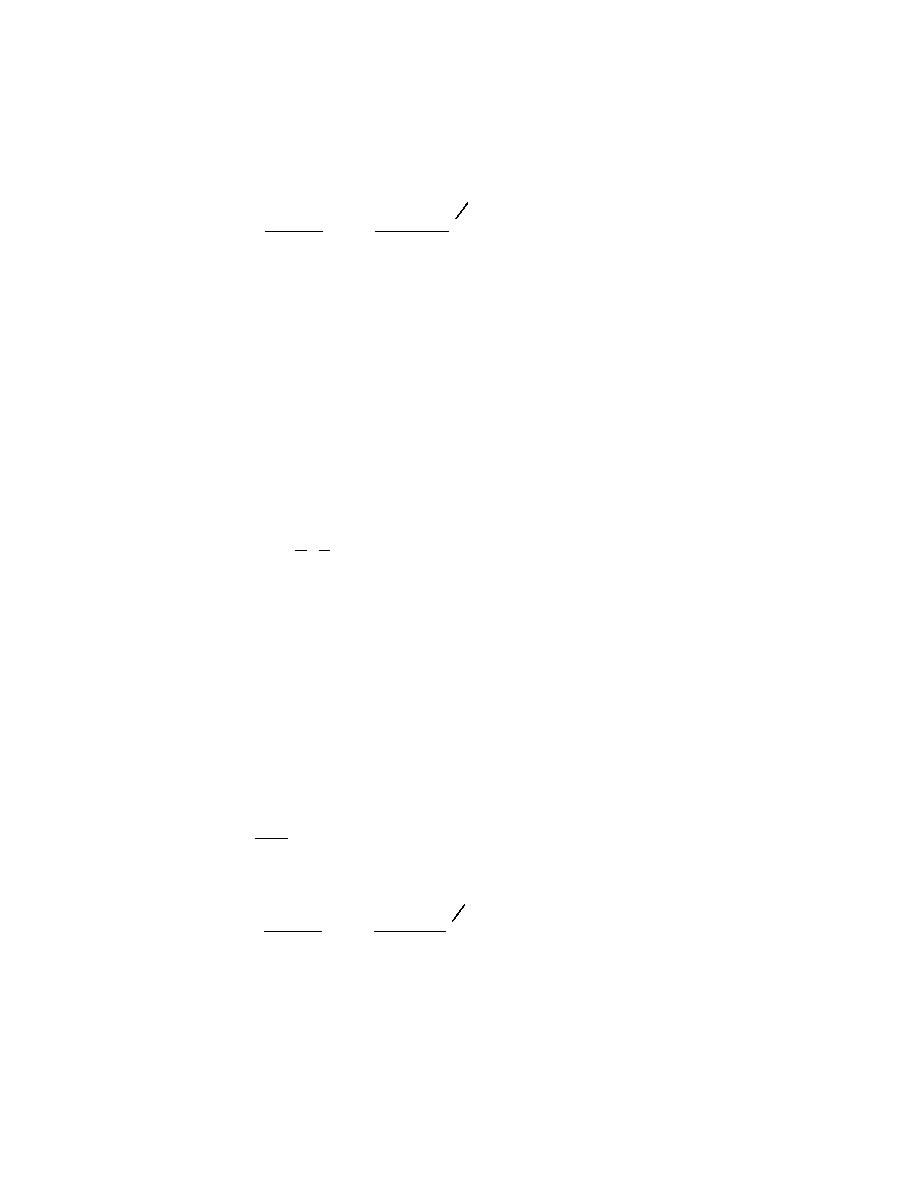
to be determined. A useful formulation of static equilibrium thickness is
1
fiu (1 - si )
2
2
BS
ηeq =
1 + 1 +
(26)
2(1 - si )
2
2BS si g
flow along the ice cover underside. The overall strength coefficient for the ice is a
combination of several material properties, i.e.
= k0λKp (1 - p)
(27)
where
k0 = lateral stress coefficient (the fraction of the longitudinal acting force that
is directed normal to the banks)
λ = friction coefficient for ice sliding against ice at a shear boundary
p = accumulation porosity
Kp = Rankine passive pressure coefficient
π φ
Kp = tan2 +
(28)
4 2
with φ being the angle of internal resistance, which is commonly assumed to be
equal to the dry angle of repose of a granular material.
Calculation of equilibrium thickness using eq 26 requires knowledge about the
average velocity and energy slope of the flow, as well as about the specific gravity
of the ice, accumulation porosity, friction factor for flow along the accumulation,
and the overall strength coefficient . For jam equilibrium, bed slope, water surface
slope, and energy slope are taken as being equal. While values of angle of internal
resistance φ and porosity p had been directly measured for the beads, values of
cannot be readily calculated from eq 27. Instead, values were back-calculated using
eq 26, but this approach involves fi as an additional unknown. However, the Darcy-
Weisbach definition of friction slope for the ice-affected layer of flow
fiu2
Sf =
(29)
8gRi
combined with eq 26 leads to an alternate form of eq 26
1
4Ri(1 - si ) 2
BS
ηeq
=
1 + 1 +
.
2(1 - si )
(30)
siBS
For this equation, only values of slope and hydraulic radius of the ice-affected flow
area are needed to evaluate ηeq.
Multi-layer bead accumulations, or jams, were allowed to form in the flume at
different levels of steady discharge. Detailed slope measurements and velocity pro-
files were obtained in the region where the accumulation thickness appeared uni-
form. Figure 18 presents an example of a measured velocity profile and the fitted
25



 Previous Page
Previous Page
