coupled numerical model of ice jam formation. Such a model includes both ice
velocity and the effects of ice momentum on the force balance, utilizing the full
conservation of mass and momentum equations for the ice.
FORMULATION
Introduction and assumptions
Formulated here are the one-dimensional, unsteady flow equations for water
and ice. The equations, derived in integral form, are based on the conservation of
mass and momentum for water and ice flow during jam formation and breakup.
The integral equations are then discretized as finite-difference equations, approxi-
mating the conservation laws in their integral form. The equations are expressed in
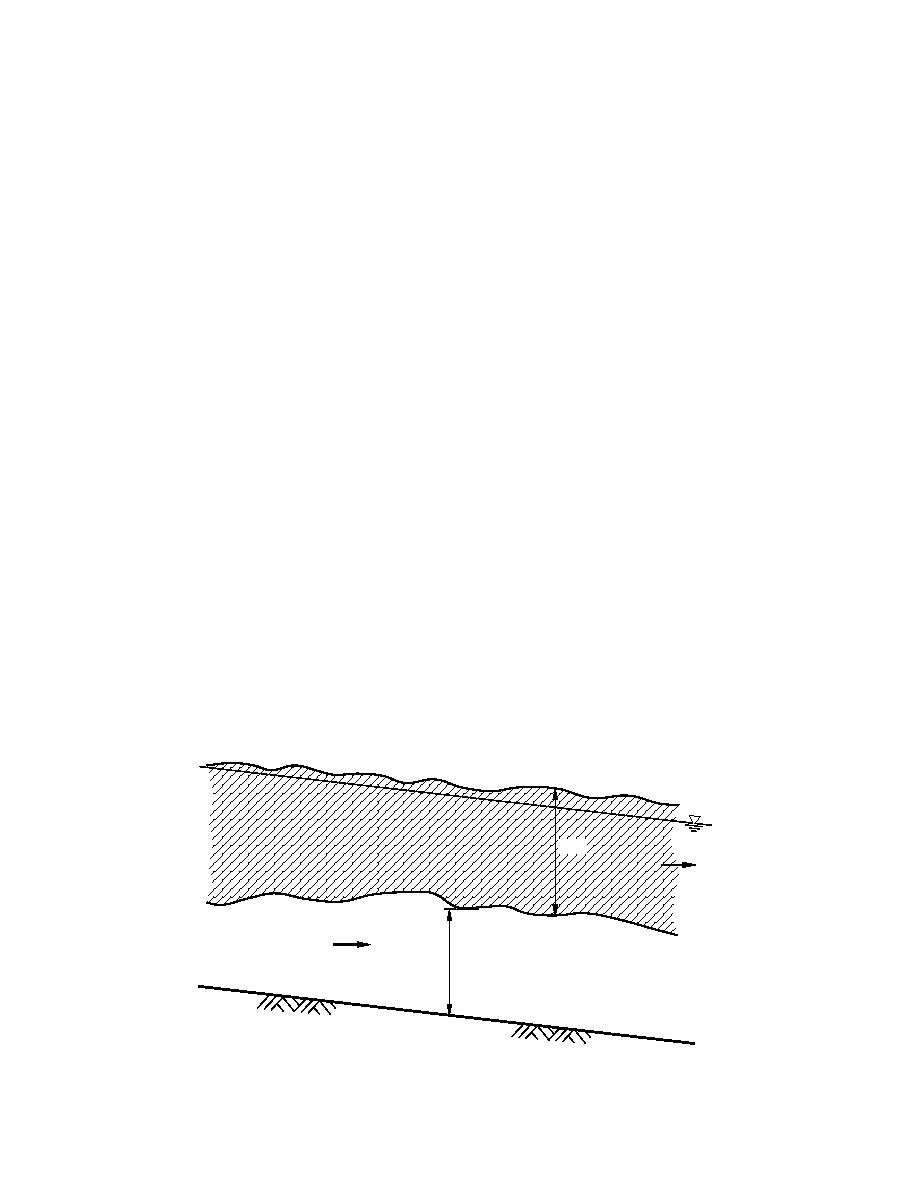
terms of four dependent variables that fully describe the flow, as shown in Figure
23, namely the velocities of the ice cover and under-ice water flow (υ and u, respec-
tively), the ice cover thickness (η), and the under-ice water depth (d). All four vari-
ables are functions of time and space. The equations are derived first in a general
form, and then simplified in accordance with the assumptions listed below. Addi-
tional simplifications for certain flow conditions are discussed subsequently.
The assumptions made in developing the equations for water and ice flow
include the usual St. Venant assumptions for one-dimensional flows (e.g., Cunge et
al. 1980) are as follows:
Flow is one-dimensional, with velocity uniform across each cross section, and
water level horizontal at each cross section.
Streamline curvature is small and vertical accelerations are negligible, so that
pressure distribution is hydrostatic.
The effects of turbulence and boundary friction can be accounted for through
resistance laws identical to those used for steady-state flow.
Average channel-bed slope is small so that the cosine of the angle it makes
with the horizontal may be taken as unity. (This assumption is valid for bed
slopes to about 0.01.)
Ice
η(x,t)
υ(x,t)
Water
u(x,t)
d(x,t)
Bed
Figure 23. Ice and water flow.
29



 Previous Page
Previous Page
