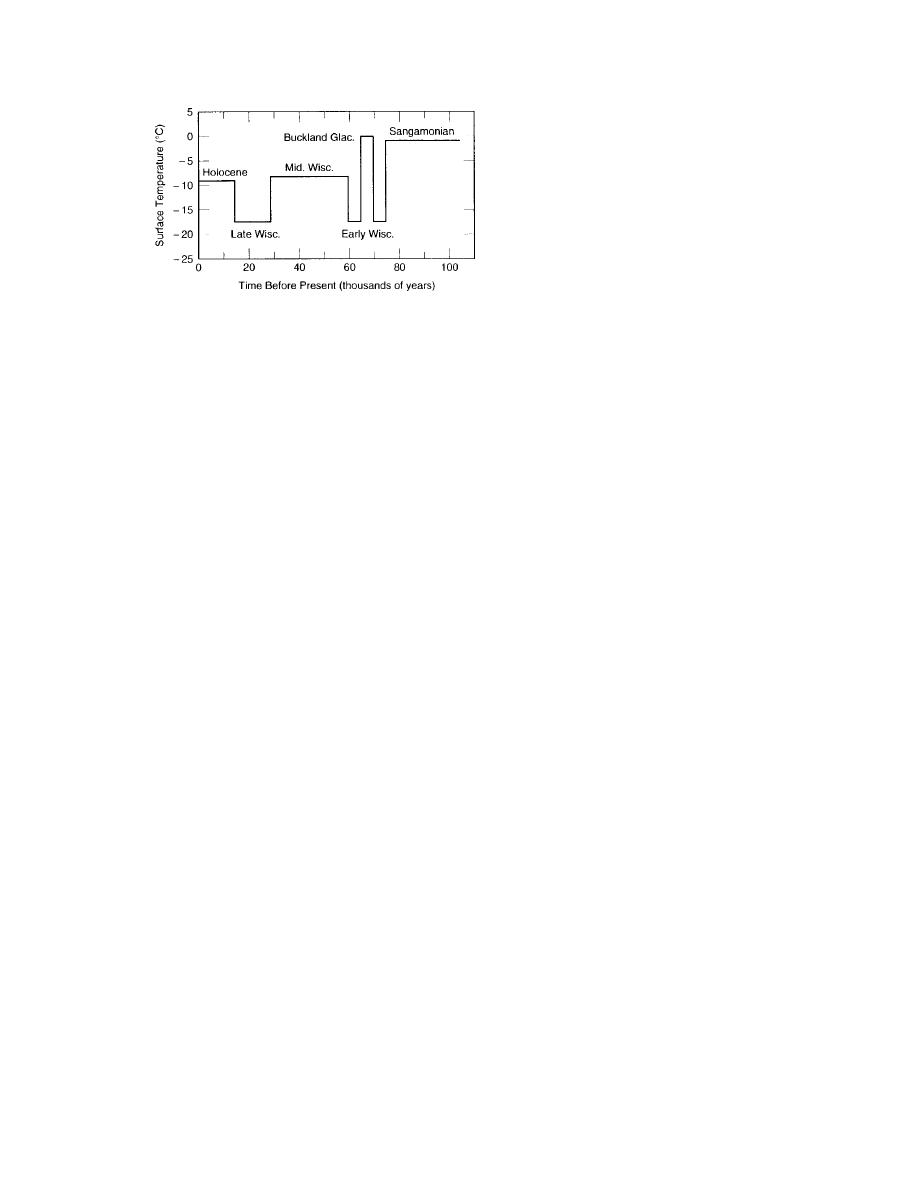
Figure 8. Paleotemperature history at Macken-
zie Delta, Canada (after Allen et al. 1988).
glacial intervals. Figure 7a is based on the Vostok ice core while Figure 7b is calculated from information
given by Brigham and Miller (1983) for Prudhoe Bay, Alaska.
There have been surprisingly few systematic thermal studies of the origin of permafrost and the total
time required for its formation. Osterkamp and Gosink (1991) studied the response of permafrost thickness
to surface temperature variations. They used quasi-steady and numerical models to predict the position of
the permafrost bottom, with arbitrary initial permafrost thicknesses, but were interested in the inverse prob-
lem of deducing paleotemperatures from the present permafrost data at Prudhoe Bay, Alaska. Allen et al.
(1988) used a quasi-steady model (which will inherently underpredict the growth time) for the same pur-
pose in the Mackenzie Delta region of Canada, using still another paleotemperature history (Fig. 8). They
assumed that Illinois permafrost was completely melted during the Sangamon interglacial, although this is
highly unlikely given the time available, unless Illinoisian glaciation limited the permafrost thickness to
modest values. Nevertheless, they used an initial permafrost thickness and predicted about 800 m of growth
in 75,000 years. Their thermal model is based on the work of Lachenbruch et al. (1982) dealing with the
rate of thaw of thick permafrost zones during several millennia. Romanovsky et al. (1988) noted qualitative
aspects of the origin and disappearance of permafrost in the Transbaikal area of Russia. Katasonov (1988)
used "cryogenic structures" to argue for the origin of permafrost early in the Quaternary and its persistence
up to the present.
In this report an attempt is made to predict the rate of permafrost formation, starting with no permafrost,
i.e., its origin, using a simple conduction model. If the soil forming the permafrost exists before freezing
starts, the growth is heterogenetic. When the permafrost forms as the soil material is gradually deposited at
the surface, the permafrost is said to have a syngenetic origin. The thermal conditions for each type of
growth will be examined.
THEORY
The solution to conductive heat transfer problems, with solidification phase change, has interested engi-
neers and mathematicians for over a century. These problems (often referred to as Stefan problems) are in-
herently nonlinear and solution methods are very restricted. A classical solution for the case of a constant
temperature, semi-infinite medium that undergoes a step change of surface temperature was given by Neu-
mann (1860) and expanded upon by Carslaw and Jaeger (1959); it is called the Neumann solution. Tao
(1978) extended the similarity technique of Neumann to the semi-infinite slab with arbitrary initial temper-
ature. This is precisely the solution we need, but unfortunately this exact solution is such that numerical
computations are extremely difficult because of transient functions that require an increasing number of se-
ries terms as time increases. For permafrost formation the time scales are so huge that Tao's solution is im-
practical and cannot be used. Like the exact solution of Lozano and Reemsten (1981), for flux boundary
conditions, Tao's solution is perhaps best used numerically to verify the accuracy of approximate and nu-
merical solutions or for short-time solutions.
The search for practical solutions for engineering design has led to some convenient approximate solu-
tion methods for Stefan problems. The heat balance integral technique solves the energy equation on aver-
6





 Previous Page
Previous Page
