the bonds must be simple grain boundaries with
grain-boundary grooves. Kuroiwa's (1962) Figure
9 shows bonding between polycrystalline grains,
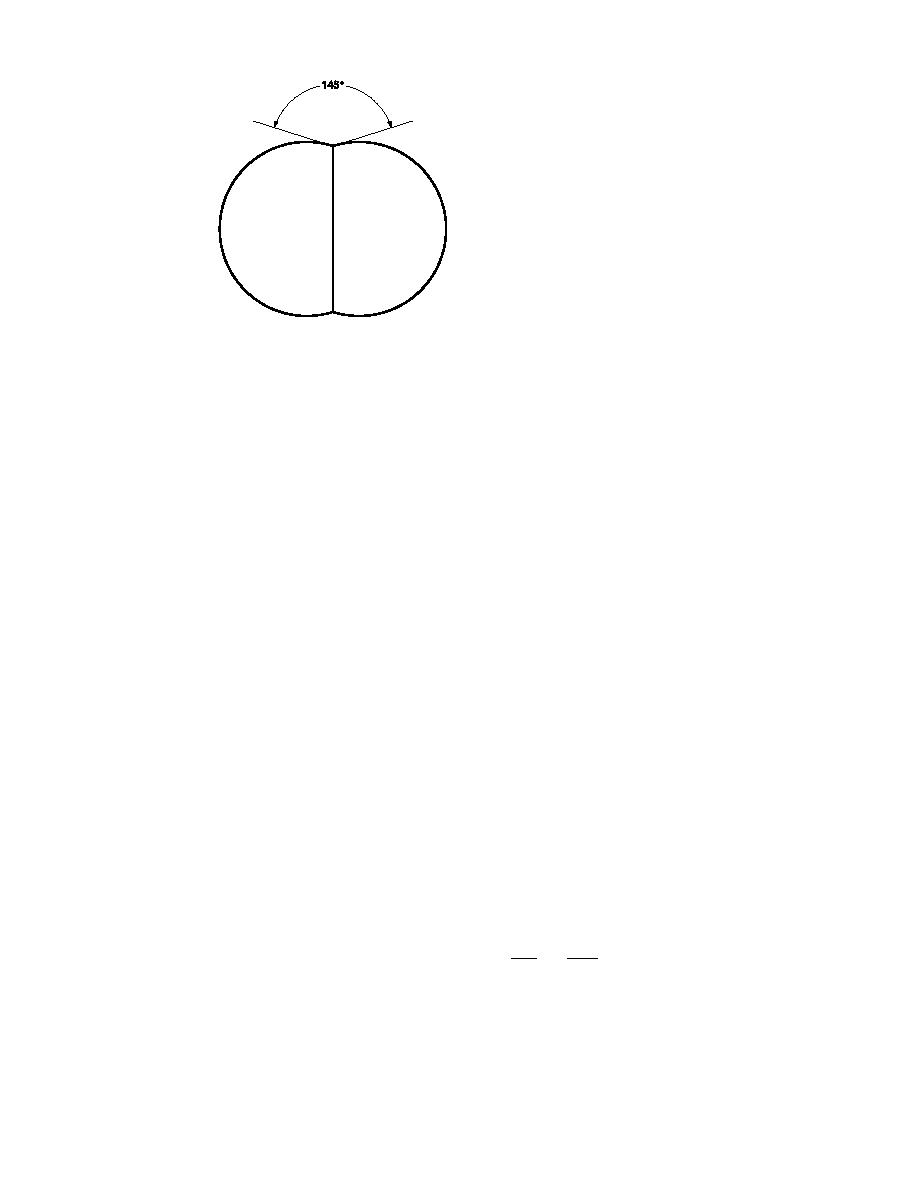
Figure 9. Equilibrium
but the actual bond appears to connect only two
form of two grains
of the crystals, one within each grain.
of ice consisting
When two ice grains consisting of a single crys-
of single crystals
tal each are joined by a bond, the equilibrium
where the grain-
form of the arrangement should be as shown in
boundary groove
Figure 9. For the old grains shown in Figure 7b,
angle is 145. Ac-
the grain-boundary groove angles are about 138
tual bonds may
and 148, close to the equilibrium value observed
not have had time
by Ketcham and Hobbs (1969). For the fresh snow
to achieve this
shown in Figure 8, the grain-boundary groove
configuration.
angle appears to be much smaller because the
bond has not yet had sufficient time to approach
the equilibrium condition. However, a closer ex-
amination of this bond could prove that the actual
Keeler (1969) found that the rate of bond growth
angle is closer to the equilibrium value.
was much greater in natural snow than predicted
Zhang and Schneibel (1995) described the sin-
by sintering theory. Although he attributed this
tering of grains joined by grain-boundary grooves.
to stresses in the snow, the effect of stress on the
They explained the imbalance of forces that oc-
thermodynamics is very small and thus it seems
curs in the grain-boundary groove before the equi-
likely to me that this is due to the presence of a
librium condition has been established; they
macroscopic temperature gradient. These are al-
assumed that the dihedral angle was fixed
ways imposed on the snow cover by environmen-
throughout the process. Sintering in their model
tal factors, and thus water vapor is driven through
was limited to grain boundary and surface diffu-
the snow. This enhanced vapor movement should
sion and expressing their results in terms of the
be much greater than vapor movement due to
diffusivity ratio of these two processes. Unfortu-
differences in curvature or stress. A much greater
nately, the values for the diffusivities are still
rate of vapor flow should cause faster bond
uncertain for ice, so it is not possible to calculate
growth, just as it causes faster grain growth
meaningful rates of bond growth based on these
(Colbeck 1983b). While the bonds must move to-
processes. Furthermore, in seasonal snow covers
ward their equilibrium shape, the rate at which
it seems likely that the shape is determined by the
the bonds grow is probably controlled by vapor-
requirement for equilibrium, but the rate is deter-
density gradients caused by macroscopic tempera-
mined by vapor flux due to the macroscopic tem-
ture gradients, not by microscopic curvature or
perature gradient. In spite of these limitations,
stress differences. Without an imposed tempera-
the ideas expressed by Zhang and Schneibel (1995)
ture gradient, depth hoar could not grow at all in
are clearly applicable to sintering in snow and, if
snow, and rounded crystals would grow much
fact, are vital to understanding what is actually
more slowly than observed (de Quervain 1958).
observed in snow. For this reason their work on
Given that the rate-limiting factor in mass flow in
surface and grain-boundary diffusion is summa-
snow is the vapor density gradient, which is con-
rized here.
trolled by the temperature profile, the classical
Surface diffusion is proportional to the gradi-
theory of sintering may have little to do with the
ent of the chemical potential, or the gradient of
rate of formation of bonds in dry snow. This prob-
curvature along the surface. As the resulting flux
ably explains why the rate of sintering has often
reconfigures the surface, the growth or decay of
been found to occur faster than is described by
the surface is given by the gradient of the flux, so
models or laboratory experiments.
2K
The basic geometries of rounded grains and
ra
=B
(2)
their bonds are controlled by phase equilibrium.
s2
t
Thus, the shape of the bond, but not its rate of
growth, can only be understood by examination
where ra is the normal vector to the surface, K is
of the equilibrium condition at the bond. Ice grains
the surface curvature, which is positive for con-
in dry snow generally consist of single crystals, so
vex surfaces, s is length along the surface, and
7





 Previous Page
Previous Page
