where θi is a weighting factor for the ice variables
(
)
(
)
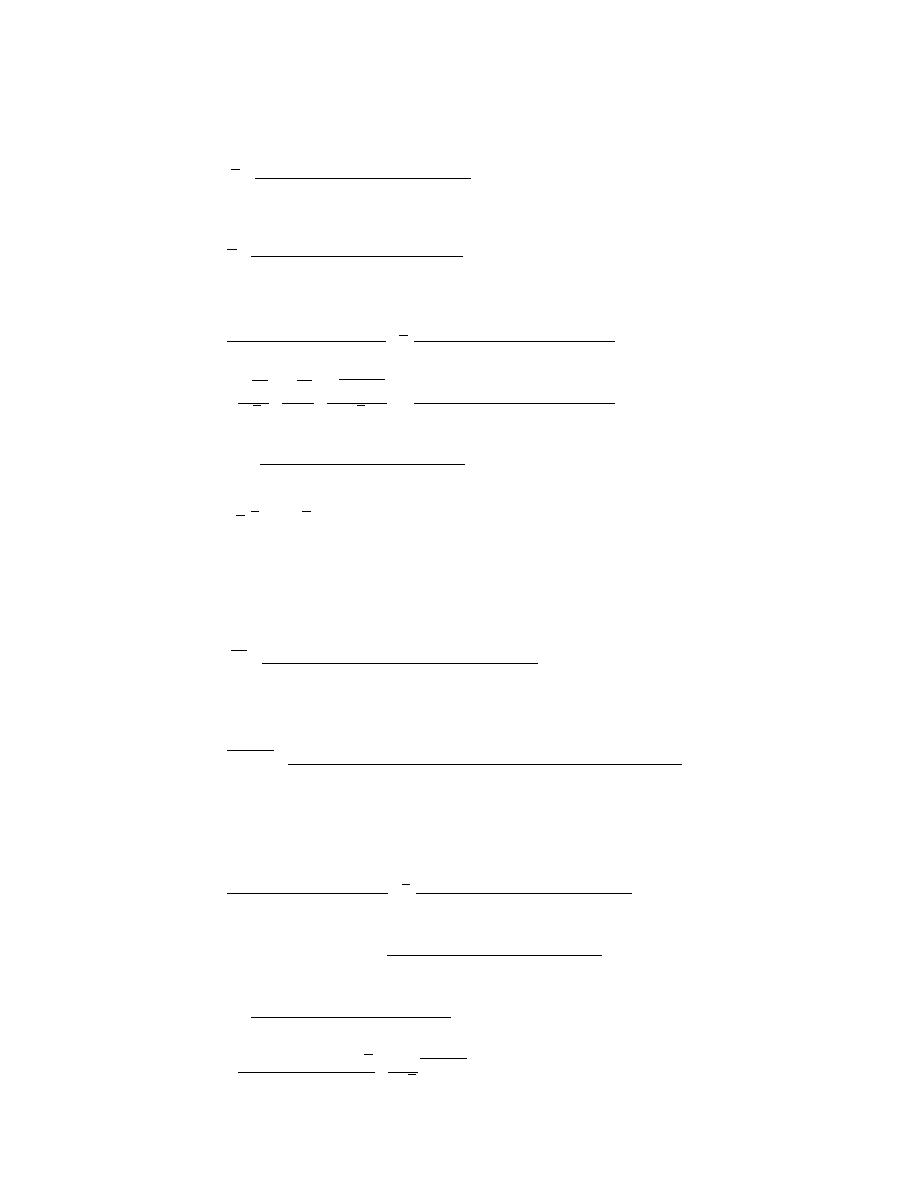
θi υn+1 + υn+1 + (1 - θi ) υn 1 + υn
j+1
j+
j
j
υ=
(124)
2
and
(
)
(
).
θi ηn+1 + ηn+1 + (1 - θi ) ηn 1 + ηn
j+1
j+
j
j
η=
(125)
2
The conservation of water momentum equation transforms to
(u
)(
) + u θ(u
)
(
)
- ujn+1 + (1 - θ) ujn 1 - ujn
n+1
+ ujn+1 - ujn 1 + ujn
n+1
j+1
+
j+1
+
2∆t
∆x
(
)
(
)
θ
djn+1 - djn+1 + (1 - θ) djn 1 - djn
fb u2 fb u2 fi (u - υ)
2
+
+1
+ g
+
+
+
∆x
4B
8d
8d
(126)
(
)
(
)
θ ηn+1 - ηn+1 + (1 - θ) ηn - ηn
j+1
j+1
+ gsi
- gS = 0
j
j
o
∆x
where u , d , and + υ are defined as above. Note that the last term in eq 114 has been
expanded for clarity and that the discretization of these terms containing squared
variables are weighted averages of the squared terms. They are not squares of the
weighted averages of the variables. This minor detail preserves the physical mean-
ing of the terms. Those terms are
()
()
()
( )
n+1
n+1
n
n
+ (1 - θ) u2
θ u2
+ u2
+ u2
j+1
j+1
u2 =
j
j
(127)
2
and
(
)
(
)
(
)
(
)
2 n+1
2 n
n+1
n
θ [u - υ]
+ [u - υ]
+ (1 - θ) [u - υ]
+ [u - υ]
2
2
j+1
j+1
=
j
j
. (128)
(u - υ)
2
2
The conservation of ice momentum equation transforms to
(υ
) - (υ
) + υ θ (υ
) + (1 - θ )(υ
)
n+1
n+1
n+1
n+1
n
n
n
n
j+1 - υj
j+1 + υj
j+1 + υj
j+1 - υj
i
i
2∆t
∆x
(
)
(
)
θ ηn+1 - ηn+1 + (1 - θ ) ηn - ηn
(
)
j+1
i j+1
+ g(1 - si )Kp (1 - p) + gsi
i
j
j
∆x
(
)
(
)
θ dn+1 - dn+1 + (1 - θ) dn - dn
j+1
j+1
+ g
- gS
j
j
(129)
o
∆x
g(1 - si )k0λKp (1 - p)η
fi
(u - υ)2 = 0.
-
+
8si η
B
46



 Previous Page
Previous Page
