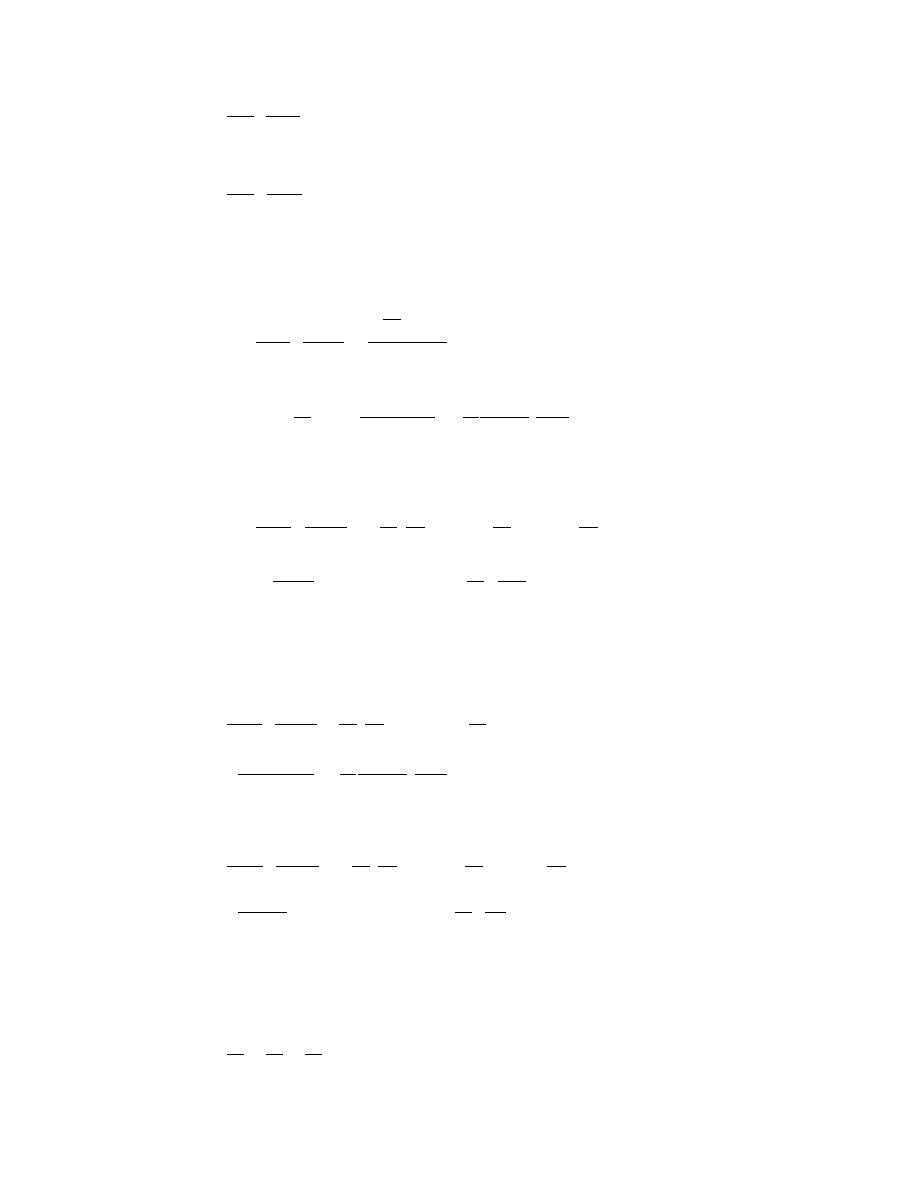
(d) + (ud) = 0.
(106)
t
x
In a similar fashion, the conservation of ice mass equation becomes
(η) + (υη) = 0.
(107)
t
x
The conservation of water momentum and conservation of ice momentum equa-
tions contain a combination of single and double integrals. By Taylor series expan-
sion as above, the conservation of water momentum becomes
d2
()
+ dsi η
2
u2d
(ud)
t2 x2
+
+g
∫∫
dxdt
t
x
x
t1 x1
(108)
u - υ
fbu2 (B + 2d)
2
t2 x2
fi
B
d
1 + f B + 2d u dxdt = 0
- g ∫ ∫ ηsi
+ dSo -
b(
)
8gB
x
t1 x1
and the conservation of ice momentum becomes
(υ η) + gs
η2
2
(υη) +
η2
t2 x2
2 + g(1 - si ) x Kp (1 - p) 2 dxdt
∫∫
t
i
x
x
t1 x1
(1 - si )
(109)
2
t2 x2
f
k0λKp (1 - p)η2 - ηSo + η
d
- i (u - υ) dxdt = 0.
+g ∫ ∫
x 8gsi
t1 x1 B
Under the assumption that these equations also hold everywhere in the (x,t)
plane, the conservation of water momentum equation is
(u d) + g
2
(ud) +
d2
d
+ dsi η - gsi η - gdSo
x 2
t
x
x
fbu2 (B + 2d)
u - υ
(110)
2
fi
B
+
1 +
= 0.
fb (B + 2d) u
8B
In a similar manner, the conservation of ice momentum equation becomes
(υ η) + gs
2
(υη) +
η2
η2
+ g(1 - si ) Kp (1 - p)
x 2
i
x
2
t
x
g(1 - si )
(111)
f
k0λKp (1 - p)η - gηSo + gη
d
- i (u - υ) = 0.
2
2
+
B
x 8si
The partial derivatives with combined dependent variables in the foregoing equa-
tions are then expanded to separate the variables. The conservation of water mass
equation becomes
u
d
d
+u +d
= 0.
(112)
t
x
x
43



 Previous Page
Previous Page
