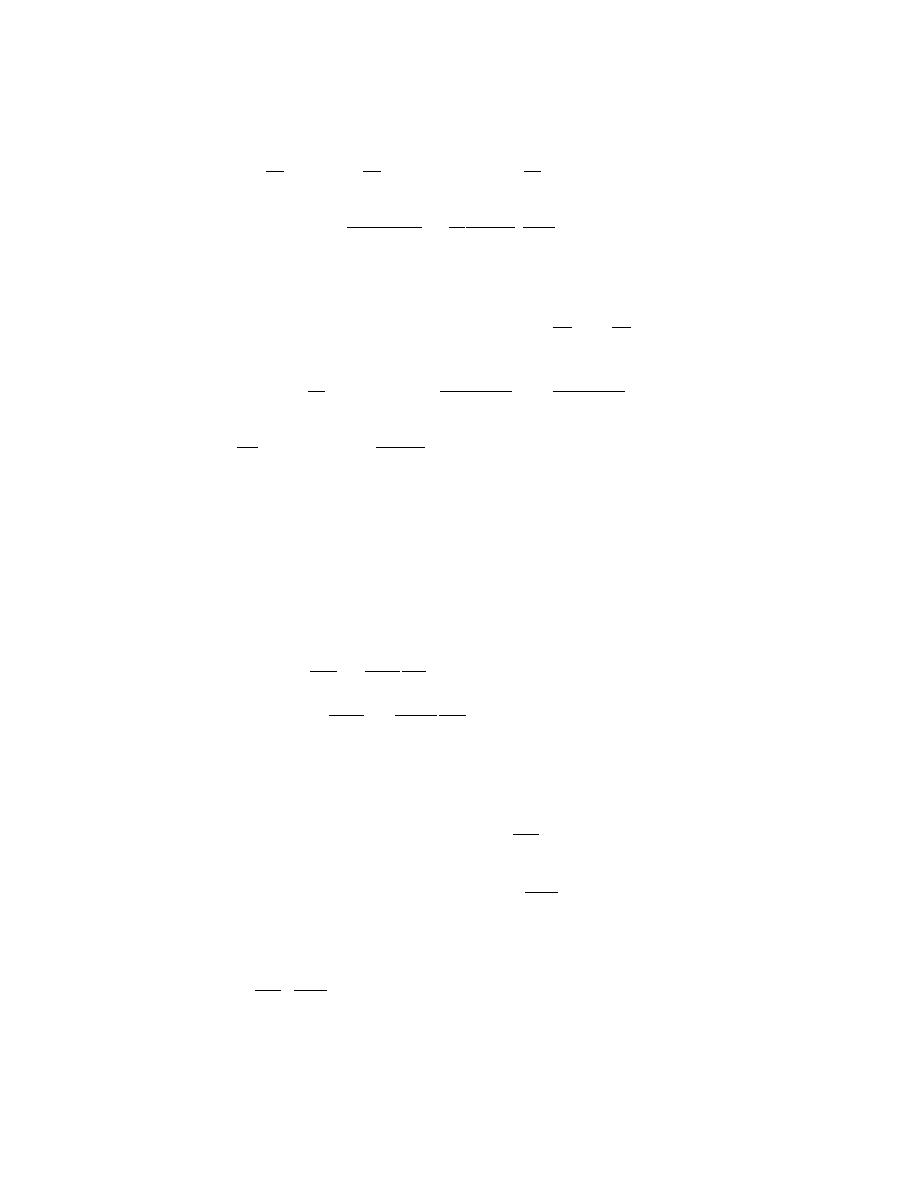
[
]
() ()
x2
t2
(ud)t 2 - (ud)t1
dx + ∫ u2d
- u2d dt
∫
t1
x1
x2
x1
t2 2
d2
t2 x2
d + ds η
d
+ dsi η dt - g ∫ ∫ ηsi
-
+g ∫
dxdt
i
2
x
x
2
x
t1
t1 x1
1
(101)
2
fbu2 (B + 2d)
u - υ
2
t2 x2
t2 x2
fi
B
- g ∫ ∫ dSodxdt + ∫ ∫
dxdt = 0.
1 +
fb (B + 2d) u
8B
t1 x1
t1 x1
Conservation of ice momentum is
t2 2
η2
[(vη)
]
() ()
x2
t2
η
- v η dt + gsi ∫ dt
- (vη)t
dx + ∫ v2η
2
∫
t1 2 x
2 x
t2
t1
x1
x2
1
x1
1
2
t2 K (1 p)η2
Kp (1 p)η2
t2 x2
d
p
g ∫ ∫ η So dxdt + g(1 si ) ∫
-
dt
t1 x1
x
2
2
x
x
t1
(102)
1
2
g(1 si ) t2 x2
1 t2 x2
∫ ∫ k0λKp (1 p)η dxdt = 0 .
fi (u v) dxdt +
2
2
-
∫∫
8si t1 x1
B
t1 x1
Discretization of the system of equations
The equations are transformed from their integral form into a differential form
to facilitate the discretization needed to proceed with numerically simulating jams.
By assuming the dependent variables to be continuous, differentiable functions
enables their expansion as Taylor series. The terms in the conservation of water
mass equation become thereby
(d) ∆t +
(d) ∆t2 + ...
2
(d)t = (d)t +
t2
2
t
2
1
(ud) ∆x +
(ud) ∆x2 + ....
2
(103)
(ud)x2 = (ud)x1 +
x2
2
x
Then, by retaining only the first-order terms and assuming that the increments ∆x
and ∆t approach zero
[(d)
]
(d) dtdx
x2
x2 t2
- (d)t dx = ∫ ∫
∫
lim
t2 → t1
t2
t
1
x1
x1 t1
[
]
(ud) dxdt.
t2
t2 x2
∫ (ud)x2 - (ud)x1 dt = ∫ ∫
lim
x2 → x1
(104)
x
t1
t1 x1
Consequently, the conservation of water mass equation reduces to
(d) +
(ud) dxdt = 0.
t2 x2
∫∫
(105)
t1 x1 t
x
If this equation is to hold everywhere in the (x,t) plane, then it will hold for an
infinitely small volume, such that the eq 105 can be rewritten as
42



 Previous Page
Previous Page
