equal to the change in ice storage over the time interval and considering ρ as con-
stant results in
t2
x2
∫ (υAi si )x - (υAi si )x dt + ∫ (Ai si )t - (Ai si )t dx = 0 .
1
1
(37)
t
x
2
2
1
1
Further simplifications are made subsequently, such as expressing Ai in terms of
jam thickness η(x,t) .
Conservation of water momentum
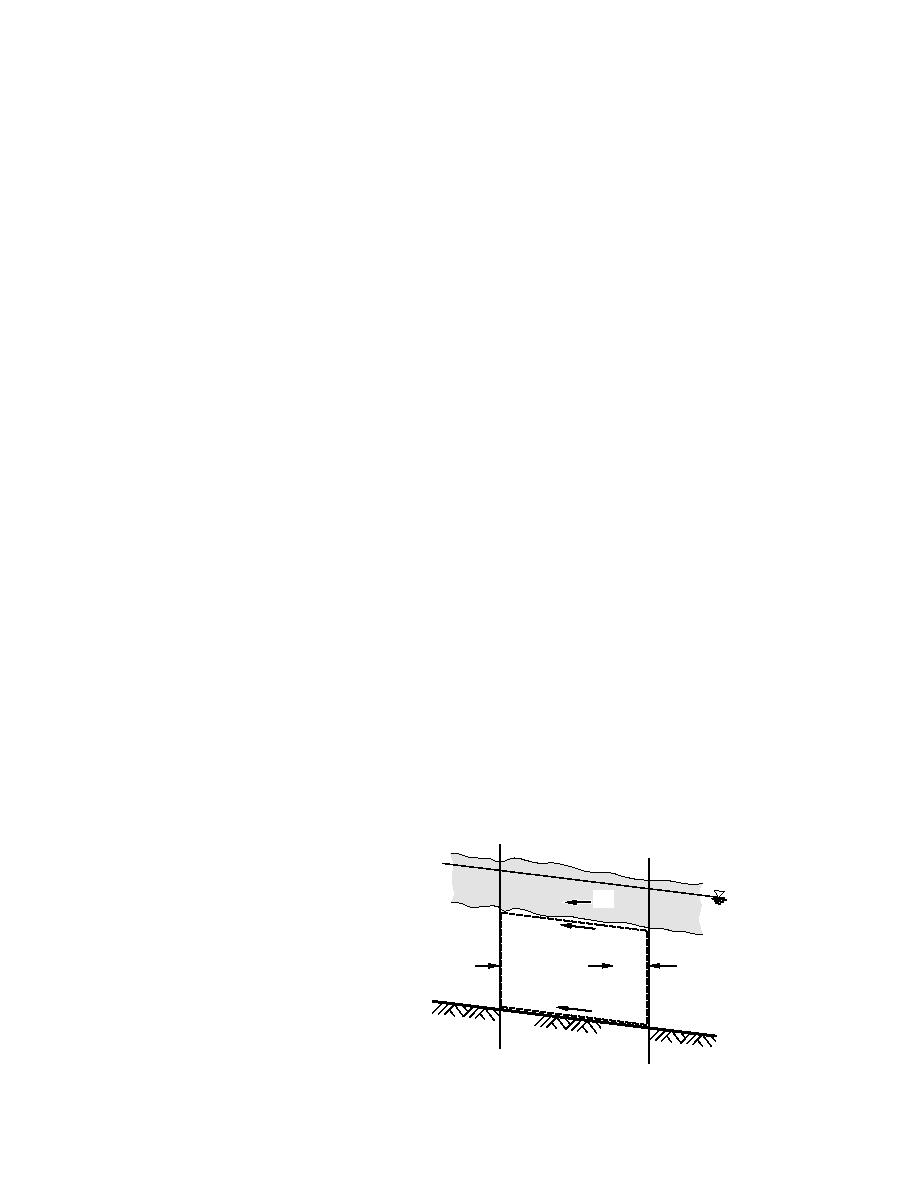
The analysis examines the control volume for water flow beneath an ice jam
whose channel cross section is prismatic. Figure 25 depicts the forces acting on the
control volume that is bounded by x1, x2, and the bed and the bottom of the jam.
Conservation of momentum in the x-direction requires that the change of
momentum within the control volume between times t1 and t2 equal the sum of the
net flux of momentum into the control volume and the integral of the external
forces acting on the control volume during the same period. The momentum inside
the control volume at any instant is
x2
∫ (ρAu) dx
(38)
x1
so the net increase in momentum ∆M between times t1 and t2 is
[(ρAu)
]
x2
- (ρAu)t dx .
∆M = ∫
(39)
t2
1
x1
The net momentum flux Mf into the control volume between times t1 and t2 is
(
) - (ρAu )
t2
Mf = ∫ ρAu2
2
dt .
x2
(40)
t1
x1
The external forces acting on the water control volume include: hydrostatic pres-
sure; gravity forces due to the weight of the water, ice, and pore water; and shear
stress at the bed, banks, and jam underside. The hydrostatic pressure forces acting
at sections x1 and x2 are and as depicted in Figure 25. With the level of the phreatic
surface above the bed denoted as D(x), the vertical distance above the bed as δ(x),
and the local width as b(δ), for any section x
Fg2
Figure 25. Forces acting on the
Ffi
water control volume.
Fp1''
Fg1
Fp1'
Ffb
x1
x2
32





 Previous Page
Previous Page
