6. Acknowledgments

[7] S. Bhukhanwala and T. V. Ramabadran. Automated global

enhancement of digitized photographs. IE E Transactions

E

on Consumer Electronics, 40(1):272 278, February 1994.

The ERS-1 SAR images and associated tracked ice mo-

[8] M. Black and P. Anandan. A framework for the robust es-

tion vectors were provided courtesy of Mark Drinkwater to

timation of optical flow. In Proceedings of IE E Computer

E

advance the rendering of sea-ice motion products of Antarc-

Society International Conference on Computer Vision, pages

tic sea ice as started under NSF OPP-9818645. ERS-1 scat-

231236, 1993.

terometer images were originally processed by David Long

[9] M. J. Black. Robust incremental optical flow. PhD thesis,

of Brigham Young University as part of the collaborative

Yale University, 1992.

ESA-supported AO2.USA.119 project:SAR data were also

[10] R. N. Bracewell, K.-Y. Chang, A. K. Jha, and Y.-H. Wang.

supplied courtesy of ESA, 1992, and processed to ice mo-

Affine Theorem for two-dimensional Fourier Transform.

tion under the same study. Further development of a mo-

Electronics Letters, 29(3):304, February 1993.

[11] P. J. Burt and E. H. Adelson. The laplacian pyramid as a

tion tracking algorithm was made possible through ONR

compact image code. IE E Transactions on Communica-

E

N00014-03-1-0045 and N00014-02-1-0244.

tions, COM-31,4:532540, 1983.

[12] E. D. Castro and C. Morandi. Registration of translated and

rotated images using finite fourier transform. IEEE Transac-

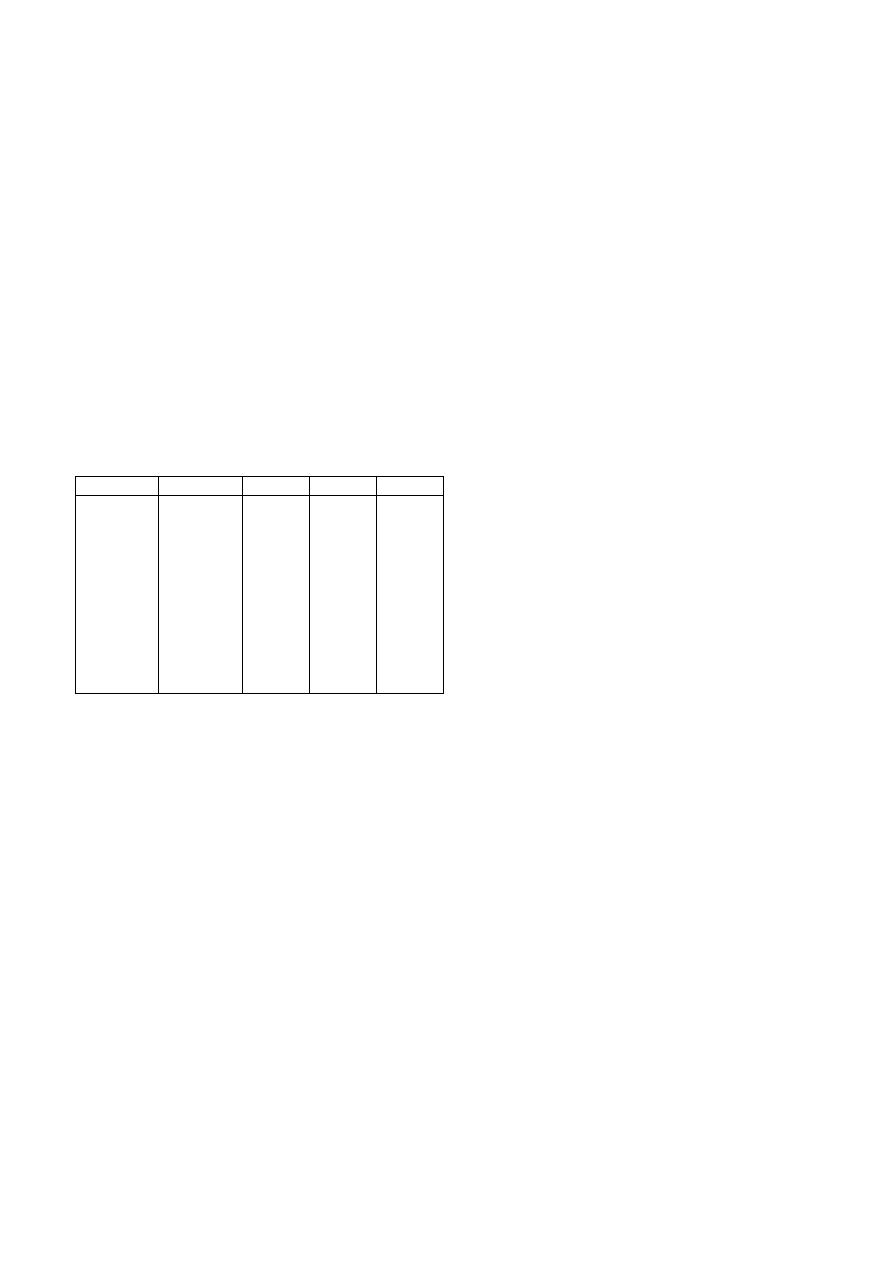
Table 1. Comparison of PSNR between