120
2000
Fire Shut-down
Bottom Depth (m)
100
1600
80
1200
Water Level (m)
60
800
Total Consumption (m3)
40
400
20
Pool Depth (m)
0
0
31 Dec 92
31 Dec 93
31 Dec 94
31 Dec 95
Figure A1. Measured SPWW dimensions and water consumption.
Assuming that the shape factor α is approximately constant as the well develops,
the change in pool volume becomes
dVp = 2αHRdR + αR2dH
(A6)
Inserting eq A6 into eq A4 yields
)(
)
(
dQ = γ pπR2dL 1 γ p 2αHRdR + αR2dH .
(A7)
Because water consumption, water levels, and pool depths were measured, we
may use eq A7 to determine pool radius throughout well development. Note that
the specific gravity of ice increases approximately linearly with depth over the pool
depths of interest (Kuivinen et al. 1982):
(
)
γ p = 0.68 + 0.0028 Dp 60 ,
(A8)
where Dp is the average pool depth in meters.
Measurement uncertainties in dL and dH and slight timing differences between
these measurements and those for dQ can yield unrealistic values of R if eq A7 is
L
R
dR
Initial Water Surface
dL
Final Water Surface
H
D
Initial Pool Shape
Final Pool Shape
dD
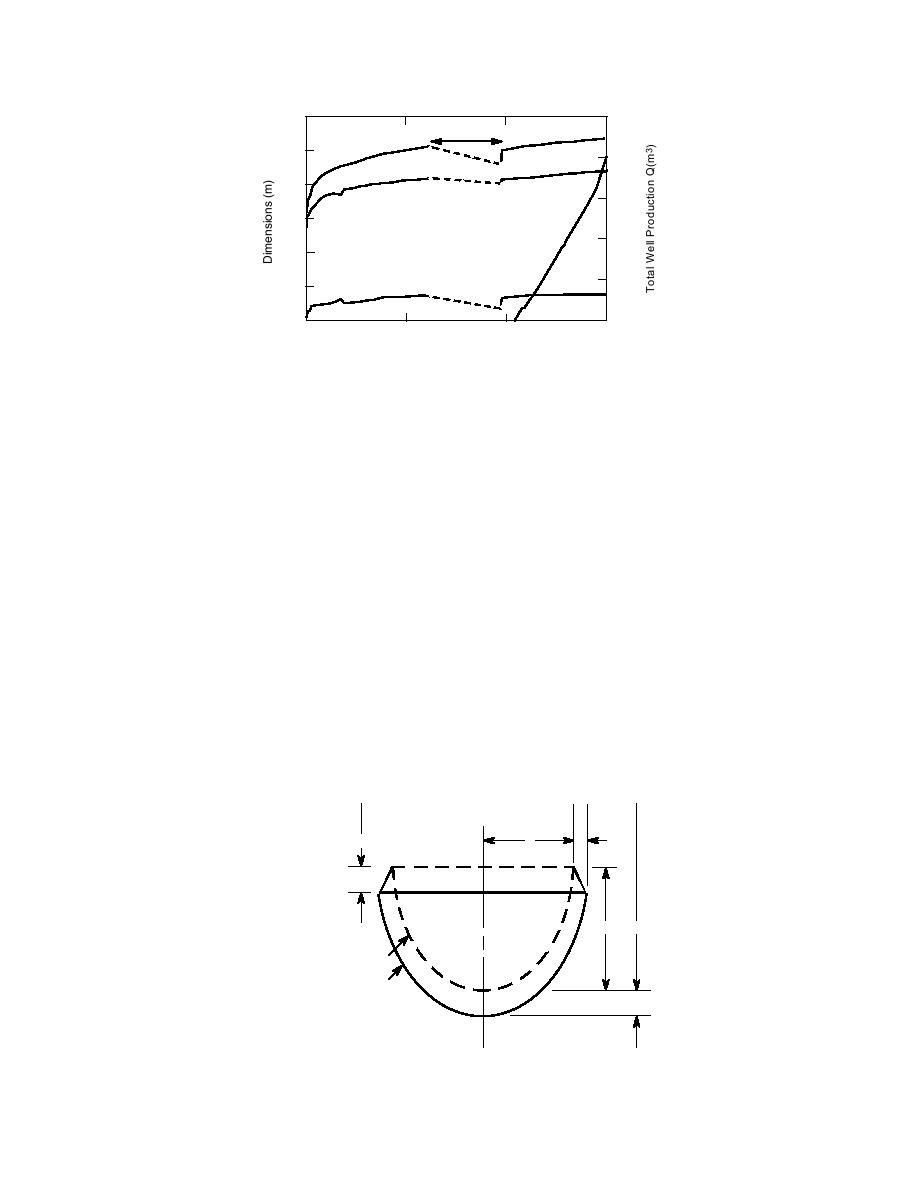
Figure A2. Schematic of well pool, showing changes in dimensions as the pool deepens.
27



 Previous Page
Previous Page
