of measurements of the modulus of elasticity as a
1010
function of temperature for sealant materials, and
a.
in recognition of the incompatibility of such data
10 9
with movement capability-based design calcula-
tions, this paper presents a review of simple engi-
10 8
neering mechanics-based analysis techniques for
the structural design of rubber materials subjected
10 7
to tension, compression, and shear loading in long
rectangular joint seal configurations.
10 6
Solutions and techniques described by Rivlin
and Saunders (1949), Payne (1956), Gent and
Lindley (1958, 1959), Gent (1974), and Gent et al.
10 5
100 80 60 40 20
0
20
40
60
80
100
(1974), for rubber materials that can be consid-
1010
ered incompressible under hydrostatic loading,
are summarized here and in some cases extended
b.
10 9
to the plane strain configuration of a joint seal.
Previous reviews of these solutions, and of the
10 8
corresponding engineering practice in general,
have been presented by Payne and Scott (1960),
10 7
Lindley (1967), Gent and Meinecke (1970), Gent
(1978a, b), and Stanton and Roeder (1982). Recent
10 6
examinations and extensions of these solutions
have been presented by Chaloub and Kelly (1991).
10 5
A design example illustrating use of solutions
10 4
from Payne (1956) and Gent and Lindley (1959) is
100 80 60 40 20
0 20 40 60
80 100 120 140
Temperature (C)
shown in Appendix A.
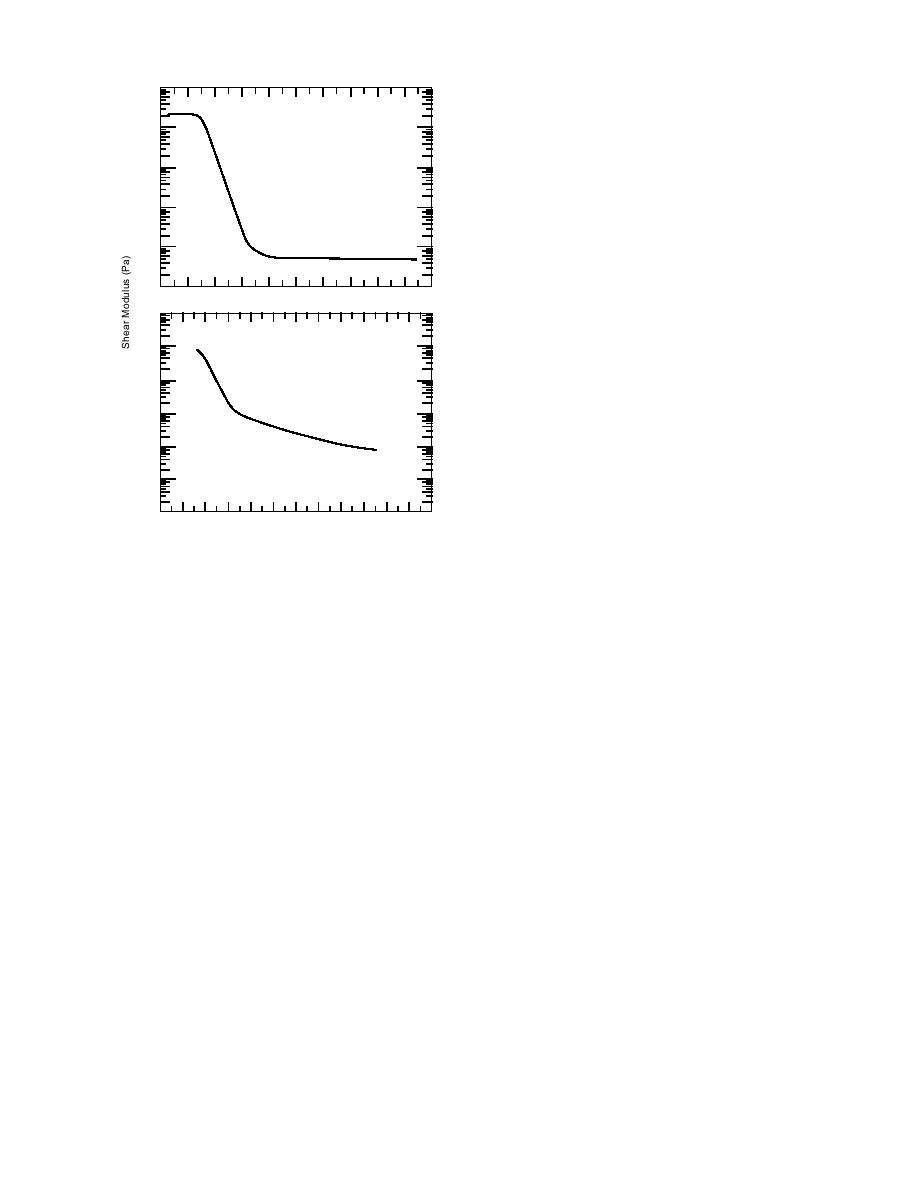
Figure 1. Real part of shear modulus G as a function
of temperature T for (a) a natural rubber and (b) a
EXTENSION AND
polysulfide sealant, from 50-Hz harmonic loading
COMPRESSION LOADING
tests (Nashif and Lewis 1991).
Gent and Lindley (1959), using small deforma-
tion, linear elasticity theory and realistic assump-
could make similar suggestions about the rubber
tions regarding structural deformations, gener-
subjected to slower loads from modulus vs. tem-
ated solutions for the stress distribution in bonded
perature results of quasi-static loading tests, which
rubber blocks during compression of the blocks.
are also conventional thermal analysis tests.
From these solutions they obtained expressions
Lewandowski et al. (1992) have demonstrated the
for the nominal stressstrain relations of the
measurement and usefulness of such data for
blocks. Payne (1956) and Gent and Lindley (1959)
pavement sealants.
also suggested approximate relations for the cor-
It is easy to envision the potential practicality
responding large deformation problem. These and
of such information for the selection of cold cli-
other solutions described here for a block in a
mate joint sealants. For example, a designer could
plane strain configuration are directly applicable
use modulus vs. temperature data of the candi-
to the extension and compression of long, formed-
date sealants together with climatic temperature
in-place, rectangular butt joint seals, and can be
data for the region of interest, and make a selec-
presented as such with only slight modification
tion using a rationale that incorporates a severe
of terminology.
winter design temperature. This is in contrast to
current practice, which typically utilizes standard
Shape factor, modulus
bond tests of model seal structures at a given low
of elasticity, and
temperature (e.g., ASTM 1991c, d), but which does
apparent modulus
not, in general, reveal explicitly the temperature
The apparent modulus of a butt joint seal in
range at which the model seal or the sealant ma-
extension or compression was given by Gent and
terial stiffens. In recognition of the potential uses
Lindley as
2



 Previous Page
Previous Page
