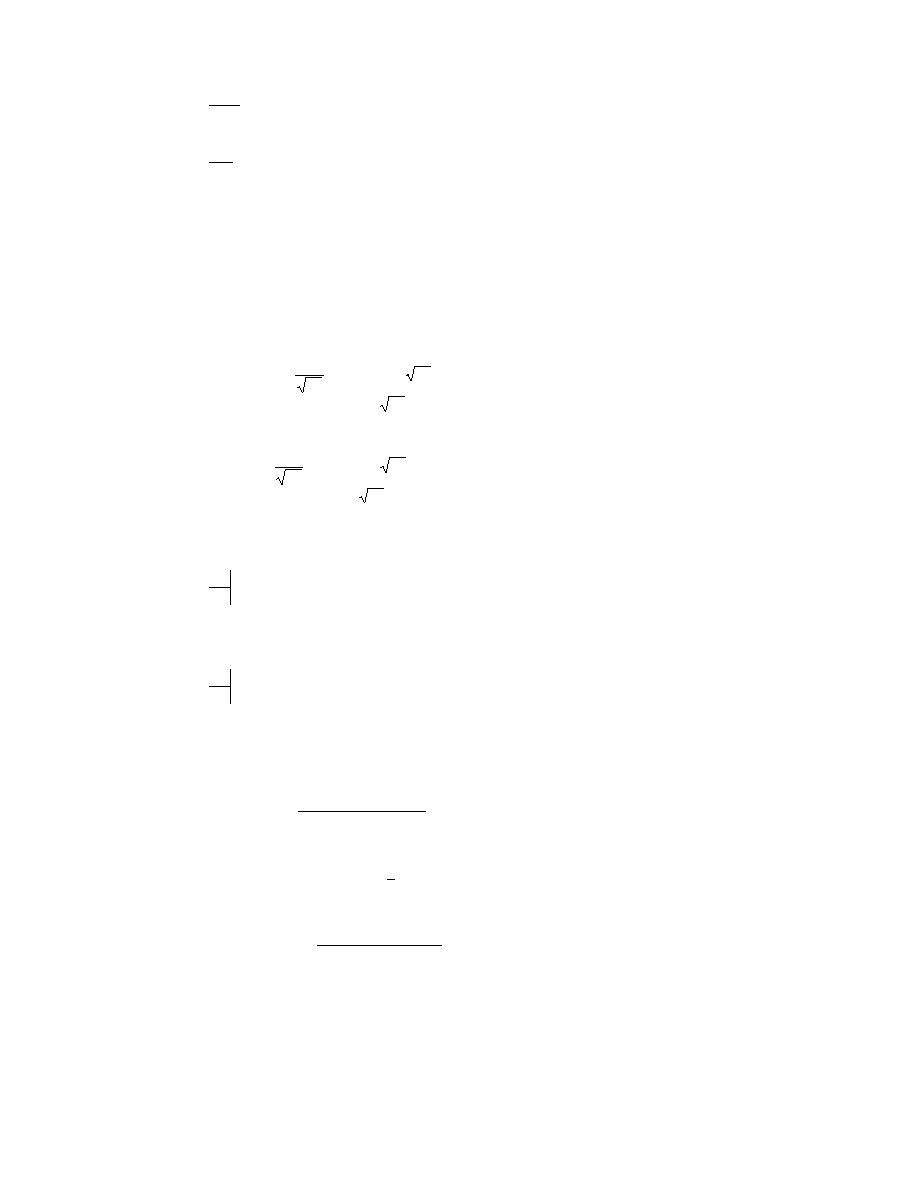
d2θ*
- θ* (∆* )-1/ 4 = 0
(47)
dξ
2
d∆*
- 8(θ* - ∆* ) ξ-1 = 0
(48)
dξ
with the boundary conditions
ξ = 0, θ* = 1; ξ = ∞, θ* = ∆* = 0.
(49a,b)
Patankar and Sparrow (1979) observed that an analytical solution of eq 4749 was not
possible, but Wilkins (1980) showed that an analytical solution does exist and can be
written as
7
ξ
1-
, 0 ≤ ξ ≤ 42
θ = θ* =
(50)
42
0
, ξ > 42
8
ξ
1-
, 0 ≤ ξ ≤ 42
∆* =
(51)
42
0
, ξ > 42
Using eq 50, the temperature gradient
θ
X X =0
can be found. Integrating
θ
X X =0
from Z = 0 to Z = Z, the heat conducted into the base of the fin over a distance Z can be
evaluated. The final result is
g ρl (ρl - ρv ) k 3hfg L7
Z 7/8 .
q (Z) = 4.9371
(52)
l
lkf
3w3
The ideal heat transfer qideal (Z) can be found by assuming the entire fin to be isother-
mal at temperature Tb and using h from eq 34. This gives
gρl (ρl - ρv ) k 3hfgL7
qideal (Z) = 5.333
Z 3/ 4 .
(53)
l
lkf
3w3
The fin efficiency η expressed as the ratio of q(Z)/qideal(Z) is found to be
η = 0.9257 Z 1/8 .
(54)
14





 Previous Page
Previous Page
