y
x
z
Tsat
Tfb
H
kf
L
w
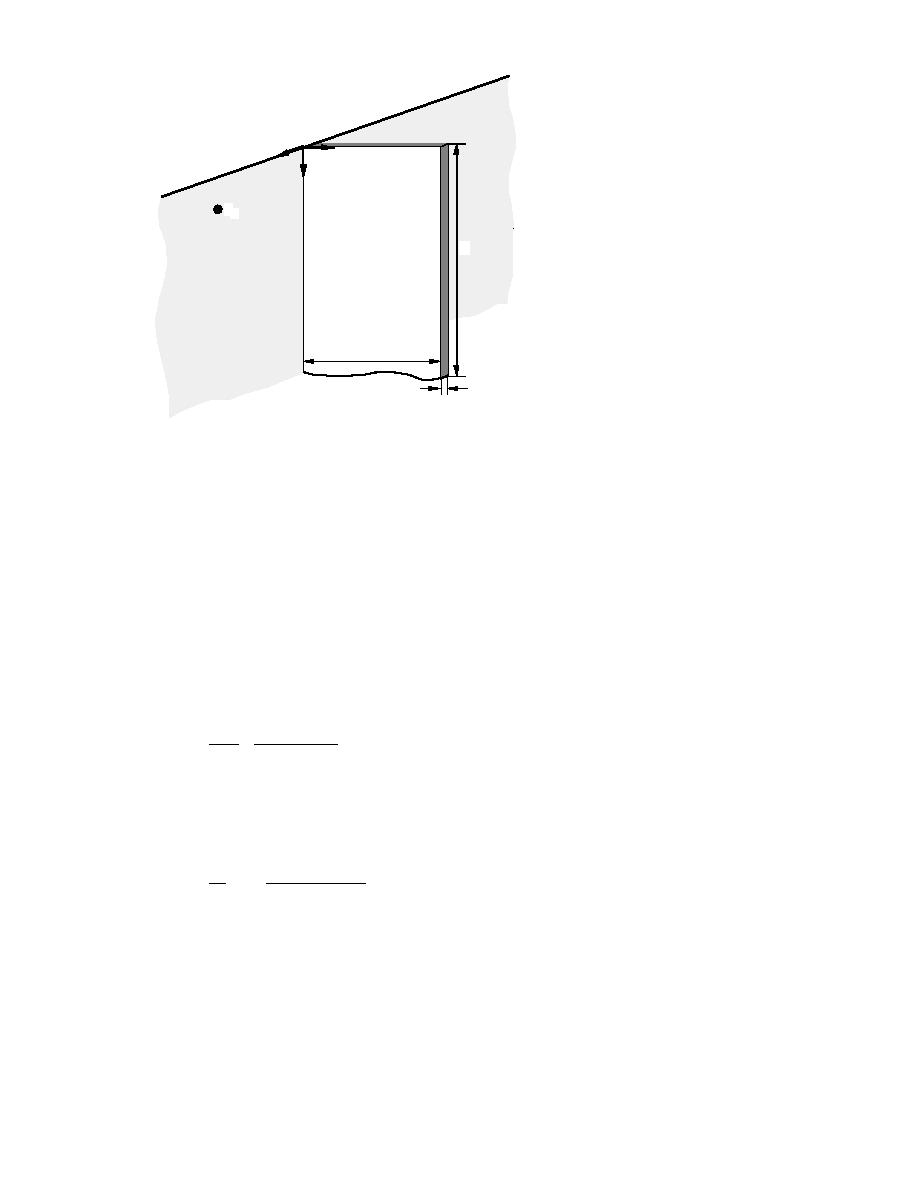
Figure 7. Two-dimensional vertical fin of
rectangular profile.
The analysis assumes the fin temperature Tf and the film thickness δ to be functions of
x and z; that is, Tf = Tf (x,z) and δ = δ (x,z). These assumptions can be justified as follows.
As the condensate film flows downward along the fin, more condensate is added to it, and
its thickness increases along z to accommodate the increased flow rate. Along the x
direction, the temperature differential, Tsat Tf, decreases from the base (x = 0) of the fin to
the tip (x = L) of the fin. Consequently, δ also decreases along the x direction. Thus the
physics of the process dictates that δ is a function of x and z. The effect of the growth of δ
with z is to increase the thermal resistance of the film, thereby decreasing the heat conduc-
tion into the fin. The decreased heat flow implies that the fin temperature at a given x
must decrease along the z direction. Thus the temperature distribution in the fin is also
two-dimensional; that is, Tf = Tf (x,z).
Considering a fin element of dimensions dx, dy and w and making an energy balance
gives
2kl (Tf - Tsat )
2T
=
f
.
(35)
kf wδ
2
x
Equation 35 assumes that in the fin, conduction in the x direction is dominant, while in the
film, conduction in the y direction is dominant. The momentum equation for the z direc-
tion takes the form
4 lkl (Tsat - Tf )
(δ 4 ) =
.
(36)
gρl (ρl - ρv ) hfg
z
Equations 35 and 36 constitute two coupled partial differential equations for Tf (x,z)
and δ (x,z).
For convenience, the following dimensionless quantities are introduced into eq 35
and 36:
θ = (Tf - Tsat )/(Tfb - Tsat ) , X = x / L
12





 Previous Page
Previous Page
