other functions (properties) of a state. The compos-
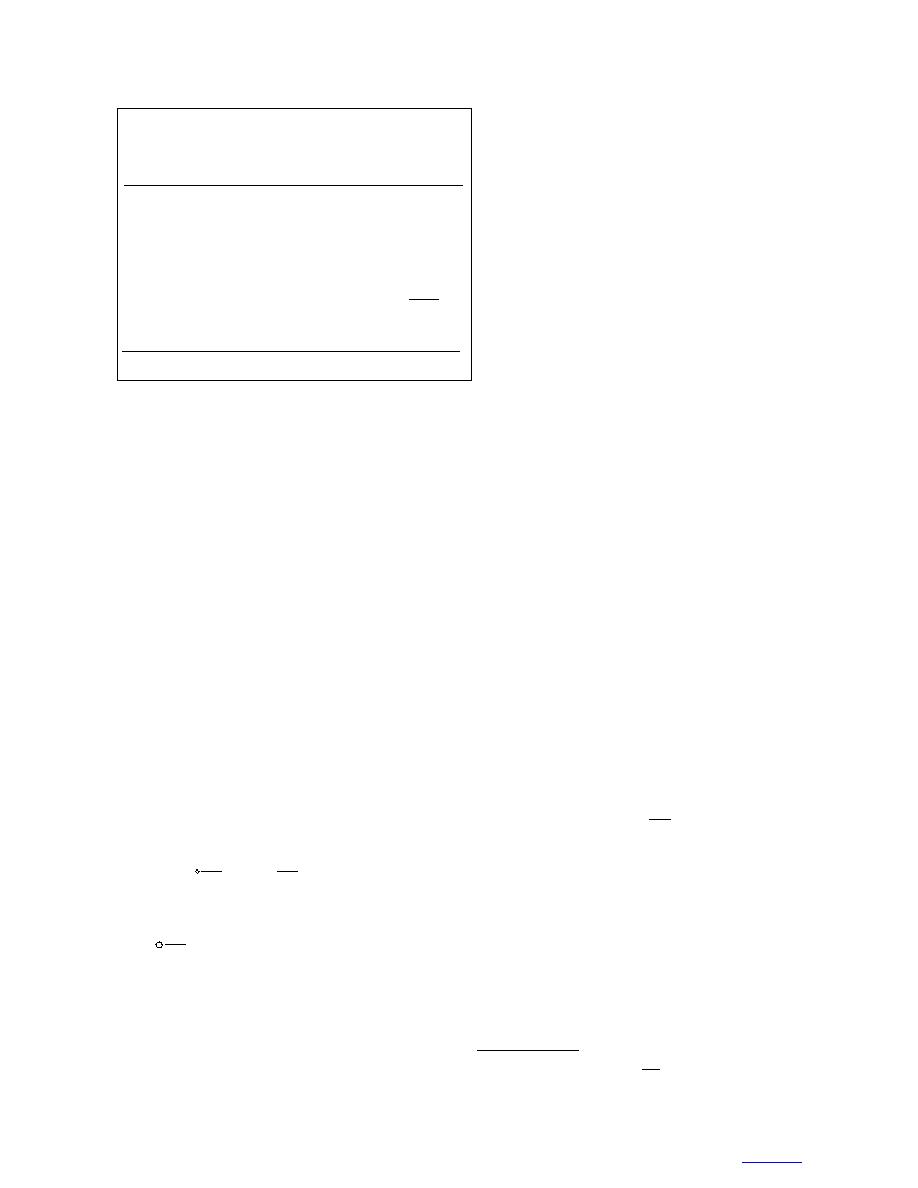
Table 1. Types of reversible work done by thermo-
ite functions are Gibbs free energy, enthalpy, and
dynamic systems (Silver and Nydahl 1977).
Helmholtz free energy. They were defined for con-
Generalized
Generalized
Element
venience from applying the first and second laws to
Type of work
force
displacement
of work
systems under various constraints. For example,
P
V
PdV
Volumetric
Gibbs free energy (a quantity of great interest in study-
ing freezing soils) was developed to help study sys-
F
l
Fdl
Length
tems that exist at constant temperature and pressure.
ψ (surface tension)
ψdAr
Ar (area)
Surface
Gibbs free energy, G: For constant temperature and
gz
m (mass)
gzdm
Gravitational
pressure, d(PV) = PdV and d(TS) = TdS. Applying
r 2ω 2
the first law in the form δQ = dU + δW and the rela-
r2ω2/2
dm
m (mass)
Centrifugal
tion that TdS ≥ δQ* (from eq 2a), we obtain TdS ≥
2
ε
εdq
q
Electrical
dU + δW, where δW = PdV + δWa and Wa is all of the
η
dη
work other than PV work. Thus, d(U + PV TS) ≥
Chemical*
δWa, and G ≡ U + PV TS becomes a convenient
* = chemical potential (see eq 18); η = number of moles.
Heat capacity, C, is the amount of heat, δQ, that
δWa < 0 for a spontaneous transformation to occur at con-
must be added to a system to change the temperature
stant temperature and pressure. This will be discussed
by dT, or C = δQ/dT. Cv is the heat capacity at constant
again in the section "Thermodynamic equilibrium."
Enthalpy, H: H ≡ U + PV ≡ G + TS. Enthalpy applies
volume, and Cp is the heat capacity at constant pres-
sure.
to systems at constant pressure, such as laboratory sys-
The fundamental thermodynamic principles needed
tems at atmospheric pressure. It was developed simi-
for the study of freezing soils are
larly to Gibbs free energy by applying the first law at
The zeroth: If two systems are in thermal equilibri-
constant pressure.
Helmholtz free energy, A: A ≡ U TS ≡ G PV. Helm-
um with a third, then they are in equilibrium with each
other.
holtz free energy was developed for constant tempera-
Conservation of mass: Matter is not created or
ture systems.
destroyed; it can only be changed to other chemical
species or to energy.
Fundamental equations
First law: Energy is conserved. A mathematical state-
The basic balance equations of thermodynamics
ment of the first law is
relate the heat and work transferred during a process to
a difference in thermodynamic functions such as
dU = δQ δW, or ∆U = Q W
(1)
enthalpy and entropy. For a closed system, the energy
balance equation is eq 1. Making substitutions for the
where U is the energy of the system.
heat term (see the definition of entropy) and for the
Second law: Every system that is left to itself will
work term (from Table 1) yields
change toward a condition in which its ability to do
dU = TdS - PdV + Fdl + ψdAr + εdq
work will have decreased. Another way to express the
second law is that entropy can be produced, but never
ω2
+ ∑ i dηi + gzdm + r 2
dm+, etc. ...
destroyed. A mathematical statement of the second law
(3a)
2
is
δQ
δQ
Equation 3a is known as the property relationship (Sil-
∫ dS > ∫
, or dS >
(2a)
.
ver and Nydahl 1977). For the engineering study of
T
T
thermodynamics, this relationship is often stated for
For all irreversible cycles
systems in which there is only expansive work:
δQ
∫ T <0
(2b)
dU = TdS PdV.
(3b)
However, for the study of freezing soil, the property
and for any change of state in an isolated system
relationship often used is
dS > 0.
(2c)
dU = TdS - PdV + ∑ i dηi .
(3c)
Other important definitions include the composite
δQ
* In a reversible process, dS =
functions, so called because they are combinations of
.
T
3





 Previous Page
Previous Page
