EXAMPLE MATHCAD 6.0 WORKSHEET FOR GIROUD AND
NOIRAY DESIGN METHOD
This is a file to calculate the aggregate depth needed for different K
values of the geotextile. It uses eq 43, 33, 35, 36, and 37 (a' > a, mean-
ing that the parabola between wheels is wider than the sum of the widths
of the parabolas under the wheels), 30 and 31 as well as 5 and 7 (on-
highway trucks). The original reference is Giroud and Noiray (1981).
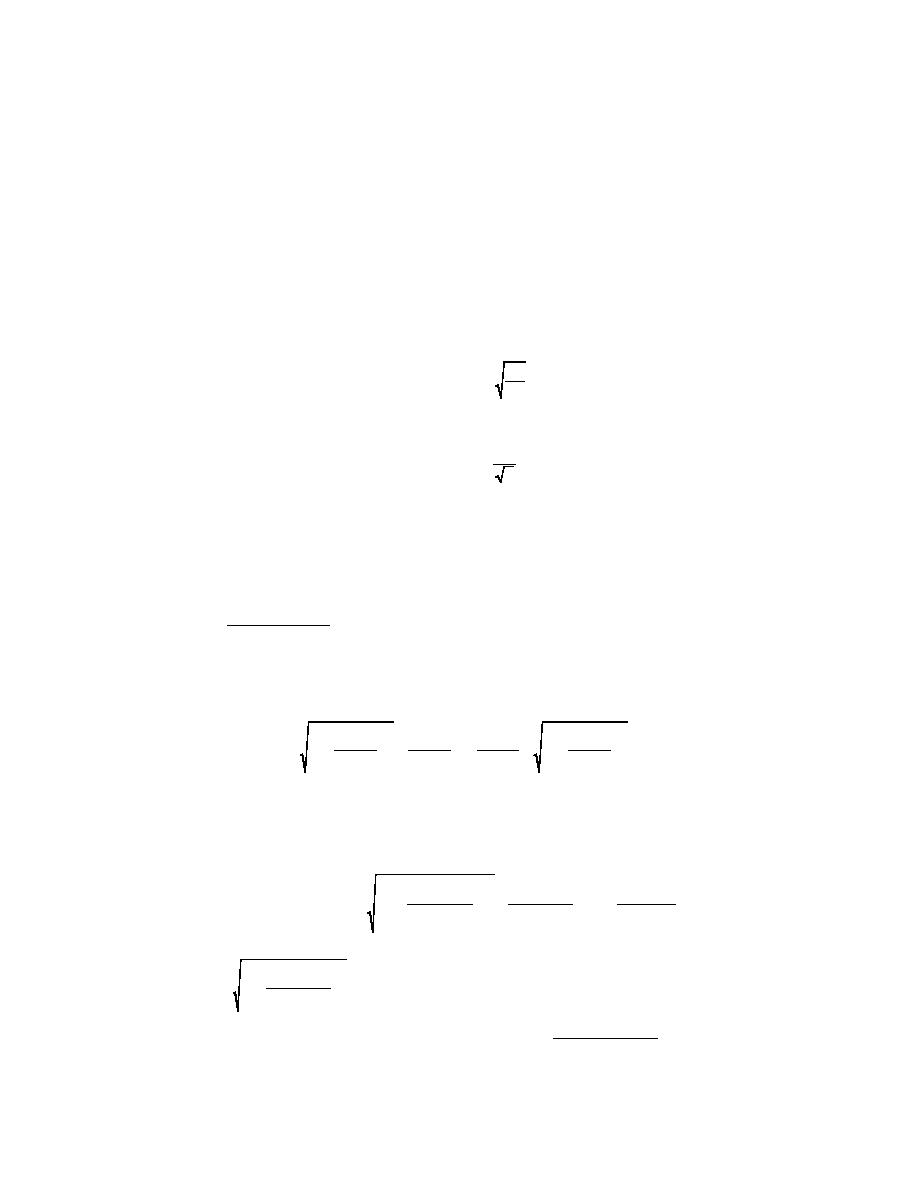
K = 200 000 N m1
e = 2.0 m
tan α = 0.6
P = 230 000 newton
Pc = 414 000 P
r = 0.3 m
P
B=
Width of wheel load (on road), 5:
B = 0.745 m
Pc
h = 0, 0.1 ... 1.0 m
B
L=
Length of wheel load(on road), 7:
L = 0.527 m
2
Width of parabola under wheel, 30:
Width of parabola between wheels, 31:
a(h) = 0.5 (B + 2 h tan α)
aprime(h) = 0.5(e B 2 h tan α)
Settlement of geotextile from original position, 33:
r ⋅ aprime(h)
s(h) =
a(h) + aprime(h)
Equation for half length of parabola under the wheel, 36:
2
2
2 ⋅ s(h)
a(h)
2 ⋅ s(h)
2 ⋅ s(h)
b(h) = a(h)1+ 0.5 1+
+
⋅ ln
+ 1+
2
a(h)
2 ⋅ s(h)
a(h)
Equation for half length of parabola between the wheels, 37:
2
[
]
2 ⋅ r s(h)
aprime(h)
r s(h)
⋅ ln 2 ⋅
bprime(h) = aprime(h) ⋅ 1+ 0.5 1+
+
+
[
]
aprime(h)
aprime(h)
2 ⋅ r s(h)
2
[
]
2 ⋅ r s(h)
2
+ 1+
aprime(h)
b(h) + bprime(h)
e(h) =
1
The elongation of the geotextile, 35:
a(h) + aprime(h)
27



 Previous Page
Previous Page
