2
layered system is used to refer to the set of 7 (k10 )5 (lb/0n. )
Pa
i
1 5
x
stresses, strains, and displacements occurring
at that point for a particular loading condition.
A set of layer moduli (Mr1,..., Mrn) is said to be
compatible at radius r with a given set of non-
linear models ( f1,...,fn) if each Mr agrees
(within a specified tolerance) with the modu-
lus determined by the model fi evaluated at
the middepth of layer i and at radius r.
1 σ3 (lb/in. )
2
The fundamental assumption of NELAPAV
2
may be stated as follows: "The state of a point
w = 16.1%
4
γ d = 105 lb/ft3
7
p = (r,z) in a layered system is primarily deter-
7 x 10 4
104
20 (lb/in.2 )
mined by the modulus of elasticity of each of
0
10
the layers in the system at the radius, r, of the
0
70
140 (kPa)
Deviator Stress
point." Once this assumption has been made,
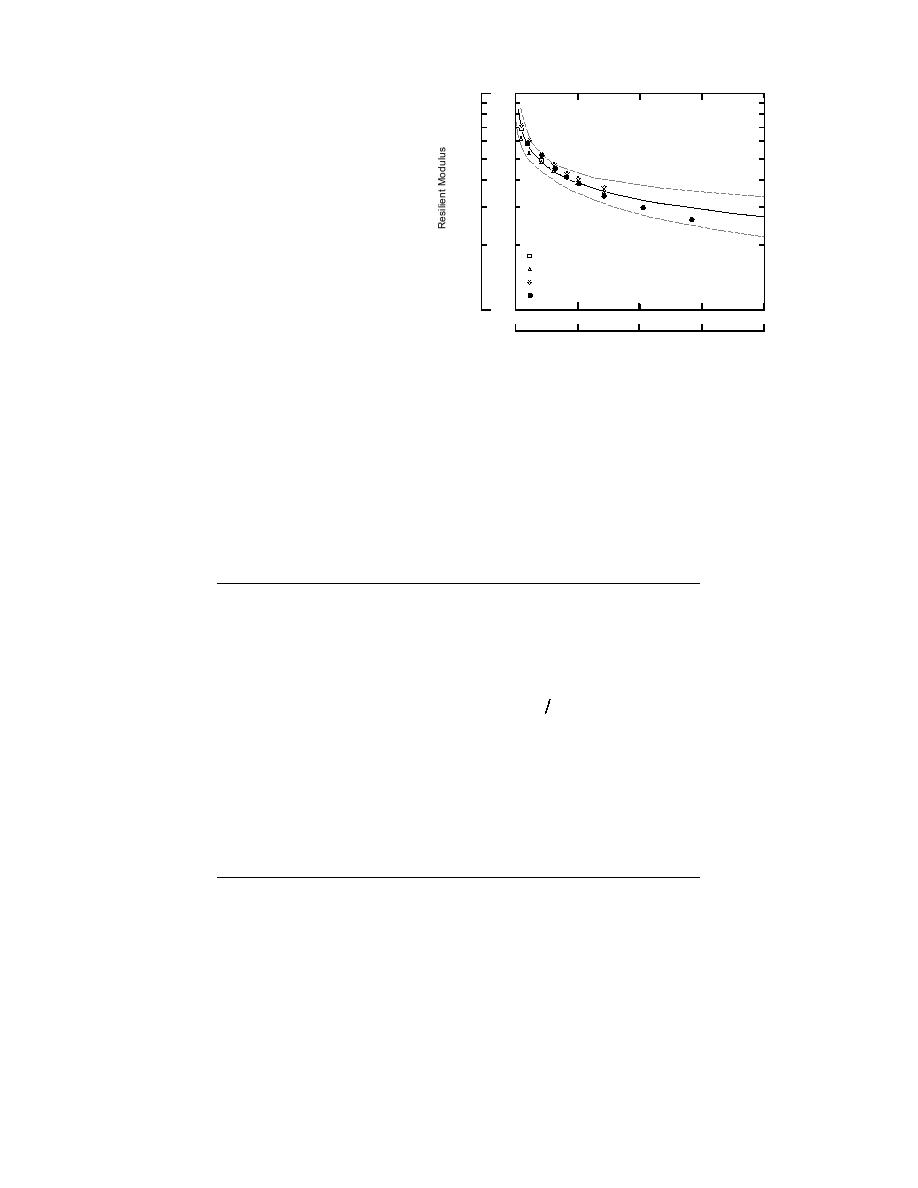
the state of any point in the layered system Figure 5. Stress dependence of resilient modulus in 1206
may be determined by computing a set of com- clay subgrade material (from Berg et al. 1996).
patible moduli at the radius of the point and
then evaluating the resulting layered system at the
routines iteratively in order to come up with a set
point, holding the moduli constant.
of compatible moduli for the layered system at the
NELAPAV operates as a "front-end" to
radius of each of the desired calculation points.
CHEVLAY in that it calls the basic CHEVLAY
The tolerances for compatibility used in this study
Table 4. Models currently available in NELAPAV (from Yang 1988).
Model
no.
Name
Specification
Mr = constant
0
Linear
Mr = k1θk2
1
Bulk stress
k2 + k3 (k1 - σd ) σd < k1
Mr =
2
Deviator stress
k2 + k4 (σd - k1) σd ≥ k1
Mr = k1( J2 τoct )k2
3
Second stress invariant
Mr = k1τoct k2
4
Octahedral shear stress
Mr = k1σ vk2
5
Vertical stress
Mr = k1σ1k2
6
Major principal stress
Mr = k1( J12 + J12 )k2 (1 + τoct )k3 kck4
7
First stress invariant
0
p
octahedral shear stress
and anisotropic
consolidation ratio
Notes:
θ = bulk stress
k1, k2, k3, k4 =
constants
σd =
deviator stress
σv =
vertical stress
σ1 =
major principal stress
J10 =
first stress invariant due to overburden only
J1p =
first stress invariant due to overburden and load
kc =
anisotropic consolidation ratio
10



 Previous Page
Previous Page
