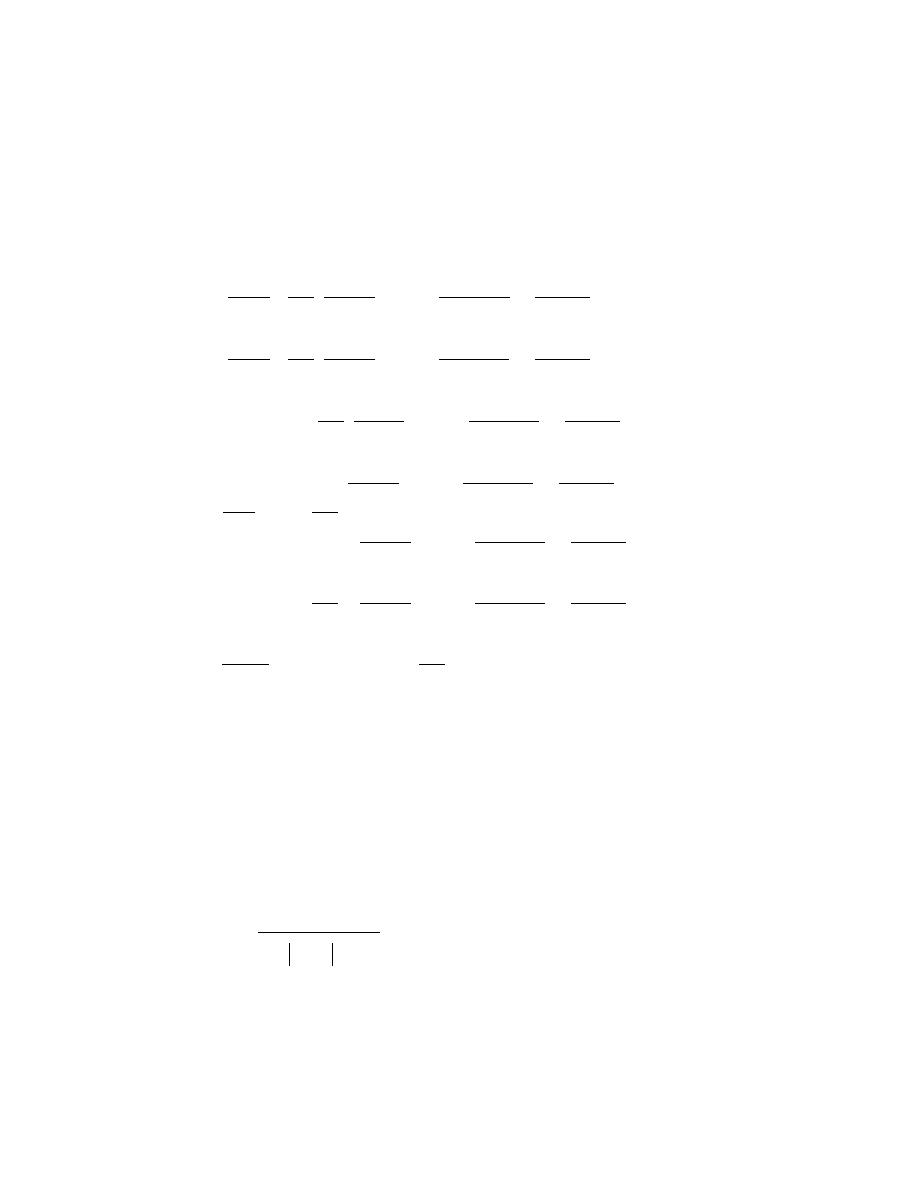
⎤ ⎡∆Ψ j+1,1 ⎤ ⎡ B1 ⎤
⎡γ 1 a1+
⎢ -
⎥ ⎢∆Ψ
⎥⎢
B2 ⎥
⎢a2 γ 2
+
a2
⎥⎢
j+1,2 ⎥
⎢
⎥
⎢
⎥⎢
⎥=⎢
⎥
(5.8)
⎢
+ ⎥⎢
⎥⎢
⎥
an-1 ⎥ ⎢∆Ψ j+1,n-1 ⎥ ⎢ Bn-1 ⎥
an-1 γ n-1
-
⎢
⎢
γ n ⎥ ⎢∆Ψ j+1,n ⎥ ⎢ Bn ⎥
-
an
⎦ ⎣
⎦
⎦⎣
⎣
where
∆t ⎡ ∂Ki+1/ 2
Ψ i+1 - Ψ i ⎫ Ki+1/ 2 ⎤
⎧
∂Bi
⎨cos ϕ -
+
a =
=
⎬-
⎢
⎥
i
∂Ψi+1 ∆zi ⎢ ∂Ψ i+1
zi+1 - zi ⎭ zi+1 - zi ⎥
⎩
⎣
⎦
(5.9)
∆t ⎡ ∂Ki-1/ 2
Ψ - Ψ i-1 ⎫ Ki-1/ 2 ⎤
⎧
∂Bi
cos ϕ - i
ai- =
=
⎬+
⎢
⎥
⎨
∂Ψ i-1 ∆zi ⎢ ∂Ψ i-1
zi - zi-1 ⎭ zi - zi-1 ⎥
⎩
⎣
⎦
⎧ ∆t ⎡ ∂Ki+1/ 2 ⎧
Ψ i+1 - Ψ i ⎫ Ki+1/ 2 ⎤
⎨cos ϕ -
⎬+
i =1
⎪
⎢
⎥
∆zi ⎢ ∂Ψ i ⎩
zi+1 - zi ⎭ zi+1 - zi ⎥
⎪
⎣
⎦
⎪
⎡ ∂Ki+1/ 2 ⎧
Ψ - Ψ i ⎫ Ki+1/ 2 ⎤
⎪
cos ϕ - i+1
⎬+
⎢
⎥
⎨
⎪
∂Ψ i ⎩
zi+1 - zi ⎭ zi+1 - zi ⎥
∂B
⎪ ∆t ⎢
γ i = i = φi + ⎨
2 ≤ i ≤ n - 1 (5.10)
⎢
⎥
∂Ψ i
⎪ ∆zi ⎢ ∂Ki-1/ 2 ⎧
Ψ i - Ψi-1 ⎫ Ki-1/ 2 ⎥
⎨cos ϕ -
-
⎬+
⎪
⎢ ∂Ψ i-1 ⎩
zi - zi-1 ⎭ zi - zi-1 ⎥
⎣
⎦
⎪
⎪ ∆t ⎡ ∂K
Ψ i - Ψi-1 ⎫ Ki-1/ 2 ⎤
⎧
⎪
⎨cos ϕ -
⎢-
⎬+
i=n
i-1/ 2
⎥
⎪ ∆zi ⎢ ∂Ψ i-1 ⎩
zi - zi-1 ⎭ zi - zi-1 ⎥
⎣
⎦
⎩
∂θwj+1,i
∆t
( v j +1,i+1 - v j+1,i ) - sources(i) + losses(i) . (5.11)
φi =
, Bi = θ wj+1,i - θ wj ,i +
∂Ψ i
∆zi
To ensure numerical stability when solving Equation (5.8), ∆t is chosen such that
Ksat for the different USCS soil types are found in Table 5.1.1 located in the next section.
5.1.3 Hydraulic Parameters
The relationship between volumetric moisture content and pressure head is highly
nonlinear. Following the work of van Genuchten (1980) it is
θmax - θr
θw = θr +
(5.12)
(
)
nvG mvG
1 + αvG Ψ
where θr is the residual volumetric water content, θmax is the maximum volumetric water
content, αvG (cm1) is a constant related to the reciprocal of the bubbling pressure head, n
is a constant dependent on the distribution of pores, and mvG = 1 1/nvG. Default values
for θr, θmax, αvG, and nvG are found in Table 5.1.1. As can be seen in Figure 5.1, the
45





 Previous Page
Previous Page
