29
DESIGN OF ICE BOOMS
With the design loads determined, the cable tensions can be
computed. It should be noted that a sag ratio s is usually in the
range of 0.150.20. This range generally allows the midstream
anchor cables to be the same size as the main boom cables. With
lower sag ratios, the tension and, therefore the wire rope diameter,
may become prohibitively large. With higher sag ratios, the ten-
sion decreases, but the length of the wire rope increases. The
increased length may require additional boom units, which would
result in higher costs.
Assuming that the downstream deflection of the boom is 32 ft,
s would be:
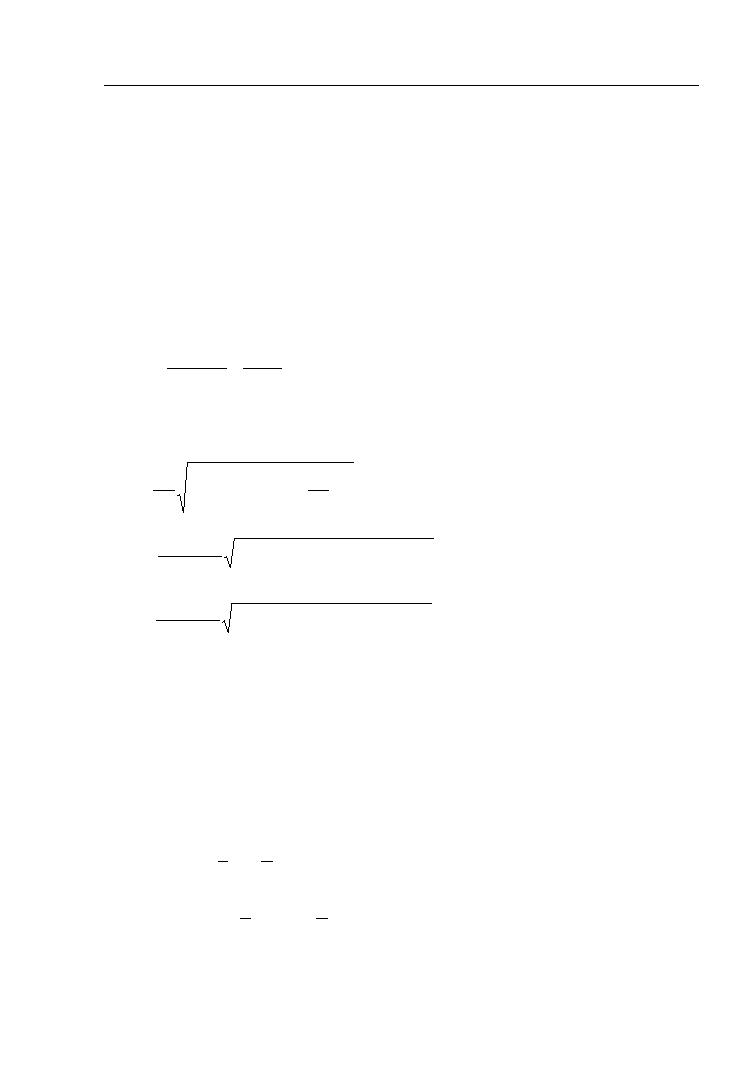
dmidspan
32 ft
s=
=
= 0.19
L
168 ft
The wire rope tension is then computed for both ends of the cable
using eq 9.
2
2x
1 + tan θ + 4s 1 -
pL
T=
L
8s
280 (168)
1 + [tan 26.5 + 4 (0.19) (1 0)] = 49, 750 lb
2
T0 =
8 (0.19)
280 (168)
1 + [tan 26.5 + 4 (0.19) (1 2)] = 32, 000 lb
2
T1 =
8 (0.19)
Using the maximum tension of 50,000 lb and a factor of safety
of 3.5 gives a boom cable tension of 175,000 lb or 88 tons.
From Table 3, a 1-1/2-in.wire rope of galvanized improved
plow steel-fiber core (GIPS-FC) 6 19 construction with a break-
ing capacity of 82.80 tons would provide a factor of safety of 3.3.
The next largest wire rope diameter (1-5/8 in.) with a breaking
strength of 96.30 tons would increase the factor of safety to 3.9.
The length of the boom cable can be found from eq 7:
S0 = L 1 + s2 + tan2 θ
8
1
3
2
= (168) 1 + (0.19)2 + tan2 (26.5) = 205 ft
8
1
3
2
The final "eye-to-eye" cable length has to be determined after the
junction plates have been designed.



 Previous Page
Previous Page
