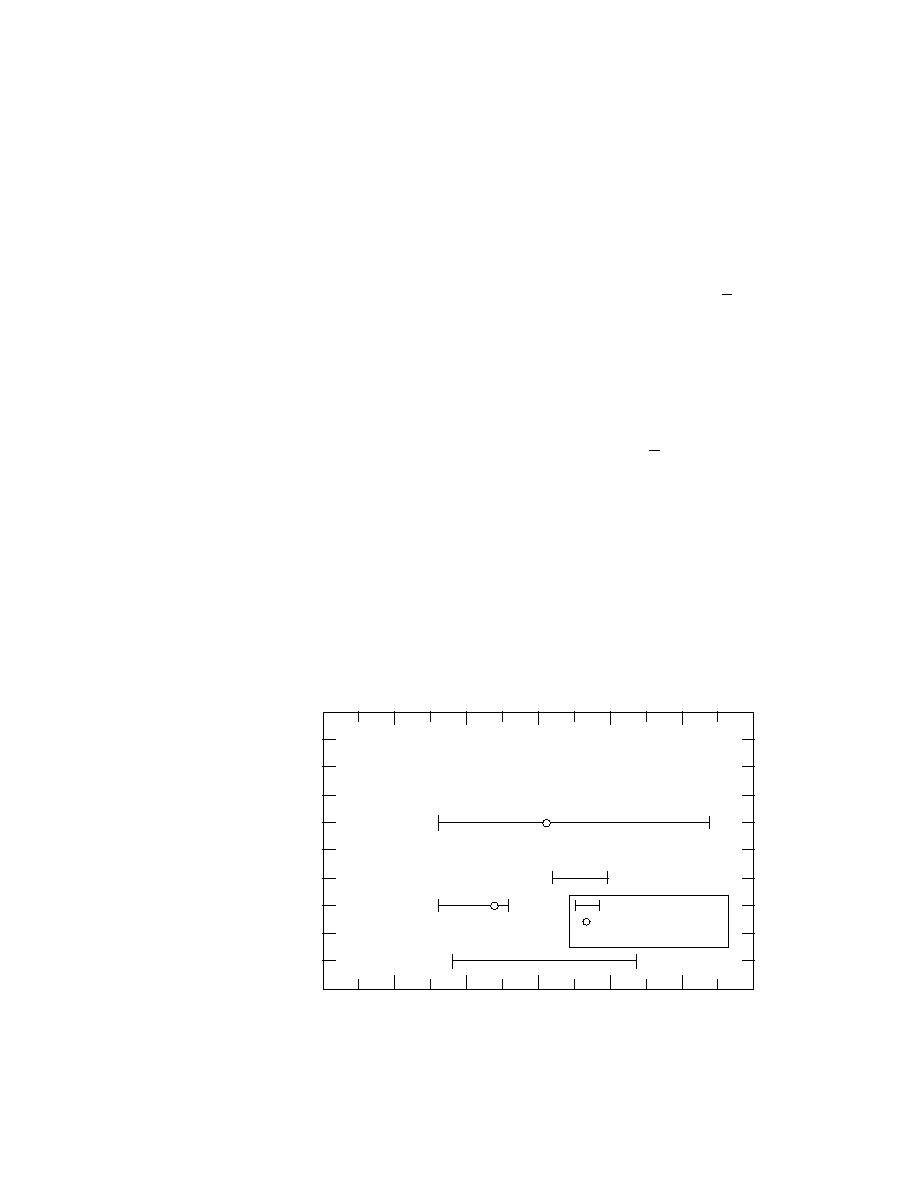
Andres (1980) calculated = 1.0 for the 1978
and bank; λ is the lateral stress coefficient, some-
breakup jam on the Athabasca River (Alberta).
Later, Andres and Doyle (1984) used Beltaos'
times called k1; and Kp is the passive pressure co-
(1978) approach to determine breakup jam char-
efficient (ratio of longitudinal to vertical stress at
acteristics for the Athabasca River breakup jams
the time of failure), labeled kx in some models. The
in 1977 and 1979 as well as 1978, reported previ-
lateral stress coefficient is often assumed to be 0.33
ously. Assuming Ci to be 96 Pa, they found to
(e.g., Tuthill and Mamone 1998). The coefficients
range from 0.8 to 2.7, with an average of 1.6. How-
k0 and Kp are calculated by
ever, they point out that is very sensitive to er-
rors in discharge, and that the unexpectedly wide
φ
k0 = tan(φ) and Kp = tan 2 45 +
range of is probably attributable to errors in es-
(23)
2
timating discharge. Tuthill and White (1997) re-
ported = 1.17 when modeling a breakup jam on
where φ is the angle of internal friction in degrees.
the Salmon River, Connecticut. Prowse (1986) cal-
φ is sometimes approximated using the angle of
culated = 1.06 for the 1983 ice jam at the
repose in dry materials, and often assumed to be
confluence of the Liard and Mackenzie Rivers,
45 in ice. Substituting, eq 22 becomes
Northwest Territories, while Rivard et al. (1984)
calculated higher values (2.0, 1.6) for two cross
φ
sections of the Mackenzie River jam that same year.
= tan(φ) tan 2 45 + λ(1 - p)
(24)
Korbaylo and Shumilak (1999) used a value of =
2
1.5 when modeling the 1986 Churchill River
breakup jam. Reported values of are summa-
in which the dependence of the friction coefficient
on angle of internal friction can be seen.
rized in Figure 9.
Michel (1980) calculated an average value of φ
If the lumped variable approach is not used,
= 21 based on data from 10 jams. Weiss et al. (1981)
the friction coefficient is calculated using the fol-
report 11 < f < 34 in a series of shear box tests on
lowing equation using theory developed for
ice rubble, with higher values for rubble formed
granular materials:
from thicker parent ice sheets. Slower displace-
= k0Kpλ(1 p)
ment for the same ice thickness also resulted in
(22)
Korbaylo and Shumilak (1999)
X
Tuthill and White (1997)
X
Beltaos et al. (1996)
X
Andres and Doyle (1984)
Prowse (1986)
X
Rivard et al. (1984)
= Range
Beltaos (1982)
= Mean Value
Andres (1980)
X
= Only Value Reported
X
Beltaos (1978)
0.5
1.0
1.5
2.0
2.5
0
Coefficient of Internal Strength,
Figure 9. Calculated values of the coefficient of internal strength ().
14



 Previous Page
Previous Page
