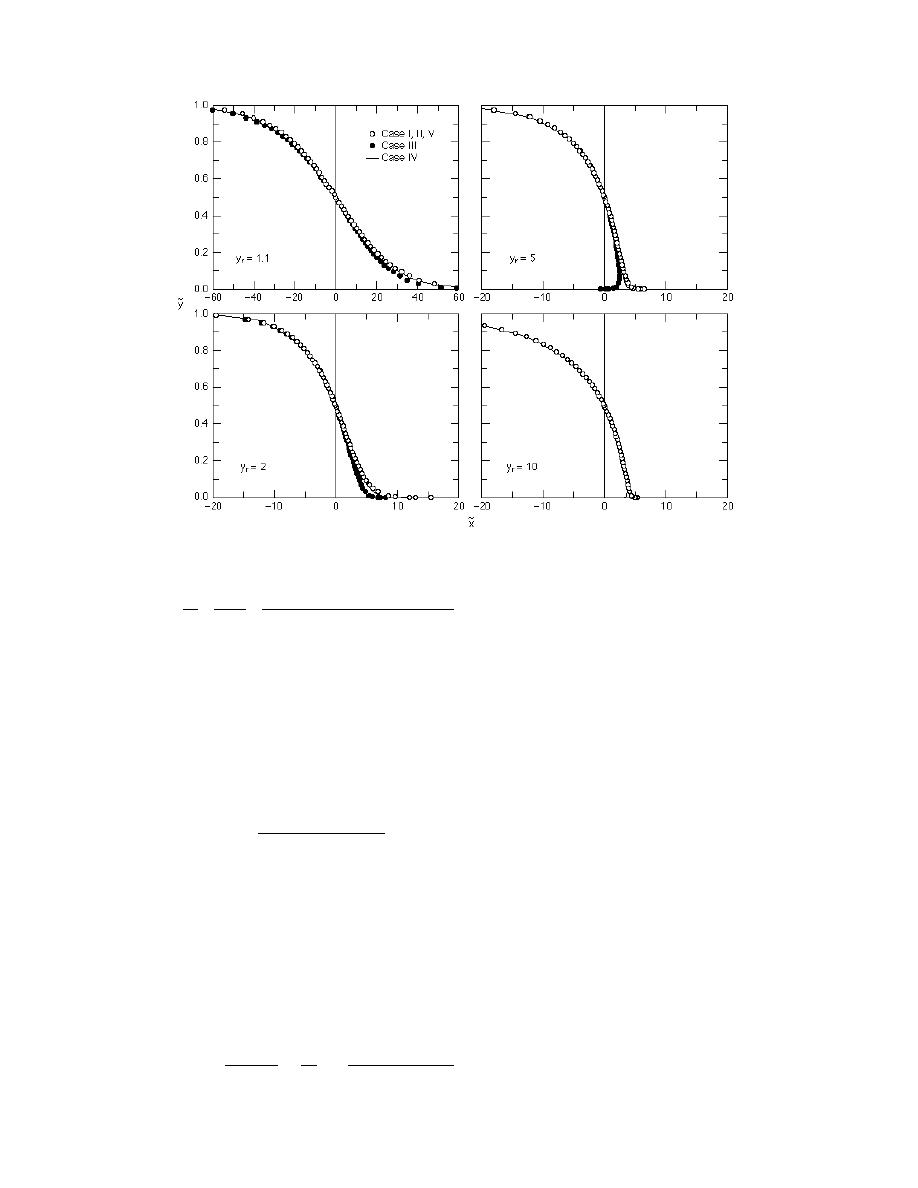
Figure 14. Monoclinal profiles for all cases at two dimensionless distance scales for depth
ratios ranging between 1.1 and 10.
(y/ y0 )(yr /2 - 1) - yr (yr/2 - 1)
3
1
q
vy
=
=
.
(42)
(yr - 1)
q0 v0 y0
Defining dimensionless unit discharge with the same form as dimensionless depth, eq 2
becomes
q=y
(43)
~~
independent of the depth ratio. Using the Chezy equation we obtain a corresponding rela-
tionship for dimensionless unit discharge in steady flow conditions as a function of depth
ratio
[(yr - 1)y + 1]3/ 2 - 1.
~
=
(44)
~
qsteady
-1
yr /2
3
In the linear wave limit as yr approaches 1, the rating curves represented by eq 43 and 44 are
identical. These rating curves, given in Figure 15, indicate that for a given dimensionless
depth as yr increases the monoclinal wave unit discharge also increases relative to that for
steady flow.
Froude number and energy gradient along a linear wave are unchanged from those of
the initial steady flow. Large amplitude monoclinal waves with discharges along the pro-
file that greatly exceed those of steady flow at comparable depths can have significantly
larger Sf and F. We define E ≡ Sf/S0, and with the Chezy equation and eq 26 obtain
[(y
]
)
2
-1 y+1
3/2
2
~
F
)2
(v/v0
r
=
E=
=
(45)
.
[(yr - 1)y + 1]
F0
3
y/ y0
~
20



 Previous Page
Previous Page
