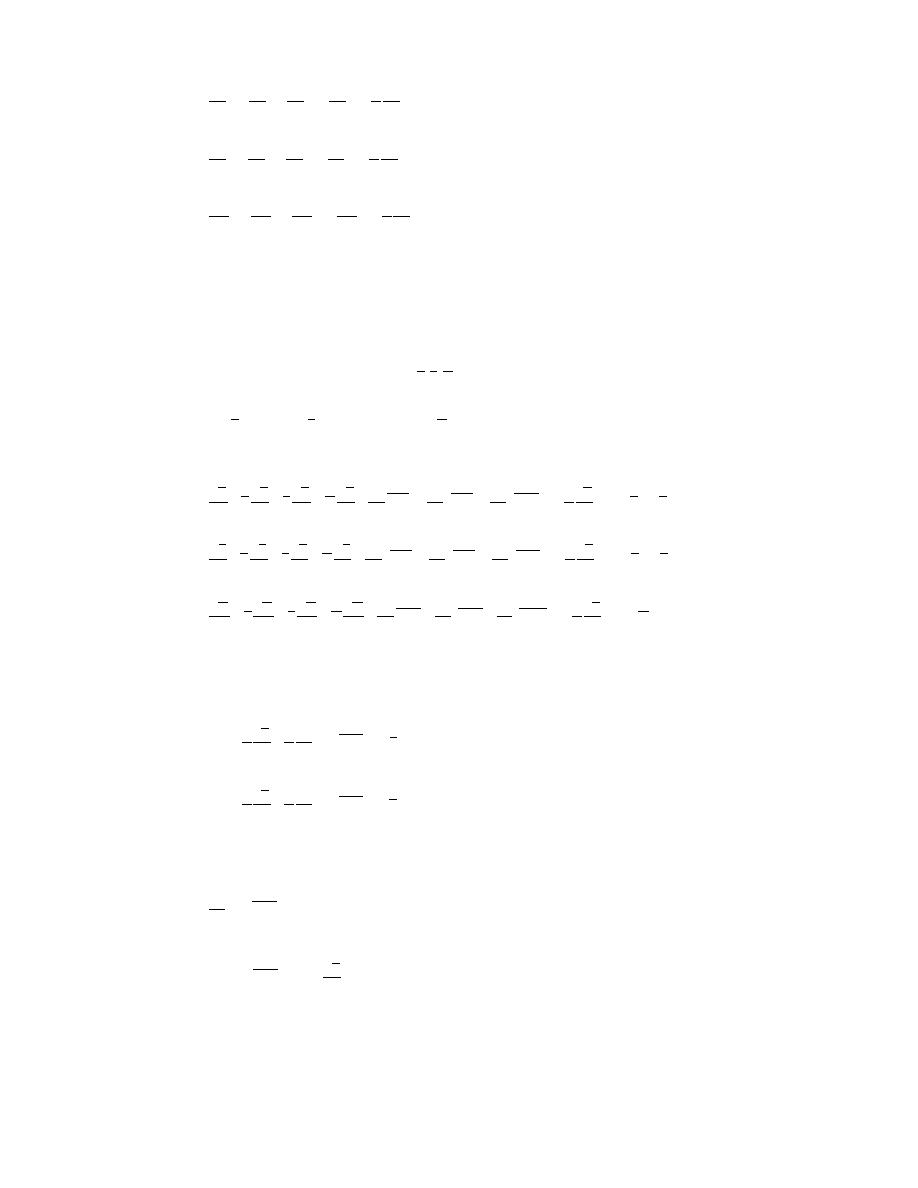
u
u
u
u
1 p
+u
+v
+w
=-
+ v∇2u + fv
ρ x
t
x
y
z
v
v
v
v
1 p
+u
+v
+w
=-
+ v∇2v - fu
(1)
ρ y
t
x
y
z
w
w
w
w
1 p
+u
+v
+w
=-
+ v∇2w - g
ρ z
t
x
y
z
where u, v, and w are the velocity components of the instantaneous wind vector, f is the Coriolis
parameter, x, y, and z represent the Cartesian coordinate system, p is the pressure, ρ is the air
Laplacian operator.
Equation 1 describes the instantaneous motion of the atmosphere. However, in turbulent flow it
is convenient to describe the mean rather than the instantaneous flow; this can be done by introduc-
ing components of the mean wind vector (u , v, w ) and the components of the turbulent wind vector,
i.e.,
u = u + u′,
v = v + v′,
w = w + w′
(2)
and
With the use of eq 2 and the application of the equation of continuity and averaging, eq 1 becomes
u
u
u
u
1 p
+u
+v
+w
+
u′u′ +
u′v′ +
u′w′ = -
+ v∇2u + f v
ρ x
t
x
y
z
x
y
z
v
v
v
v
1 p
+u
+v
+w
+
v′u′ +
v′v′ +
v′w′ = -
+ v∇2v - f u
(3)
ρ y
t
x
y
z
x
y
z
w
w
w
w
1 p
+u
+v
+w
+
w′u′ +
w′v′ +
w′w′ = -
+ v∇2w - g
ρ z
t
x
y
z
x
y
z
Considering that the vertical variation within the planetary boundary layer is usually much
greater than the horizontal variations and considering the case of steady state, eq 3 can be further
simplified as
(
)
1 p 1
0=-
+
-ρu′w′ + f v
ρ x ρ z
(4)
(
)
1 p 1
0=-
+
-ρv′w′ - f u
ρ y ρ z
However, to a good approximation, near the surface the pressure gradient and the Coriolis force can
be neglected and eq 4 can be written as
(-ρu′w′) = 0
(5)
z
or
u
τ = - ρu′w′ = ρKm
= Constant ,
(6)
z
in which Km is the turbulent diffusivity of momentum. Equation 6 states simply that the Reynolds
stress is a constant within the surface boundary layer and is independent of height. In a similar
4



 Previous Page
Previous Page
