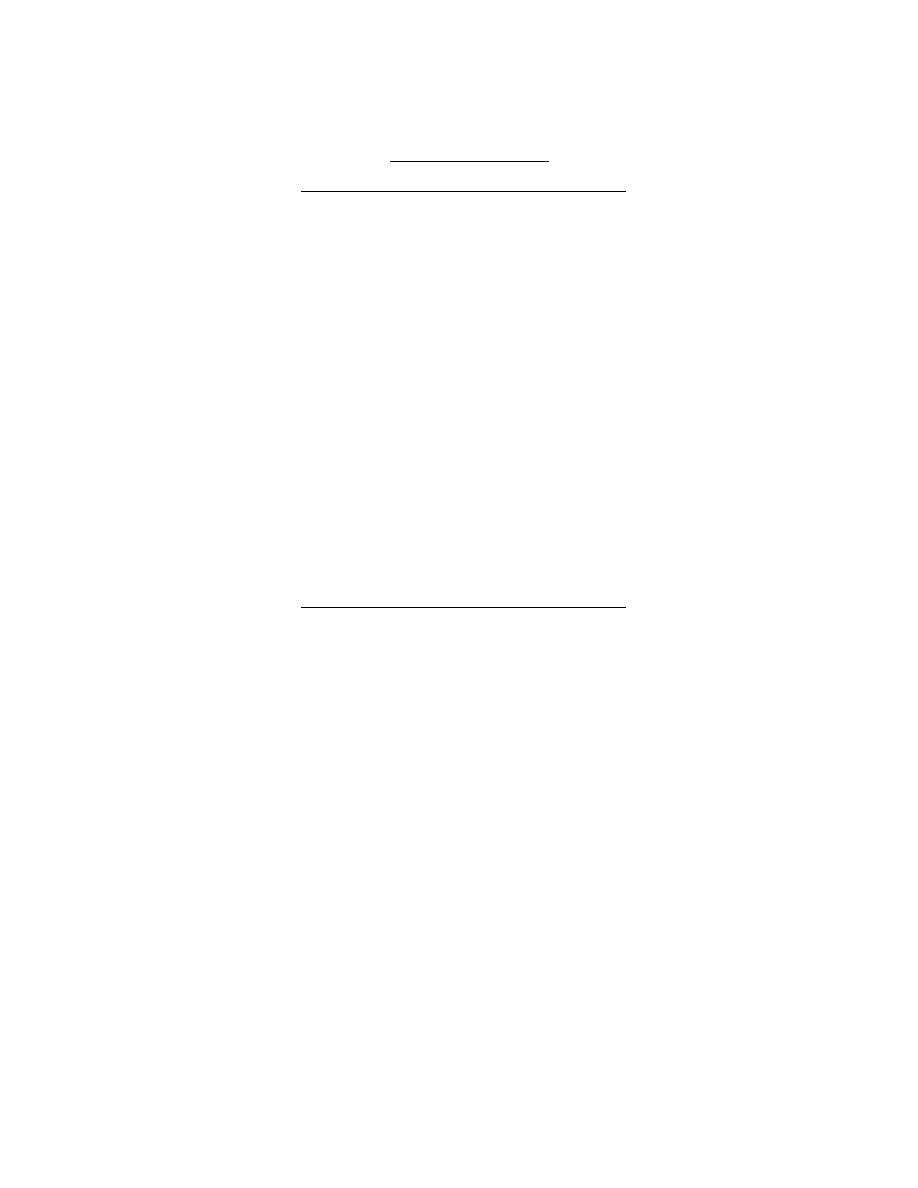
Table 12. Pipe size combinations for the example.
Pipe segment
Combination
number
(6,7)
(7,2)
(7,3)
1
0*
0
0
2
0
0
+
3
0
0
4
0
+
0
5
0
+
+
6
0
+
7
0
0
8
0
+
9
0
10
+
0
0
11
+
0
+
12
+
0
13
+
+
0
14
+
+
+
15
+
+
16
+
0
17
+
+
18
+
19
0
0
20
0
+
21
0
22
+
0
23
+
+
24
+
25
0
26
+
27
*0 = pipe size unchanged; + = pipe size increased; = pipe size
decreased.
increased by more than one discrete size. We will ignore this possibility for the
moment and return to it later, since it would result in many more combinations to
be checked, most of which would violate h1.
If we first look at all the possible combinations of increasing or decreasing the
three pipe sizes without regard to the constraints, we have 33 = 27 independent
possibilities; they are enumerated in Table 12. Combination number 1 is our design
as it now stands, the "do nothing" option. A number of these combinations are
known not to yield improvement in our design, however, and may be immediately
dismissed without further evaluation.
Specifically, any combination that increases any pipe sizes while decreasing none
will only result in additional pipe capital and heat loss costs and thus will be worse
than our design as is. Thus, the combinations 2, 4, 5, 10, 11, 13 and 14 can be
dismissed.
In addition, we know that any combination that increases the diameter of either
the final pipe servicing consumer 2 [(7,2)] or consumer 3 [(7,3)], while decreasing the
other and leaving pipe segment (6,7) unchanged, would be more costly than doing
the same yet not increasing the diameter of the one pipe; thus, we eliminate
combinations 6 and 8. As we proceed to explore the various combinations remain-
ing, we will discover that many other possible combinations will immediately be
shown to be infeasible by the infeasibility of related combinations.
In Table 13 we have listed the remaining combinations. Table 13 also gives the
68





 Previous Page
Previous Page
