σT = σn .
(14)
dφ
Yh dθ
du
= (Y - 1) w -
.
(11)
θo dz
dz
dz
Now if σT is assumed to be equal to the maxi-
mum heaving pressure, then the location where a
new ice lens forms is where σn also equals the
Criterion for
maximum heaving pressure. Snyder and Miller
lens initiation
(1985) found that they could correctly model their
To calculate when a new lens forms and the
empirically measured neutral stress data by
lensing cycle is complete, a criterion for lens ini-
tiation is required. Simply stated, a new lens will
σ n = χuw + (1 - χ)ui ,
(15)
form when ice can penetrate between the grains,
causing the grains to separate. Other factors must
in which the new soil function was determined to
be included if the porous material was not granu-
be
lar. For example, cements and rocks that have
chemical bonds between the grains must have the
1 W (φ) - Wd
bonds broken before the ice can penetrate.
χ (φ) =
2 Wsat - Wd
Geotechnical engineers express the state of
stress within a granular material by the Terzaghi
equation
()
W φ j - Wd
n
0.3
.
σT = σe + σn
∑
-
(12)
(16)
j =1 Wsat - Wd
φn
and the condition when the grains separate by
Algorithm
σe = 0 .
(13)
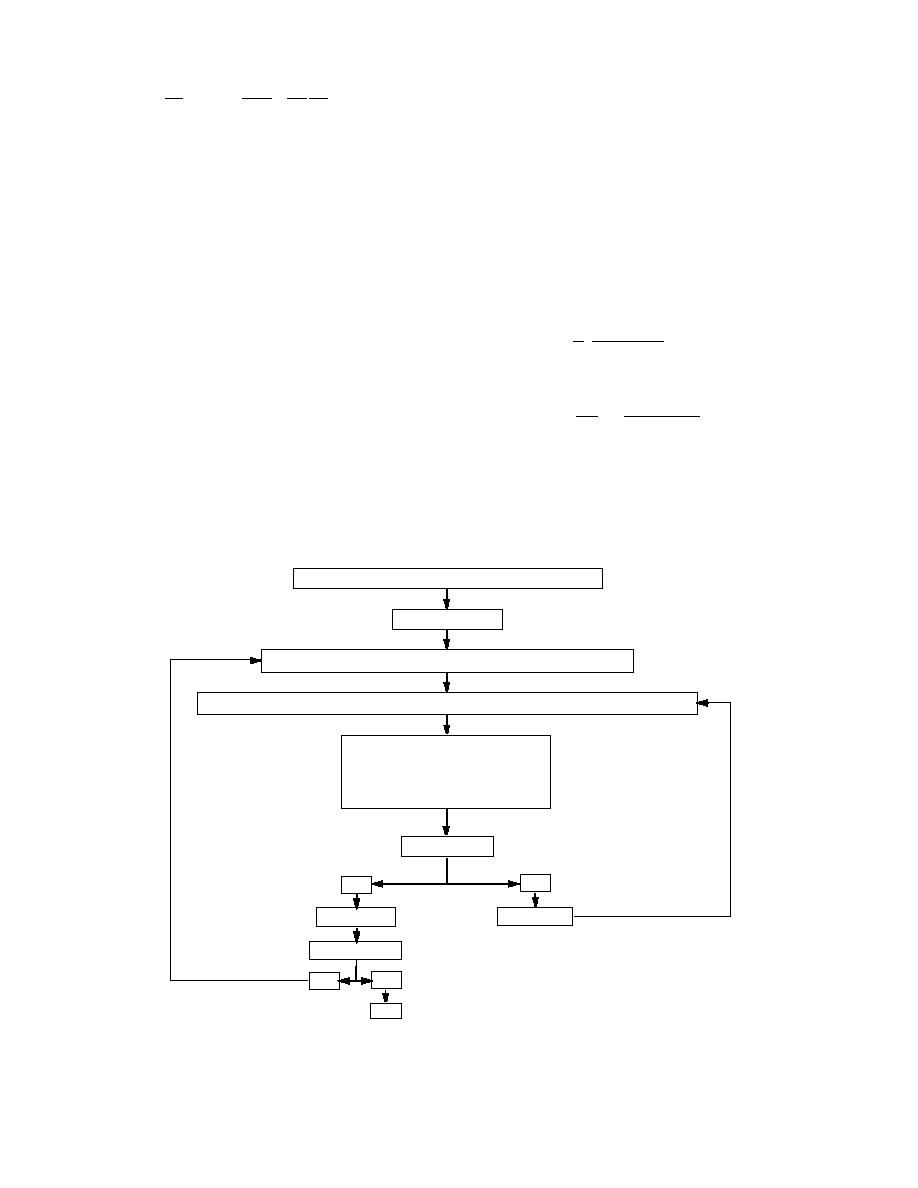
The solution strategy begins by stating values
for vi, vb and (dθ/dz)b and all necessary param-
At this instance
State: v i , v b , (d θ/dz)b , d φ and necessary soil properties
Assume: W f = Wd
Start with φ = φ b , assume u w = 0 and solve Clapeyron equation for θb
Calculate qw (φ) and qh (φ) from balance laws and W(φ), kw (φ), kh (φ) and χ(φ) from soil functions
Calculate:
θ with Fourier's law
u w with Darcy's law
u i and z with the Clapeyron equation
σn with Terzaghi's equation
Is u i = (σn ) max
No
Yes
Increment φ
Calculate W f
Within precision
Yes
No
Done
Figure 2. Flow chart of RIGIDICE.
4





 Previous Page
Previous Page
