4.1.4 Latent Heat
The latent heat flux term quantifies the energy lost or gained from the surface due to
evaporation or condensation, respectively. In order for latent heat to be present, there
must be a vapor gradient between the surface and the atmosphere. It is formulated as
(Kahle 1977, Balick et al. 1981b)
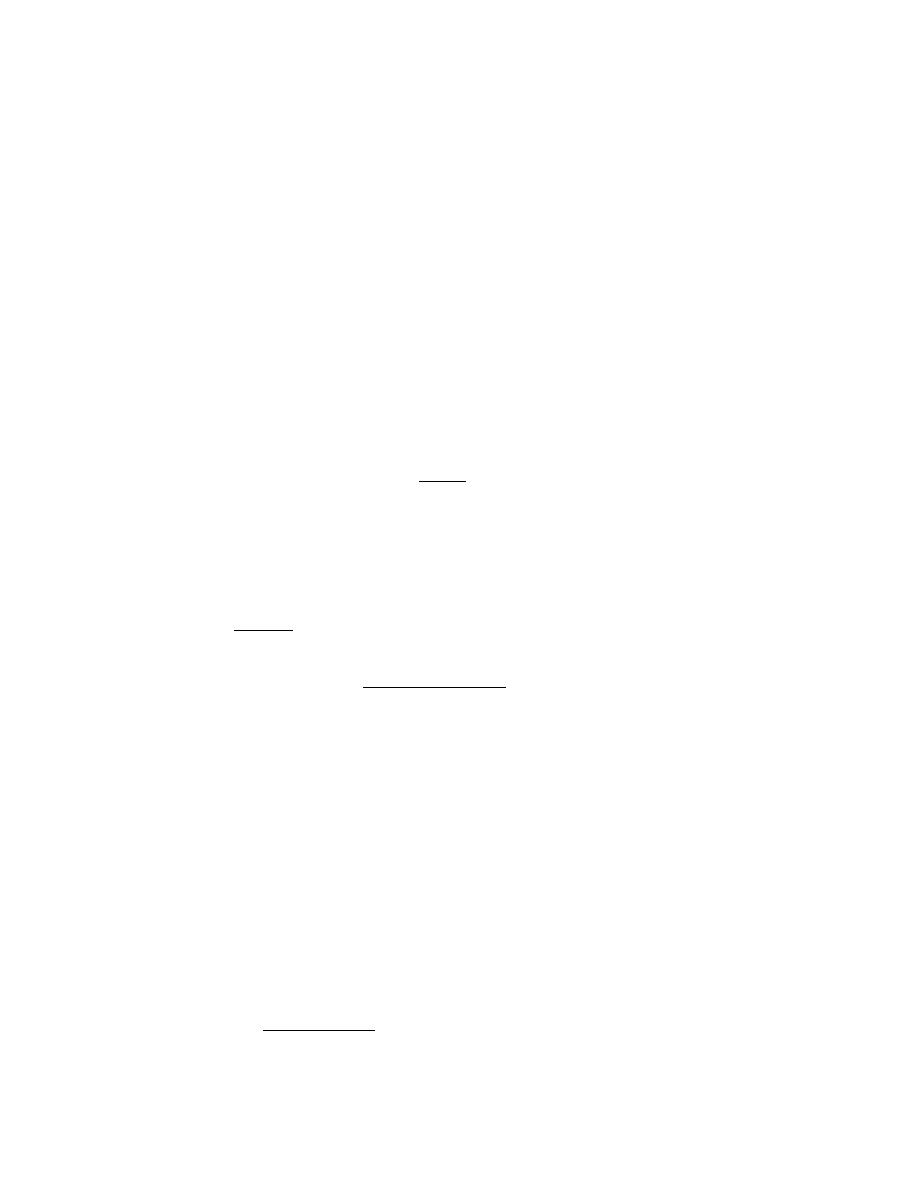
L = ρaCDW ' l (qg - qa )
e
(4.8)
J/kg), or sublimation, lsub (2.838e06 J/kg), depending on the air and surface temperatures,
qg is the mixing ratio of the air at the surface, and qa is the mixing ratio of the air at 2.0 m,
and W is the corrected windspeed (m/s). If W is below 2.0 m/s then W = 2.0 m/s else
e
h
W = W (Kahle 1977, Hughes et al. 1993). CD is described similarly to CD except that
the turbulent Schmidt number, rch, is replaced by the turbulent Prandtl number, rce (0.71),
calculating the bulk Richardson number in Equation (4.7). Balick et al. (1981) give the
latent heat of evaporation as
⎡T - T
⎤
levap = 2, 500, 775.6 - 2369.729 ⎢ a
- 273.15⎥ .
(4.9)
⎣ 2
⎦
The mixing ratio qg = M g qs (T ) with Mg the moisture factor ( 0 ≤ M g ≤ 1 ) and qs the
saturated mixing ratio. The value assigned to the moisture factor depends on the degree
of saturation of the soil. If it is raining, M g = 1 otherwise it is equal to the surface soil
moisture content (refer to Chapter 7). Balick et al. (1981) quantify the mixing ratios as
0.622ed
qs,a =
Pa - ed
(4.10)
⎡17.269(Ta - 273.15) ⎤
ed = RH s,a ⋅ 610.78 exp ⎢
⎥
Ta - 35.86
⎣
⎦
where ed is the vapor pressure (Pa) and RHs,a is the relative humidity. RHs = 1.0 and
RHa = RH and
⎧ 17.269
over water
a=⎨
⎩21.8745 over ice / snow
⎧35.86
over water
b=⎨
⎩ 7.66 over ice / snow
4.1.5 Precipitation Heat
Jordan (1991) quantifies the precipitation heat flux as
P = U p c pTp
(4.11)
fallrate(m / hr)
U p = -γ p
3600(sec/ hr)
32





 Previous Page
Previous Page
