ways less than 800 m, and diminished to less than 4 % of xdyn after 1 hour. The ratio of these
origin positions rapidly approached 1 from below in all cases. Larger diffusion coefficients
correspond to larger absolute differences in origin position early and generally larger ratio
differences from 1.
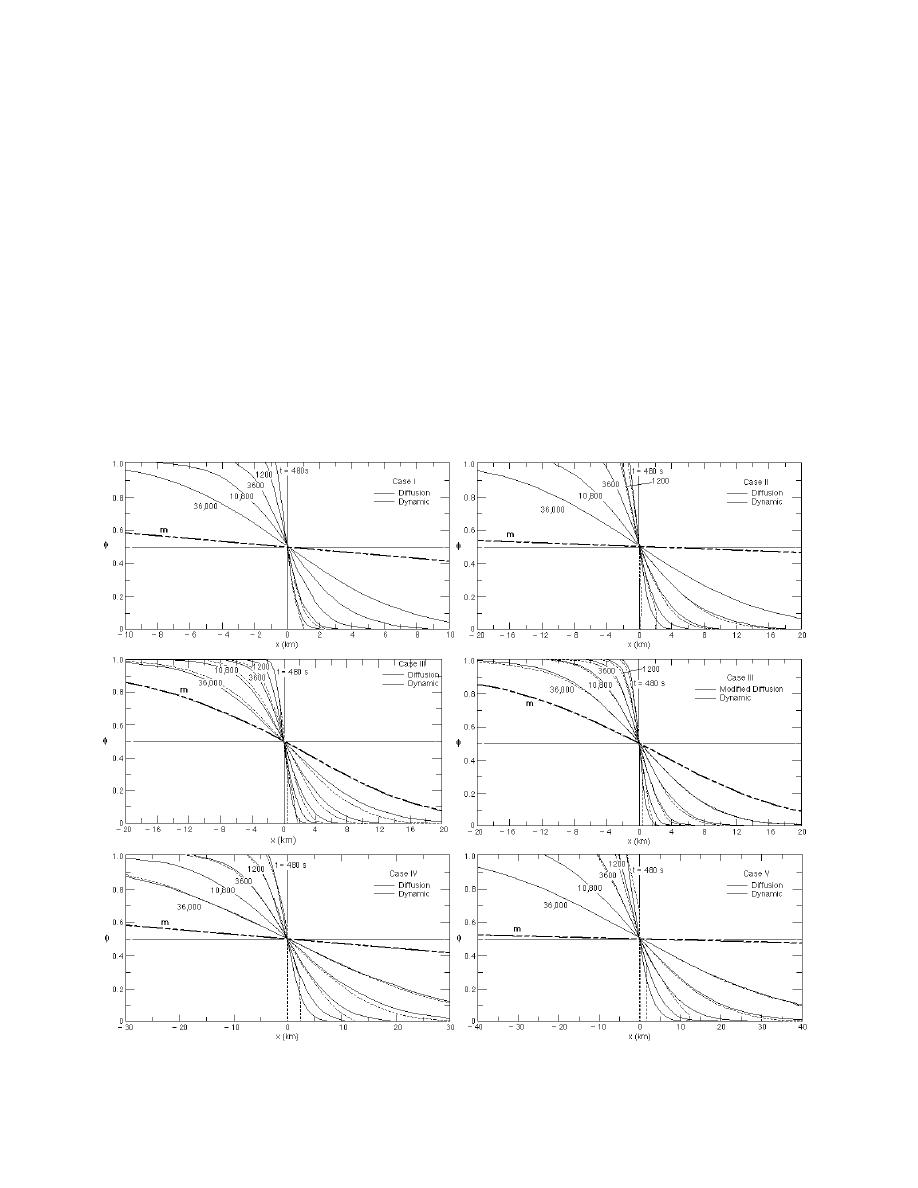
Dynamic wave and diffusion wave profiles at selected times are compared in the moving
coordinate system in Figure 5. The dynamic wave solution includes the initial shock on the
forerunner. At early time the leading edge of the diffusion profile always precedes that of the
dynamic profile by a distance that increases with D or η and the Froude number. The profiles
in case I rapidly converge and are nearly identical after 1200 s. In case II the shock front is
preserved for a longer time, and the profiles converge by 10,800 s. With high F0 in case III the
profiles tend to converge after the shock diminishes, but the dynamic wave profile retains
more steepness than the diffusion wave profile. Case III profiles with the inertial diffusion
coefficient are nearly identical after 3600 s. In case IV the shock persists for a longer time, and
profile convergence requires more than 10,800 s. The minor differences in profile steepness
remaining at large times can be minimized with the inertial diffusion coefficient. The case V
profile comparisons are similar to those of case IV, but with a low Froude number the inertial
diffusion coefficient is not needed for agreement at large times. Monoclinal wave profiles of
Figure 5. Linear diffusion wave and dynamic wave profiles and small-amplitude monoclinal wave profile m for all
cases plus case III with the modified inertial diffusion coefficient. Note the changes in the distance scale between panels.
13



 Previous Page
Previous Page
