SIMPLE FLUX MODEL

ICICLES

COLLISION EFFICIENCY

CR96_02
Page Navigation
13

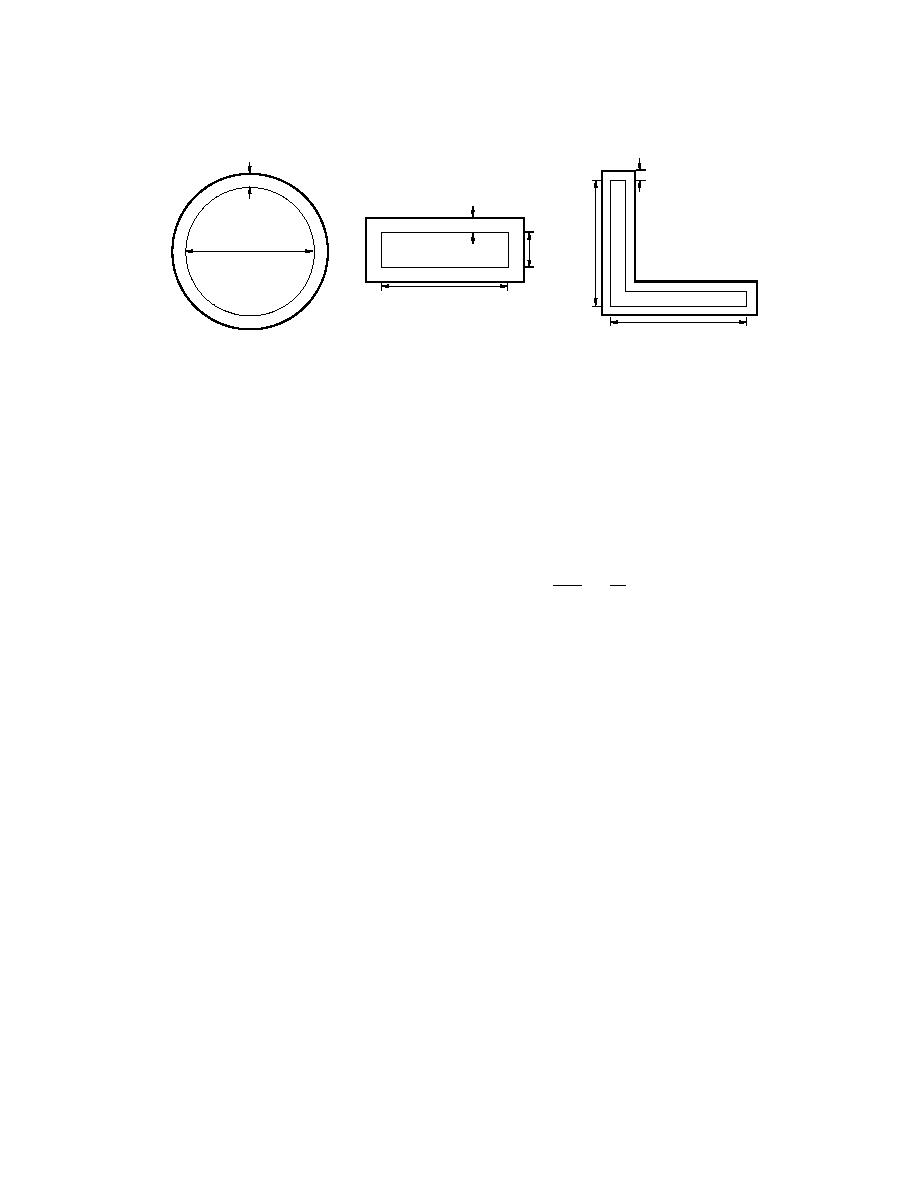
Figure 5. Equivalent uniform radial ice accretions on cylinders with circular, rectangular, and angle

cross sections for a rainfall of 1 cm. The width of the rectangle is 1/4 of its length. The angle has equal-

length legs with arbitrary thickness. The perimeter-to-"diameter" ratio S/D ranges from 3.14 for the

circle to 2.5 for the rectangle and 4 for the angle.

sumed average icicle length as both h and hm are

which intercepts the precipitation. This calcula-

proportional to Lbar1/4. As for the protoicicle, Qw

tion can be generalized to a cylinder with any cross

= 0 and Qs = 0 and the freezing fraction fs is

section to determine the uniform ice thickness on

determined using eq 21. The water that does not

its perimeter:

evaporate or freeze to the sides of the icicle forms

a protoicicle at its tip, and the heat balance for the

protoicicle is determined as before. Finally, the

(26)

Sρi j =1  10 

 

water that does not freeze at the tip is assumed to

drip off. The new total icicle mass Mi is distributed

evenly over a cylinder with length Li equal to the

where the summation gives the total precipitation

original icicle length plus the length of the

amount in N hours and S and D are the perimeter

protoicicle.

and horizontal dimension, respectively, of the cross

section. Equivalent uniform ice thicknesses are

shown in Figure 5 for three different cross sections

in a 1-cm rainfall.

6. SIMPLE FLUX MODEL

For many shapes, but not circles, the D/S ratio

The simplest case of ice accreting in freezing

decreases as ice accretes uniformly on the perim-

rain occurs if all the precipitation impinging on the

eter. For those, Req could be determined incremen-

structure freezes in a uniform radial accretion.

tally. When this is done for the rectangle in Figure

When that happens, the amount of ice on the

5, using 0.2-cm increments of rain, the final uni-

structure is directly related to the amount of rain

form radial ice thickness is 0.42 cm rather than 0.44

that falls. For example, if 1 cm of freezing rain falls

cm. This difference is well within the uncertainties

on a 2-cm-wide flat plate and freezes in place, then

in either this simple model or the detailed heat-

a layer of ice 1(ρo/ρi) = 1.1 cm thick results. On a 4-

balance model.

cm-wide plate nearby, the same thickness of ice

This simple precipitation model shows that, at

would form with twice the mass. A 2-cm-diameter

least to first order, the thicknesses of ice on compo-

horizontal circular cylinder intercepts the same

nents with the same shape cross-section but differ-

depth of rain as the 2-cm-wide flat plate. If that

ent diameters are the same. On components with

water depth is then spread uniformly around the

the same diameter but different cross sections, the

cylinder's circumference and frozen, forming a

ice thickness ratio is equal to the inverse of the

uniform radial accretion, then the layer of ice is

perimeter ratio. Thus, uniform radial ice thick-

1(ρo/ρi)/π = 0.35 cm thick. The factor of π is the

nesses determined by this model, or any other

ratio between the circular cylinder's circumfer-

model, for a circular cylinder of any diameter can

ence, over which the ice freezes, and the diameter,

be extrapolated both to cylinders with other cross

Index

Privacy Statement
Press Release
Contact