where ρ and η are the density and viscosity of the liquid (kgm3 and Pas, respectively), g is the gravitational constant
(ms2), and k is the intrinsic permeability of the porous matrix (m2) (Muskat and Meres 1936). Equations (2) and (3) indicate
that hydraulic conductivity will increase exponentially with temperature.
Hydraulic head at constant degree of saturation is a linearly decreasing function of temperature, the slope of which is
specific for each soil. The behavior in many soils has been fitted to the following equation:
β0 + T
h = h(Tr )
(4)
β0 + Tr
where h is hydraulic head (m), h(Tr), hydraulic head (m) at Tr, a reference temperature (in degrees kelvin, K = C + 273.15),
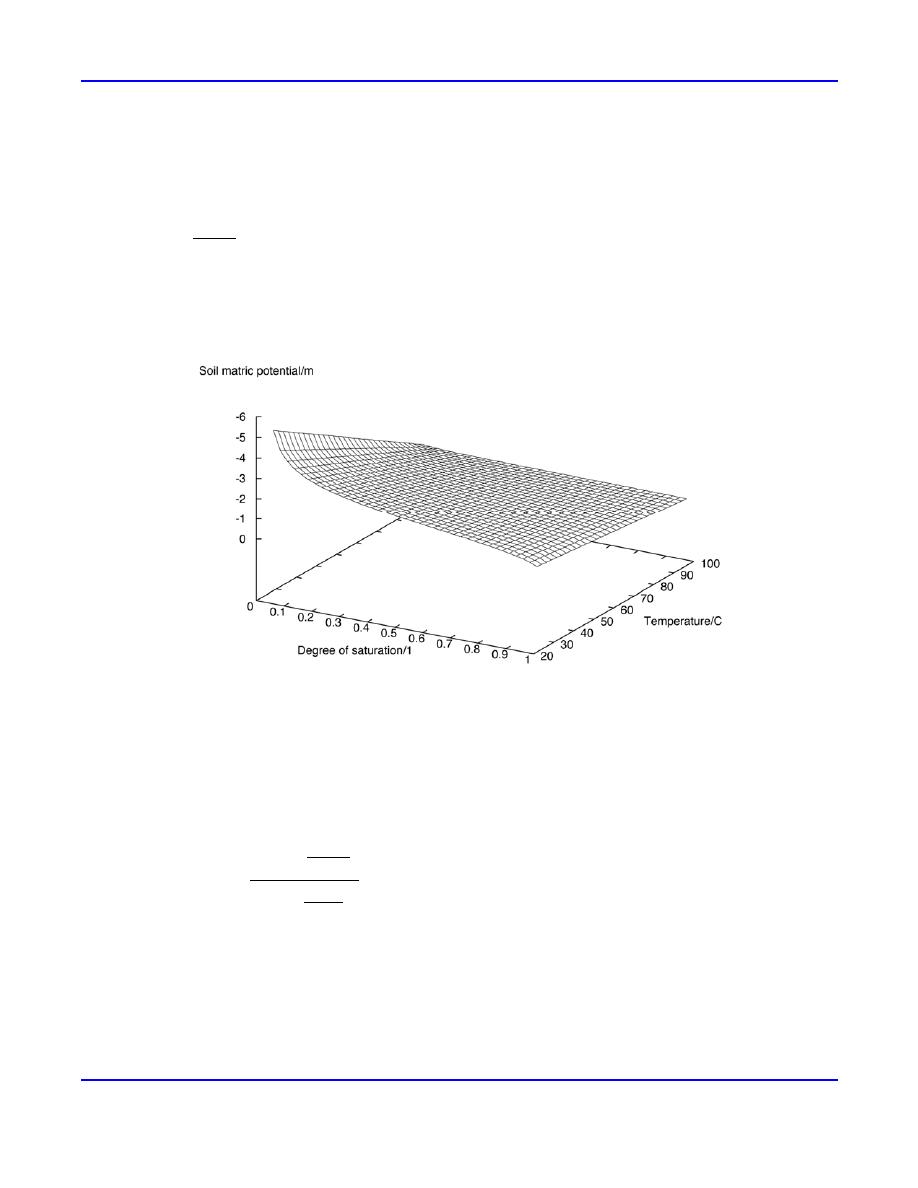
and 0, a fitted parameter (K) (Grant and Salehzadeh 1996). Figure 3 presents soil hydraulic head as a function of water
content and the 0 parameter.
Figure 3. Soil hydraulic head as function of degree of saturation and temperature.
Depending on soil, the 0 has values from 340 to 460 K. For infiltration or imbibition 0 is a simple function of the
geometric mean soil particle radius (Grant and Or in prep). This relationship is presented in Figure 4. In principle, therefore,
the 0 value of a soil can be estimated if its texture is available in a GIS database.
Cumulative infiltration can therefore be described by
1/ 2
⎡
⎛ β0 + T ⎞ ⎤
⎢ -hi (Tr )t ⎜
⎟⎥
β0 + Tr ⎠ ⎥
⎝
1/ 2 ⎢
I = [2k ρ g (θ0 - θi )]
(5)
⎢
⎛ B ⎞ ⎥
⎢ η0 exp ⎜
⎟ ⎥
T + T0 ⎠ ⎥
⎢
⎝
⎣
⎦
(Grant and Young in prep). Figure 5 presents cumulative infiltration after 180 s divided by cumulative infiltration at 25 C by
a soil with a 0 parameter value equal to 760 K. Figure 4 shows that temperature has an effect on infiltration and that these
effects are soil specific. For soils with 0 parameter values above 400 K, cumulative infiltration was an increasing function
of temperature. Coarse textured soils were likely to have 0 parameter values equal to or more negative than 400 K. For soils
with 0 parameter values closer to 340 K, which are likely to be finer textured soils, the estimated cumulative infiltration
was an increasing function of temperature to roughly 25 C, but a decreasing function of temperature from 25 to 50 C.
4
ERDC/CRREL TN-04-6





 Previous Page
Previous Page
