9
DESIGN OF ICE BOOMS
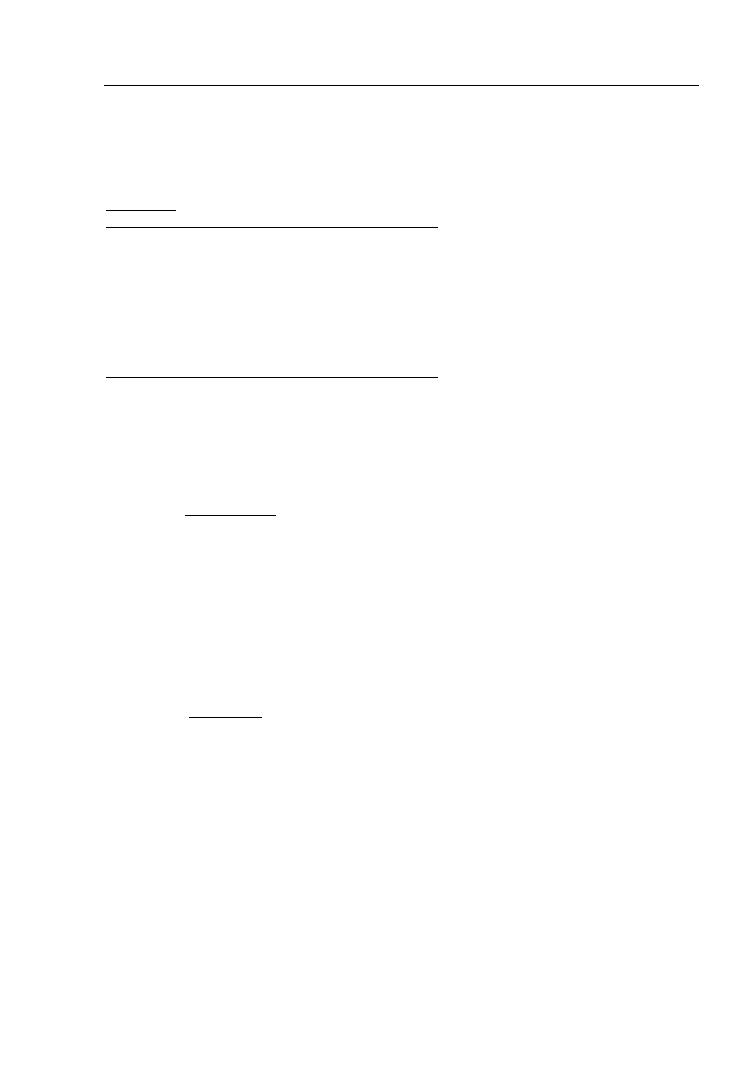
Table 2. Manning coefficients of roughness of the
bottom surface of initial ice covers (after Nezhikovsky
1964).
Initial
Cover
Cover
thickness
formed from
formed from
Brash ice
(ft)
(m)
loose slush
frozen slush
cover
0.3
0.1
--
--
0.015
1.0
0.3
0.01
0.013
0.04
1.6
0.5
0.01
0.02
0.05
2.3
0.7
0.02
0.03
0.06
3.3
1.0
0.03
0.04
0.07
4.9
1.5
0.04
0.06
0.08
6.6
2.0
0.04
0.07
0.09
10
3.0
0.05
0.08
0.10
16
5.0
0.06
0.09
--
Using SI units, the 2.22 constant disappears. The composite rough-
ness nc can be calculated from the Belokon-Sabaneev formula
(U.S. Army Corps of Engineers 1982):
2/3
ni3 / 2 + nb3 / 2
nc =
2
ni can vary from 0.01 to 0.10, depending on many factors, includ-
ing ice cover type, thickness, piece size, temperature and age.
Table 2 gives ranges of values for different types of ice covers.
Under conditions where an ice cover will form behind a boom, ice
roughness will typically be in the 0.020.04 range.
The hydraulic radius Rice of the ice-covered channel can be
found from
A
Rice =
Pbed + Pice
where A = the under-ice flow area
Pice = the wetted perimeter of the underside of the ice cover
Pbed = the wetted perimeter of the channel bed.
For wide rectangular channels, the hydraulic radius can be ap-
proximated as one-half the under-ice depth.
If channel geometry data are available, standard step backwa-
ter models, such as HEC-2 with the ice cover option (U.S. Army
Corps of Engineers 1990), are extremely useful in determining
the hydraulic parameters used in the water shear calculation, since
HEC-2 output variables include water/ice surface slope, water
velocity, depth and channel top width.



 Previous Page
Previous Page
