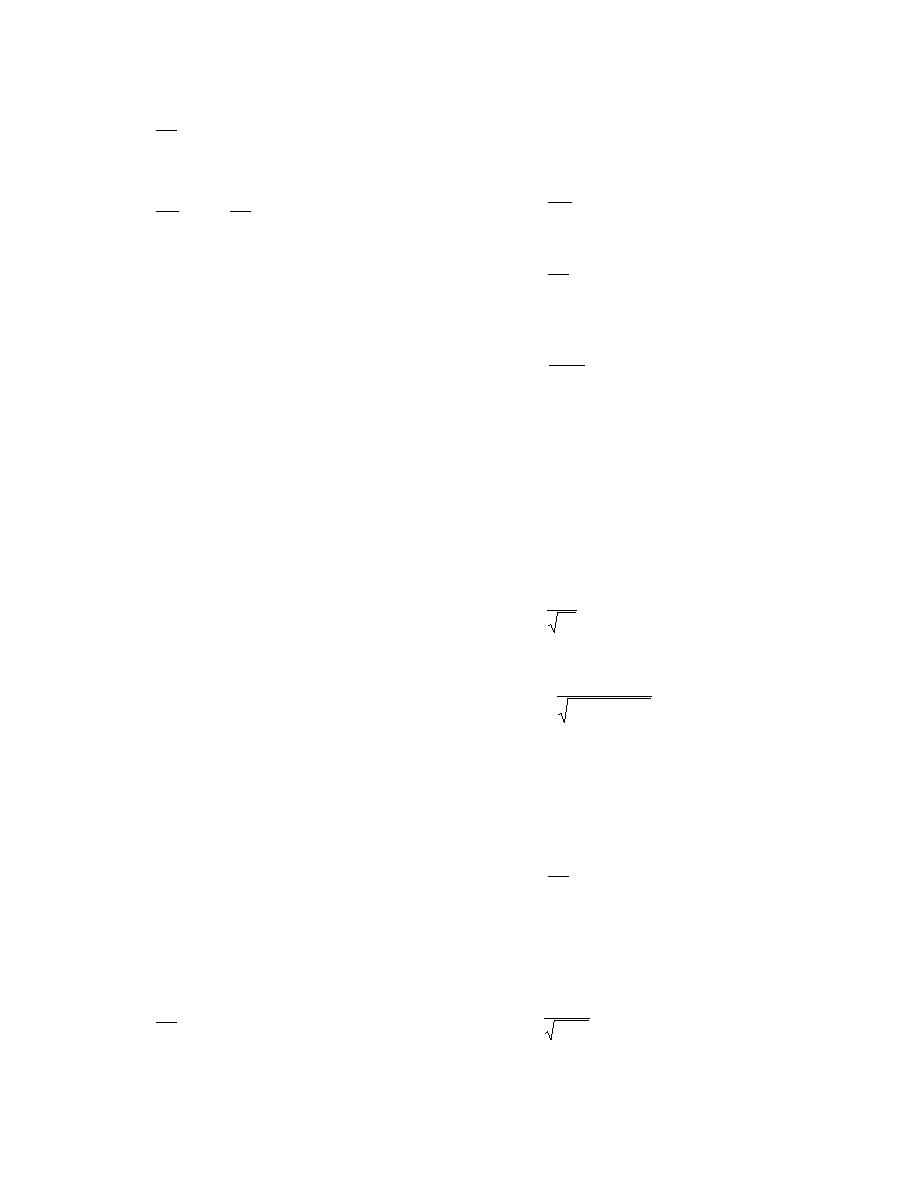
λ m = λρλ3
(5)
Tp
L
= λT
(2)
Tm
scale is
and
λF
λ
λa =
Vp
,
(6)
= λV = L .
λm
(3)
λT
Vm
or from velocity and time,
Ice modeling usually requires consideration of
λL
λa =
(7)
.
water movement, ice movement, and ice deforma-
λ2
T
tion and failure. The present discussion begins
with a brief review of the criteria for dynamic si-
The force scale can then be expressed as
militude of water flow and ice-piece transport,
λ4 λρ
then reviews the similitude criteria for ice-sheet
L
λF =
(8)
.
deformation and failure and the criteria for the
λ2
T
deformation and failure of ice rubble. The criteria
for ice-sheet, or rubble, deformation are less well
All dynamic quantities can be expressed in terms
developed or generally accepted than are the cri-
of the length, time, and density scales.
teria for water flow and ice-piece transport.
Most hydraulic processes are governed by
Modeling complexity and the constraints on
momentum balances involving inertial, gravita-
model ice selection increase markedly with mod-
tional, and viscous forces. Surface tension forces
eling situations requiring that both hydraulics and
usually are insignificant, except when considering
ice deformation criteria be met. Some modeling
very shallow flows or the movement of small ice
situations require consideration only of the simili-
pieces. The relative influences of inertial and grav-
tude criteria associated with water flow and ice-
itational forces can be expressed nondimensional-
piece transport. For those situations, an unbreak-
ly as a Froude number,
able model ice of appropriate buoyancy suffices.
V
Other modeling situations involve ice deforma-
Fr =
(9)
tion in static water and require satisfaction of the
gy
criteria for ice deformations and failure as well as
for ice-piece movement. Those situations require a
or as a densimetric Froude number,
model ice that deforms and breaks appropriately.
V
Modeling becomes complicated when the mod-
FrD =
(10)
gy(ρs - ρ)/ ρ
eled situation requires simulation of water flow as
well as the failure and transport of ice. When ther-
modynamic processes are important, thermody-
in which V is a representative velocity, y is flow
depth or an alternate length of interest, and ρs and
namic similitude criteria guide the modeling, and
ρ are solid and fluid densities, respectively. The
the skill of the modeler becomes vital. Generally,
relative influences of inertial and viscous forces
the more criteria to be met, the less accurate is the
can be expressed nondimensionally as a Reynolds
modeling, and greater reliance must be placed on
number,
the experience and interpretive abilities of the
modeler.
Vy
For more detailed coverage and discussion of
Re =
(11)
ν
the similitude criteria see Ashton (1986), ITTC
(1987, 1990, 1993), and Ettema et al. (1992).
in which ν is the kinematic viscosity of the fluid
(usually water). The relative magnitudes of inertial
Water flow and ice-piece transport
and surface-tension forces can be expressed
Dynamic similarity relates prototype and model
nondimensionally as a Weber number,
forces by a force scale; i.e.,
(12)
Fp
V
W=
(4)
= λF .
k /ρy
Fm
As the scale for mass is
in which k is the surface-tension strength of water.
3



 Previous Page
Previous Page
